Question

Maximise the function ${\displaystyle Z=11x+7y}$, subject to the constraints ${\displaystyle x\le 3,y\le 2,x\ge 0,y\ge 0}$

Answer

N/a

Explanation

Maximise Z=11x+7y, subject to the constrains `x le 3, yle 2, x ge 0, y ge 0`

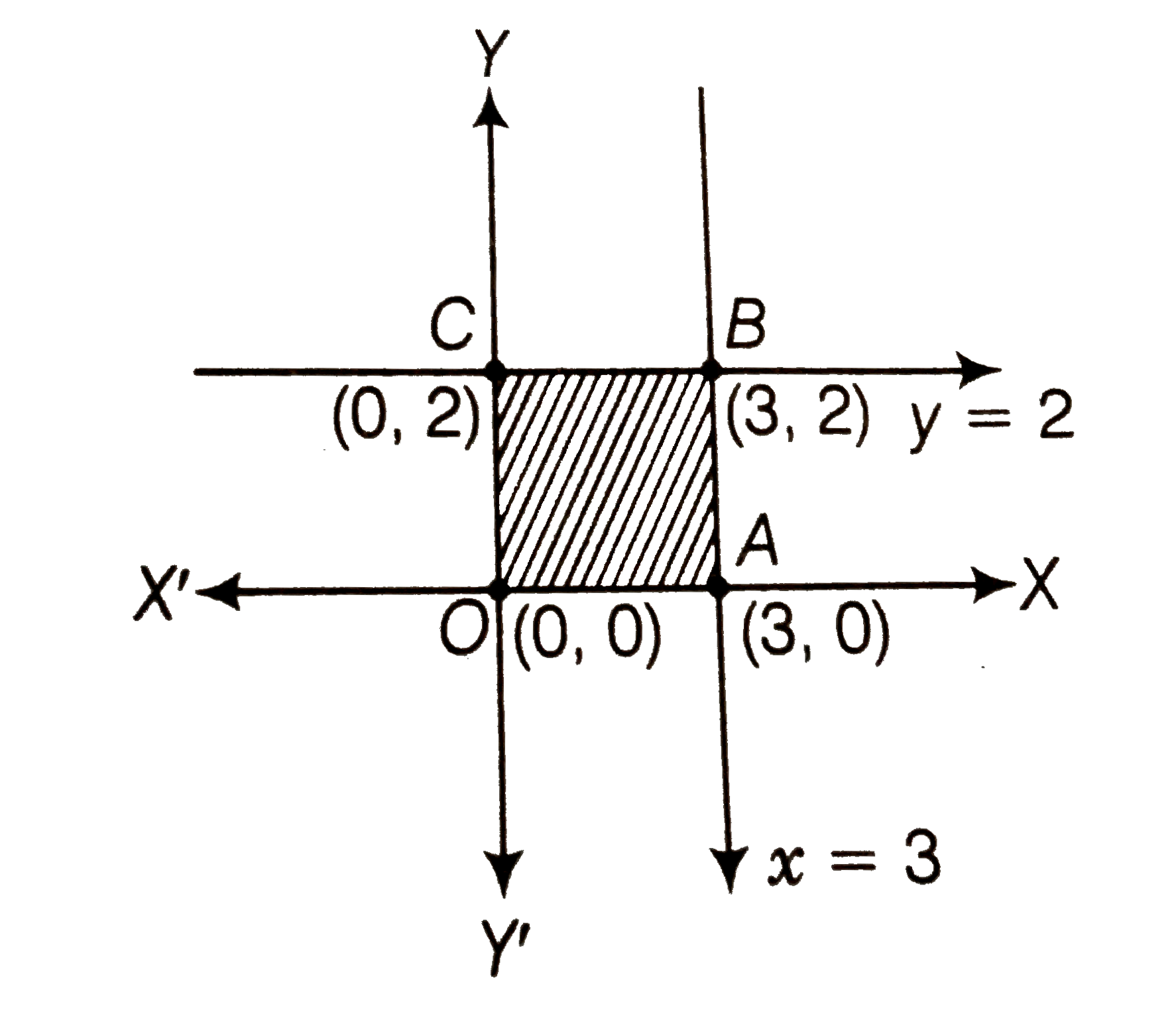
The shaded region as shown in the figure as OABC is bounded and the corrdinates of the corner points are (0,0),(3,0),(3,2), and (0,2) respectively

Hence, Z is maximum at (3,2) and its maximum value is 47