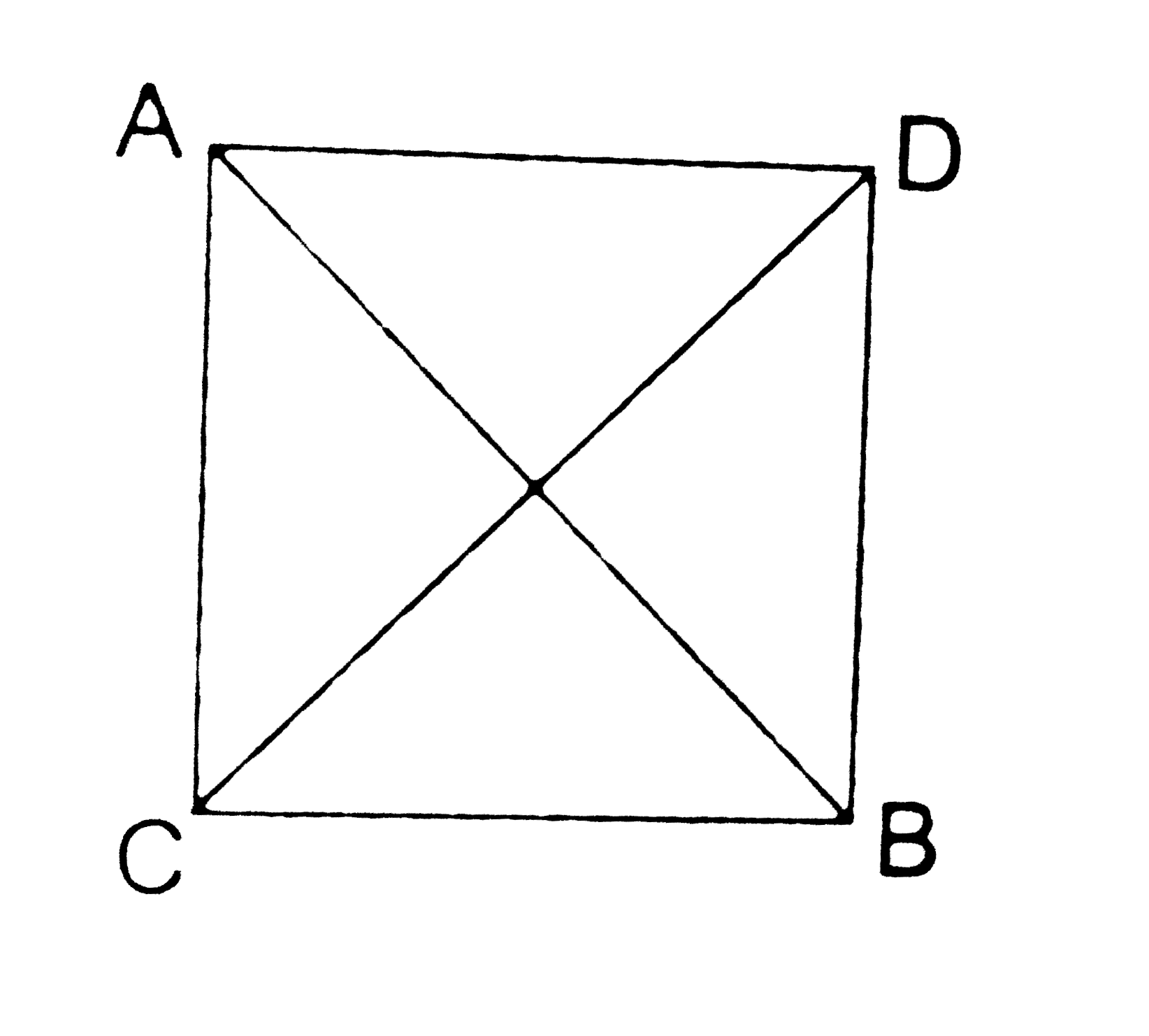
On a common hypotenuse AB, two right angled triangles, ACB and ADB are situated on opposite sides. Prove that ∠BAC=∠BDC.
Clearly, ∠ACB=90∘ and ∠ADB=90∘
Thus, the opposite angles of quad. ACBD are supplementary .
∴ ACBD is a cyclic quadrilateral.
This means that a circle passes through the points A,C,B and D
∴∠BAC=∠BDC[∠s in the same segment].
Similar Questions
Knowledge Check
- Question 1 - Select One
A point D is taken from the side BC of a right angled triangle ABC, where AB is hypotenuse. Then,
AAB2+CD2=BC2+AD2BCD2+BD2=2AD2CAB2+AC2=2AD2DAB2+AD2=BD2
Show that in a right angled triangle, the hypotenuse is the longest side.
View SolutionProve that the area of the equilateral triangle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the equilateral triangles drawn on the other two sides of the triangle.
View SolutionA side of an isosceles right angled triangle is x. Find its hypotenuse.
View SolutionA right angled triangle has legs 1 and x. The hypotenuse is y and angle opposite to the side x is θ. Shown as
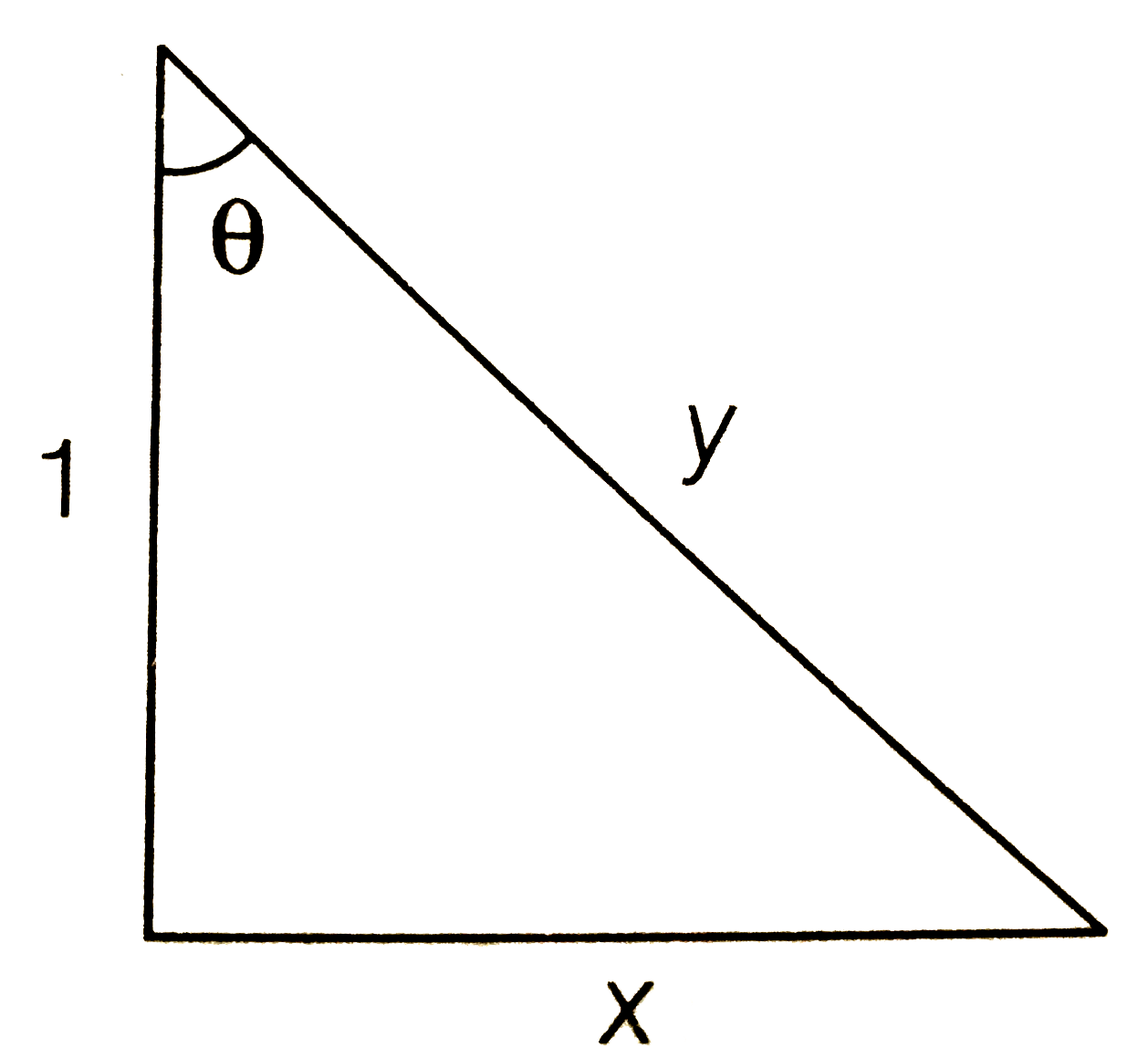
 View Solution
View SolutionFind the length of the hypotenuse in a right angled triangle where the sum of the squares of the sides making right angle is 169.
View SolutionIn a right angled triangle with sides a and b and hypotenuse c , the altitude drawn on the hypotenuse is x . Prove that ab=cx .
View SolutionProve that the area of the semicircle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the semicircles drawn on the other two sides of the triangle
View Solution

