Out of 100 students, two sections of 40 and 60 are formed. If you and your friend are among the 100 students, what is the probability that(a) you both enter the same section?(b) you both enter the different sections?
To solve the problem, we will calculate the probabilities step by step.
Step 1: Calculate the total number of ways to select 2 students from 100.
The total number of ways to select 2 students from 100 is given by the combination formula:
Total ways=(1002)=100×992=4950
Step 2: Calculate the number of ways for both to enter the same section.
There are two sections: one with 40 students and another with 60 students. We will calculate the number of ways both you and your friend can be in the same section.
1. Both in the section of 40 students:
Ways=(402)=40×392=780
2. Both in the section of 60 students:
Ways=(602)=60×592=1770
Now, add the two results together to find the total number of ways both can be in the same section:
Total ways in the same section=780+1770=2550
Step 3: Calculate the probability that both enter the same section.
The probability that both you and your friend enter the same section is given by the ratio of the number of favorable outcomes to the total outcomes:
P(same section)=Number of ways in the same sectionTotal ways=25504950
Now simplify the fraction:
P(same section)=2550÷25504950÷2550=11.941176≈1733
Step 4: Calculate the probability that both enter different sections.
To find the probability that both you and your friend enter different sections, we can use the complement rule:
P(different sections)=1−P(same section)=1−1733
Now calculate:
P(different sections)=33−1733=1633
Final Answers:
(a) The probability that you both enter the same section is 1733.
(b) The probability that you both enter different sections is 1633.
---
Ways=(402)=40×392=780
Ways=(602)=60×592=1770
Total ways in the same section=780+1770=2550
P(same section)=Number of ways in the same sectionTotal ways=25504950
P(same section)=2550÷25504950÷2550=11.941176≈1733
P(different sections)=1−P(same section)=1−1733
P(different sections)=33−1733=1633
(a) The probability that you both enter the same section is 1733.
Topper's Solved these Questions
- PROBABILITYBOOK - NCERTCHAPTER - PROBABILITYEXERCISE - EXERCISE 16.1
16 Videos
- PROBABILITYBOOK - NCERTCHAPTER - PROBABILITYEXERCISE - EXERCISE 16.3
21 Videos
- PROBABILITYBOOK - NCERTCHAPTER - PROBABILITYEXERCISE - SOLVED EXAMPLES
18 Videos
- PRINCIPLE OF MATHEMATICAL INDUCTIONBOOK - NCERTCHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTIONEXERCISE - EXERCISE 4.1
24 Videos
- RELATIONS AND FUNCTIONSBOOK - NCERTCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - EXERCISE 2.3
5 Videos
Similar Questions
Out of 100 students two sections of 40 and 60 students are formed. If you and your friends are among the 100 students, what is the probability that i. You both enter the same section? ii. You both enter the different section?
View SolutionOut of 100 students two sections of 40 and 60 are formed If you and your friend are among 100 students what is the probability (i) you both enter the same section (ii)you both enter the different sections
View Solution
Knowledge Check
Let there are 4 sections of 25 students each in a coaching class. Now, out of 150 students 100 are to be selected randomly and entrolled in these sections. Then, the probability that the students A and B (both present in 150 students) are selected and placed in the same section, is
A1013B16149C233D5149Out of two sections A and B, 10 students of section B shift to A, as a result strength of A becomes 3 times the strength of B. But, if 10 students shift over from A to B, both A and B become equal in strength. Ratio of the number of students in section A that of section B is
A2:1B5:3C3:1D9:4
In how many ways two sections of 30 students each can be formed from 60 students ?
View SolutionThe following table gives the distribution of students of two sections according to the marks obtained by them
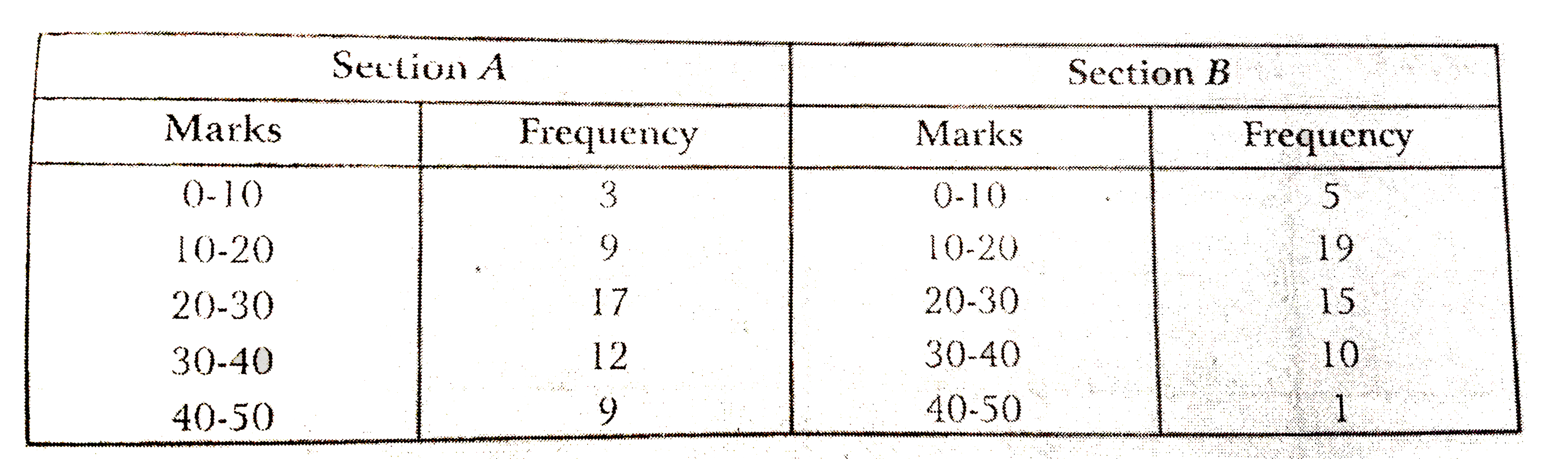
Represent the marks of the students of both the sections on the same graph by two frquency polygons. From the two polygons compare the performance of the two sections.
View SolutionIf the average marks of 30 students of section A is 55 and the average marks of 30 students of section B is 60 ,then find the combined average of both the sections.
View SolutionIf the average marks of 30 students of section A is 55 and the average marks of 30 students of section B is 60 ,then find the combined average of both the sections.
View SolutionYou have gone to a cyber-cafe with a friend. You found that the cyber-café has only three terminals. All terminals are unoccupied. You and your friend have to make a random choice of selecting a terminal. What is the probability that both of you will NOT select the same terminal is
View SolutionIn a school, there are 1,000 students, out of which 380 are girls. Out of 380 girls, 10% of the girls scored highest in GS. What is the probability that a student chosen randomly scored highest in GS given that the chosen student is a girl?What value do you observe in it?
View Solution
