P is a veriable poin on the hyperbola x2−y2=16 and A (8,0) is a fixed point . Show that the locus of the middle point of the line segment ¯¯¯¯¯¯AP is another rectangular hyperbola. Solution in Bengali
The correct Answer is:3y2−x2=12
Step by step video, text & image solution for P is a veriable poin on the hyperbola x^(2) - y^(2) =16 and A (8,0) is a fixed point . Show that the locus of the middle point of the line segment bar(AP) is another rectangular hyperbola. by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- HYPERBOLABOOK - CHHAYA PUBLICATIONCHAPTER - HYPERBOLAEXERCISE - LONG ANSWER TYPE
10 Videos
- HYPERBOLABOOK - CHHAYA PUBLICATIONCHAPTER - HYPERBOLAEXERCISE - SAMPLE QUESTIONS FOR COMPETITIVE EXAMS
12 Videos
- HYPERBOLABOOK - CHHAYA PUBLICATIONCHAPTER - HYPERBOLAEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
1 Videos
- DIAGRAMMATIC REPRESENTATION OF DATABOOK - CHHAYA PUBLICATIONCHAPTER - DIAGRAMMATIC REPRESENTATION OF DATAEXERCISE - EXERCISE (Very short Answer Type Question)
7 Videos
- MATHEMATICAL INDUCTION BOOK - CHHAYA PUBLICATIONCHAPTER - MATHEMATICAL INDUCTION EXERCISE - Sample Questions for Competitive Exams
20 Videos
Similar Questions
Find the centre, the length of latus rectum, the eccentricity, the coordinates of foci and the equations of the directrices of the hyperbola (x+2)29−(y−1)216=1.
View SolutionThe equation px2+2qxy+ry2+2sx+2ty+u=0 (where p≠q≠r and p,q,r,s,t and u are constants ) represents-
View SolutionFind the coordinates of the foci of the rectangular hyperbola x2−y2=9
View SolutionThe following table gives the frequency distribution of heights in inches of a group of 100 peple:
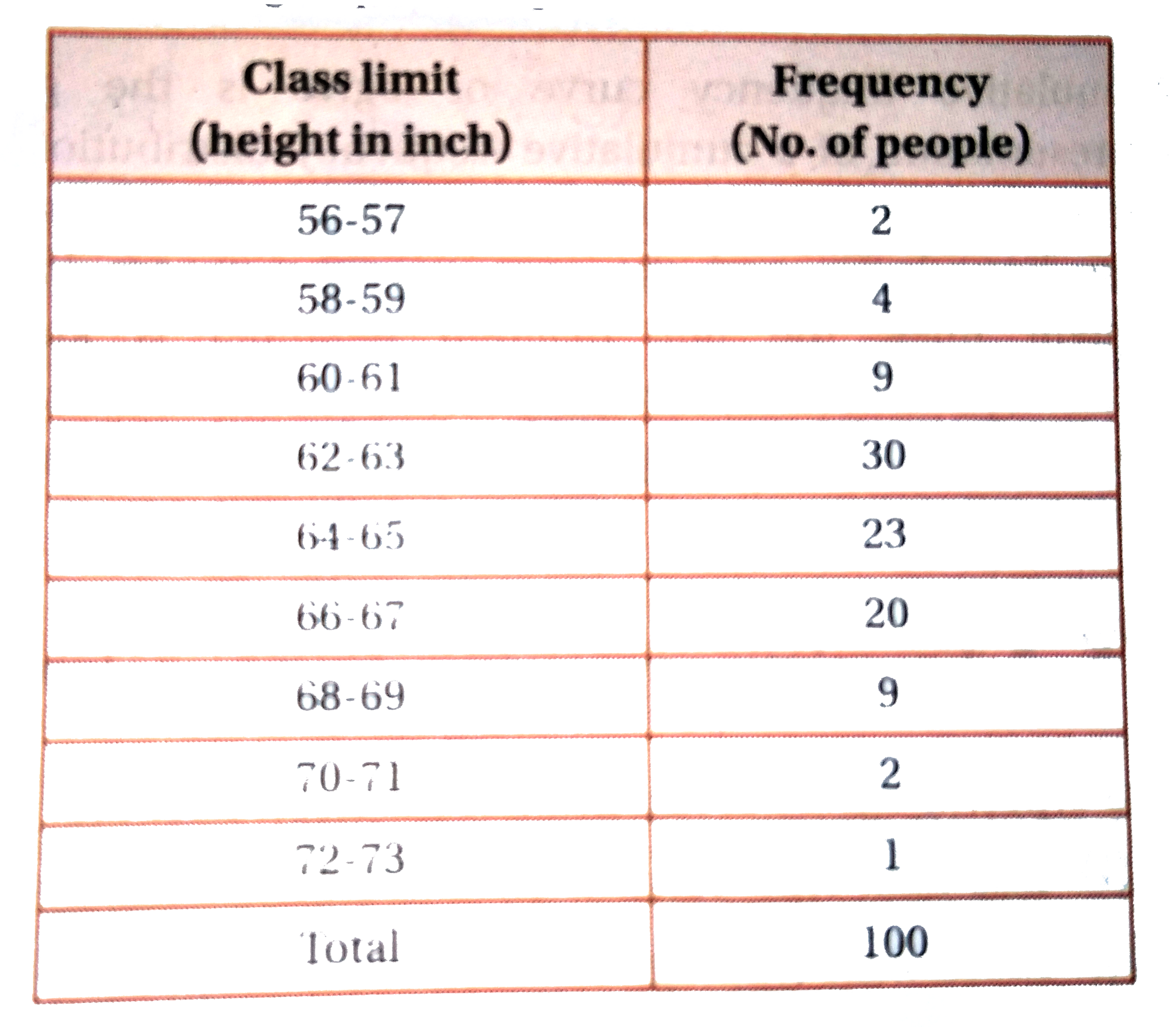 Draw a histogram for height distribution. Shade the portion of the histogram which will represent the total number of people whose heights lie between 60.5 and 66.5 inches.
Draw a histogram for height distribution. Shade the portion of the histogram which will represent the total number of people whose heights lie between 60.5 and 66.5 inches.
View SolutionIf for all n∈N and n≥1, then (32n−1) is always divisible by
View SolutionThe angle between a pair of tangents drawn from a point P to the hyperbola y^(2)=4ax is 45^(@) .Show that the locus of the point P is hyperbola.
View SolutionFind the locus of the middle points of the normals chords of the rectangular hyperbola x^(2)-y^(2)=a^(2)
View SolutionIf P is a point on the hyperbola (x^(2))/(16)-(y^(2))/(9)=1 and Q be the focus of the parabola y^(2)=8x then locus of mid-point of PQ is
View SolutionP is a variable points on the hyperbola (x^(2))/(a^(2))-(y^(2))/(b^(2))=1 whose vertex is A(a,0) The locus of the middle points AP is
View SolutionThe locus of the middle points of the chords of hyperbola x29−y24=1, which pass through the fixed point (1, 2) is a hyperbola whose eccentricity is
View SolutionP(asecϕ,atanϕ) is a variable point on the hyperbola x2−y2=a2, and A(2a,0) is a fixed point. Prove that the locus of the middle point of AP is a rectangular hyperbola.
View SolutionProve that the locus of the middle-points of the chords of the hyperbola x2a2−y2b2=1which pass through a fixed point (α,β) is a hyperbola whose centre is (α2,β2).
View SolutionThe locus of middle points of normal chords of the rectangular hyperbola x2−y2=a2 is
View SolutionFrom a point P, tangents are drawn to the hyperbola 2xy=a2 . If the chord of contact of these tangents touches the rectangular hyperbola x2−y2=a2 , prove that the locus of P is the conjugate hyperbola of the second hyperbola.
View SolutionX2−y2=16 সম পরাবৃত্তের ওপর P যেকোনো একটি বিন্দু এবং A(8,0) একটি স্থির বিন্দু। দেখাও যে, ¯¯¯¯¯¯AP রেখাংশের মধ্যবিন্দুর সঞ্চারপথ আর একটি সম পরাবৃত্ত।
View Solution
