PA and PB are two tangents drawn from point P to circle of radius 5 . A line is drawn from point P which cuts at C and D such that PC=5 and PD=15 and ∠APB=θ.
On the basis of above information answer the questions .
Number of solution(s) of the equation logcosθ(x+2)=2+3log(x+2)sin(5θ2) is
0
1
2
3
The correct Answer is:C
To solve the problem, we need to analyze the given equation step by step. The equation we need to solve is:
logcosθ(x+2)=2+3log(x+2)sin(5θ2)
Step 1: Find the value of θ
From the problem, we know that P is a point from which two tangents PA and PB are drawn to a circle of radius 5. The distances PC and PD are given as 5 and 15 respectively.
Using the Pythagorean theorem in triangle OAP (where O is the center of the circle), we have:
OP2=OA2+AP2
Given that OP=PC+OC=5+5=10 and OA=5:
102=52+AP2⟹100=25+AP2⟹AP2=75⟹AP=5√3
Now, using the cosine of half the angle θ:
cos(θ2)=APOP=5√310=√32
Thus,
θ2=π6⟹θ=π3
Step 2: Substitute θ into the equation
Now substituting θ=π3 into the equation:
logcos(π3)(x+2)=2+3log(x+2)sin(5π6)
Calculating cos(π3)=12 and sin(5π6)=12:
The equation becomes:
log12(x+2)=2+3log(x+2)(12)
Step 3: Simplify the equation
Using the change of base formula for logarithms:
log12(x+2)=−log(x+2)(x+2)=−1
Thus, we can rewrite the equation as:
−log(x+2)(x+2)=2+3(−1)
This simplifies to:
−log(x+2)(x+2)=2−3=−1
Step 4: Solve for x
Now we have:
log(x+2)(x+2)=1
This implies:
x+2=(x+2)1⟹x+2=2⟹x=0
Step 5: Check for other solutions
Now, we also need to consider the case when:
log(x+2)(12)=−1
This gives:
x+2=12⟹x=−32
Conclusion
Thus, we have two solutions:
1. x=0
2. x=−32
Therefore, the number of solutions to the equation is 2.
2. x=−32
Topper's Solved these Questions
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - EXAMPLES ( Matching Type Questions )
1 Videos
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - EXAMPLES ( Subjective Type Examples )
2 Videos
- THREE DIMENSIONAL COORDINATE SYSTEMBOOK - ARIHANT MATHS ENGLISHCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Three Dimensional Coordinate System Exercise 12 : Question Asked in Previous Years Exam
2 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
19 Videos
Similar Questions
PA and PB are two tangents drawn from point P to circle of radius 5 . A line is drawn from point P which cuts at C and D such that PC=5 and PD=15 and ∠APB=θ.
On the basis of above information answer the questions .
Area of ΔAPB is
View SolutionPA and PB are two tangents drawn from point P to circle of radius 5 . A line is drawn from point P which cuts at C and D such that PC=5 and PD=15 and ∠APB=θ.
On the basis of above information answer the questions .
value of sin2theta + cos4theta +sin5theta+tan7theta +cos 8theta
View SolutionIf (5+2√6)x2−8+(5−2√6)x2−8=10,x∈R
On the basis of above information, answer the following questions :
Number of solution (s) of the given equation is/ are -
View SolutionPA and PB are two tangents drawn from an external point P to a circle with centre C and radius=4cm If PA⊥PB then length of each tangent is
View SolutionThe number of solutions of the equation sin2θ−2cosθ+4sinθ=4 in [0,5π] is equal to
View SolutionConsider a circle , in which a point P is lying inside the circle such that (PA)(PB)=(PC)(PD) ( as shown in figure ) .
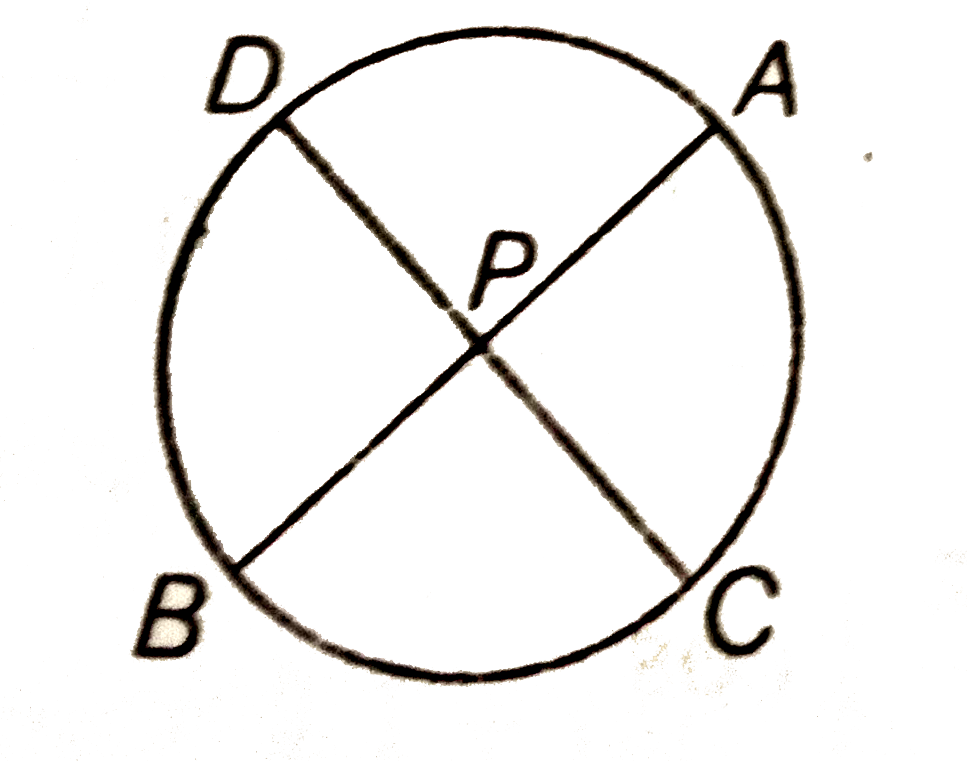
On the basis of above information , answer the questions
If logPAx=2,logPBx=3,logxPC=4, then logPD x is equal to
View SolutionConsider a circle , in which a point P is lying inside the circle such that (PA)(PB)=(PC)(PD) ( as shown in figure ) .
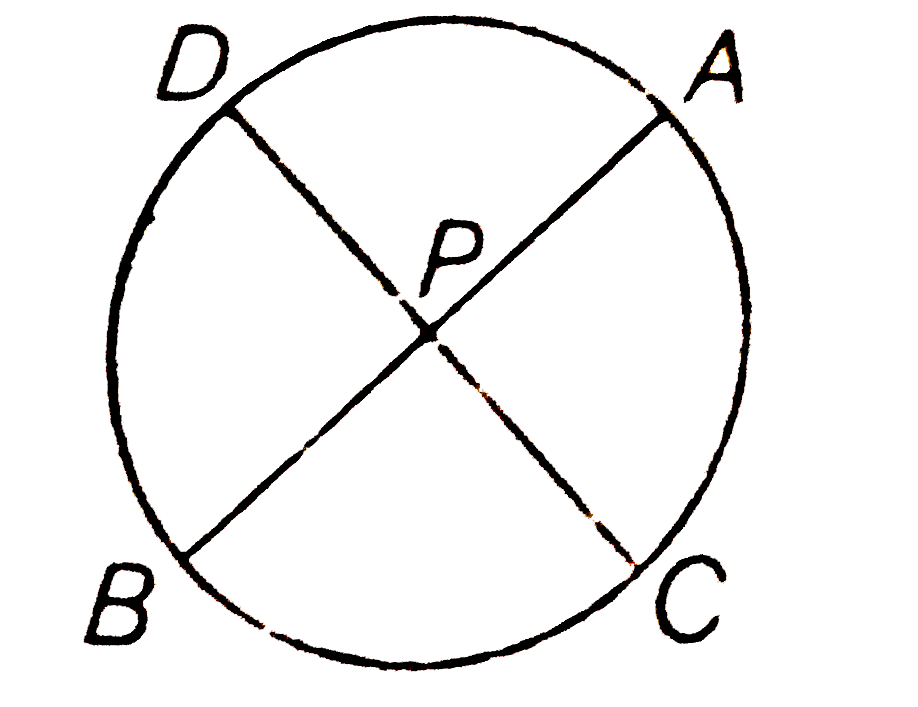
On the basis of above information , answer the questions
If PA=|cosθ+sinθ|andPB=|cosθ−sinθ|, then maximum value of (PC)(PD) , is equal to
View SolutionThe number of solution satisfying the equations tan4θ=cot5θ and sin2θ=cosθ in [0,2π] is
View SolutionNumber of solutions of the equation sin2θ+2=4sinθ+cosθ lying in the interval [π,5π] is
View SolutionConsider a circle , in which a point P is lying inside the circle such that (PA)(PB)=(PC)(PD) ( as shown in figure ) .
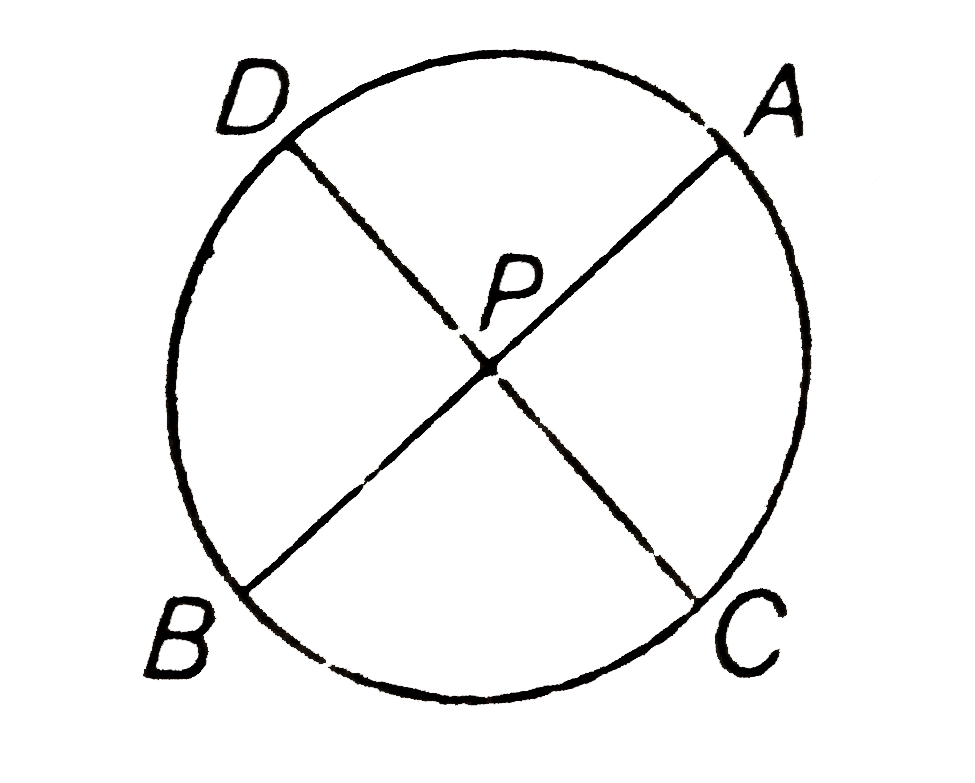
On the basis of above information , answer the question:
Let PA=4 , PB=3 cm and CD is diameter of the circle having the length 8 cm. If PC>PD , then PCPD is equal to
View Solution
