PARAGRAPH X Let S be the circle in the xy -plane defined by the equation x2+y2=4. (For Ques. No 15 and 16) Let E1E2 and F1F2 be the chords of S passing through the point P0(1, 1) and parallel to the x-axis and the y-axis, respectively. Let G1G2 be the chord of S passing through P0 and having slope −1 . Let the tangents to S at E1 and E2 meet at E3 , the tangents to S at F1 and F2 meet at F3 , and the tangents to S at G1 and G2 meet at G3 . Then, the points E3, F3 and G3 lie on the curve x+y=4 (b) (x−4)2+(y−4)2=16 (c) (x−4)(y−4)=4 (d) xy=4Solution in Tamil
x+y=4
(x−4)2+(y−4)2=16
(x−4)(y−4)=4
xy=4
The correct Answer is:A
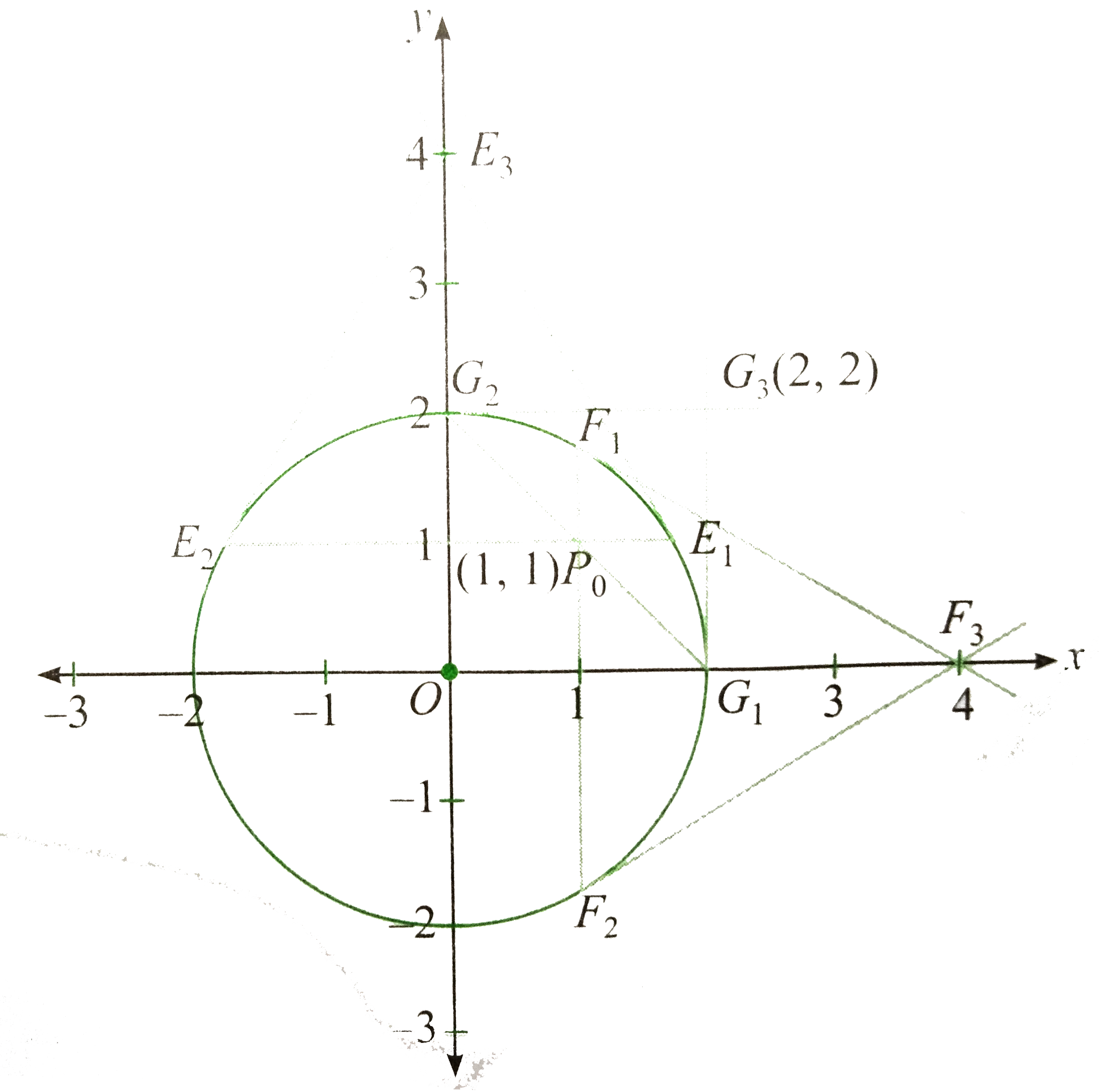
Circle is x2+y2=4.
Equation of chord through P0(1,1) parallel to x-axis is y=1
Solving this with circle, we E1(√3,1) and E2(−√3,1).
Equations of tangent to circle at E1 and E2 are √3x+y=4 and −√3x+y=4 , respectively.
These tangents meet at E3(0,4).
Chord through p0(1,1), parallel to y -axis is x=1, which meets circle at F1(1,√3) and F2(1,−√3).
Tangents to circle at F1 and F2 meet at F3(0,4).
Chord through P0 having slope -1 is x+y=2 which meets circle at G1(0,2) and G2(2,0).
Tangest to circle at G1 and G2 meet at G3(2,2).
Clearly, E3,F3 and G3 lie on line x+y=4.
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - MATRIX MATCH TYPE
6 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - JEE Main Previous Year
10 Videos
- BINOMIAL THEOREMBOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
16 Videos
Similar Questions
Find the derivative of y=ln(cosx2).
View SolutionIf P and Q are the points of intersection of the circles x2+y2+3x+7y+2p=0 and x2+y2+2x+2y−p2=0 then there is a circle passing through P,Q and (1,1) for
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
View SolutionTangents are drawn from points on the line x+2y=8 to the circle x^(2)+y^(2)=16. If locus of mid-point of chord of contacts of the tangents is a circles S, then radius of circle S will be
View SolutionLet RS be the diameter of the circle x2+y2=1, where S is the point (1,0) Let P be a variable apoint (other than RandS) on the circle and tangents to the circle at SandP meet at the point Q.The normal to the circle at P intersects a line drawn through Q parallel to RS at point E. then the locus of E passes through the point(s)- (A) (13,1√3) (B) (14,12) (C) (13,−1√3) (D) (14,−12)
View SolutionLet a tangent at a point P to the ellipse (x^(2))/(a^(2))+(y^(2))/(b^(2))=1 meets directrix at Q and S be the correspondsfocus then /_PSQ
View SolutionPARAGRAPH X Let S be the circle in the xy -plane defined by the equation x2+y2=4. (For Ques. No 15 and 16) Let E1E2 and F1F2 be the chords of S passing through the point P0(1, 1) and parallel to the x-axis and the y-axis, respectively. Let G1G2 be the chord of S passing through P0 and having slope −1 . Let the tangents to S at E1 and E2 meet at E3 , the tangents to S at F1 and F2 meet at F3 , and the tangents to S at G1 and G2 meet at G3 . Then, the points E3, F3 and G3 lie on the curve x+y=4 (b) (x−4)2+(y−4)2=16 (c) (x−4)(y−4)=4 (d) xy=4
View SolutionLet S be the circle in the xy -plane defined by the equation x2+y2=4. (For Ques. No 15 and 16) Let P be a point on the circle S with both coordinates being positive. Let the tangent to S at P intersect the coordinate axes at the points M and N . Then, the mid-point of the line segment MN must lie on the curve (x+y)2=3xy (b) x2/3+y2/3=24/3 (c) x2+y2=2xy (d) x2+y2=x2y2
View SolutionLet E1E2andF1F2 be the chords of S passing through the point P∘ (1, 1) and parallel to the X-axis and the Y-axis, respectively. Let G1G2 be the chord of S passing through P∘ and having slope -1. Let the tangents to S at E1andE2 met at F3, and the tangents to S at G1andG2 meet at G3. Then , the points E3,F3andG3 lie on the curve
Let S be the circle in the XY-plane defined by the equation
x2+y2=4
View SolutionPoint (s) at which the tangent to curve y=11+x2 is parallel to the X - axis are
View Solutionमाना कि S एक वृत्त है जो xy समतल में समीकरण x2+y2=4 के द्वारा परिभाषित है।
माना कि E1E2 और F1F2 वृत्त S की ऐसी जीवाएं हैं जो बिन्दु P0(1,1) से गुजरती हैं और क्रमश: x-अक्ष व y-अक्ष के समान्तर हैं। माना कि G1G2,S की वह जीवा है जो P0 से गुजरती है और जिसकी प्रवणता -1 है। माना कि E1 और E2 पर S की स्पर्शियां E3 पर मिलती हैं, F1 और F2 पर S की स्पर्शियां F3 पर मिलती हैं, तथा G1 और G2 पर S की स्पर्शियां G3 पर मिलती हैं। तब बिन्दु E3,F3 और G3 से गुजरने वाले वक्र का समीकरण है
View SolutionAngle between the tangents drawn from the point (5,4) to the ellipse x225+y216=1 is
View Solutionমনে করো , xy সমতলে S একটি বৃত্ত, যা x2+y2=4 সমীকরণ দ্বারা সংজ্ঞাত। মনে করো , P0(1,1) বিন্দুগামী এবং x অক্ষ ও y-অক্ষের সঙ্গে সমান্তরাল, S-এর দুটি জ্যা হল যথাক্রমে E1E2 ও F1F2,। মনে করো , -1 নতিমাত্রাবিশিষ্ট এবং P0 বিন্দুগামী S -এর একটি জ্যা হল G1G2 ,। মনে করো , S- এর E1 ও E2, বিন্দুতে স্পর্শকদ্বয় পরস্পরকে E3, বিন্দুতে, F1 ও F2, বিন্দুতে স্পর্শদ্বয় পরস্পরকে F3 বিন্দুতে এবং G1 ও G2, বিন্দুতে স্পর্শকদ্বয় পরস্পরকে G3, বিন্দুতে ছেদ করে। তাহলে E_3,F_3ওG_3 বিন্দুগুলি যে বক্রের ওপর অবস্থিত তা হল
View SolutionEquation of a tangent to the ellipse x236+y225=1, passing through the point where a directrix of the ellipse meets the + ve x-axis is
View Solution
