Point A and B are in the first quadrant,point O is the origin. If the slope of OA is 1,slope of OB is 7 and OA=OB,Then slope of AB is:Solution in Bengali
−1/5
−1/4
−1/3
−1/2
The correct Answer is:D
tanθ=7
OA=OB=r
sinθ=75√2cosθ=15√2
Now, mAB=1/2.
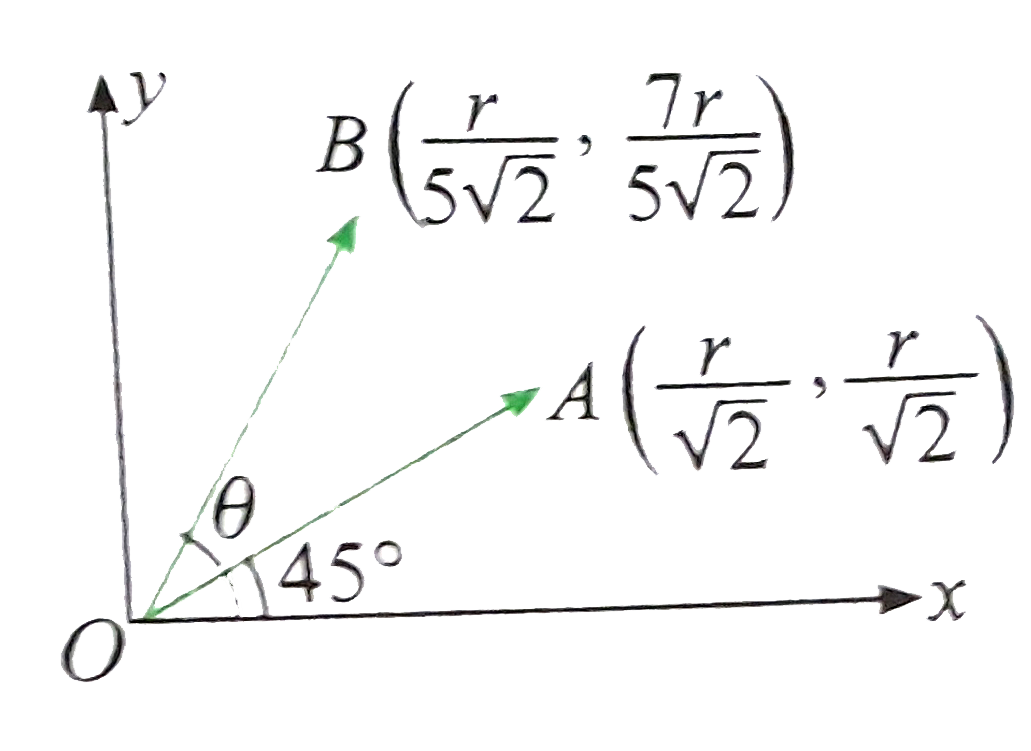
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Multiple correct
13 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Linked
10 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.6
9 Videos
- COORDINATE SYSTEMBOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGE PUBLICATIONCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
If (-6,-4), (3,5),(-2,1) are the vertices of a parallelogram, then remaining vertex cannot be
View SolutionFor points P=(x1,y1)and Q=(x2,y2) of the co-ordinate plane a new distance d(P,Q)=|x1−x2|+|y1−y2| is defined .Let O(0,0)and A(3,2). The set of points in first quadrant which are equidistant from O and A is
View SolutionFind the locus of a point whose distance from (a, 0) is equal to its distance from the y-axis.
View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
View SolutionPoint A and B are in the first quadrant,point O is the origin. If the slope of OA is 1,slope of OB is 7 and OA=OB,Then slope of AB is: a. -1/5 b. -1/4 c. -1/3 d. -1/2
View SolutionPoint A and B are in the first quadrant; point O is the origin. If the slope of OA is 1, the slope of OB is 7, and OA=OB, then the slope of AB is −15 (b) −14 (c) −13 (d) −12
View SolutionA straight line with negative slope passing through the point (2,8) cuts the cordinate axes at A and B then minimum value of OA+OB (where 0 is origin) is equal to
View SolutionIf AB is a focal chord of the parabola y^(2)=4ax whose vertex is 0, then(slope of OA ) x (slope of OB)-
View SolutionLety=x line is median of the triangle OAB where O is origin. Equation ax2+2hxy+by2=0,a,h,b∈N, represents combined equation of OA and OB. A and B lie on the ordinate x=3. If slope of OA is twice the slope of OB, then greatest possible value of a+2h+b is
View SolutionA strainght line L with negative slope passes through the point (1,1) and cuts the positve coordinates axes at the points A and B . If O is the origin , thene the minimum value of OA+OBas L varies , is
View SolutionA and B are two points on the hyperbola O is the centre. If OA is perpendicular to OB then 1(OA)2+1(OB)2 is equal to
View SolutionPoint A and B are in the first quadrant; point O is the origin. If the slope of OA is 1, the slope of OB is 7, and OA=OB, then the slope of AB is −15 (b) −14 (c) −13 (d) −12
View SolutionPoint A and B are in the first quadrant,point O is the origin. If the slope of OA is 1,slope of OB is 7 and OA=OB,Then slope of AB is: a. -1/5 b. -1/4 c. -1/3 d. -1/2
View SolutionLety=x line is median of the triangle OAB where O is origin. Equation ax2+2hxy+by2=0,a,h,b∈N, represents combined equation of OA and OB. A and B lie on the ordinate x=3. If slope of OA is twice the slope of OB, then greatest possible value of a+2h+b is
View Solutionबिन्दु A तथा B प्रथम चतुर्थांश में है, तथा बिन्दु मूल बिन्दु है। यदि OA की प्रवणता 1,OB की प्रवणता 7 तथा OA=OB है, तो AB की प्रवणता है -
View Solution
