P,Q and R represent switches in on position and P',Q' and R' represent switches in off position. Construct a switching circuit representing the polynomial PR+Q(Q'+R)(P÷QR) . Using Boolean Algebra, simplify the polynomial expression and construct the simplified circuit
To solve the given problem, we need to simplify the polynomial expression PR+Q(Q'+R)(P−QR) using Boolean algebra and then construct the corresponding switching circuit. Let's break down the steps:
Step 1: Write down the original expression
The original expression is:
PR+Q(Q′+R)(P−QR)
Step 2: Simplify the expression inside the parentheses
First, we simplify Q(Q′+R):
- Q′ is the complement of Q.
- The expression Q(Q′+R) can be expanded using the distributive law:
Q(Q′+R)=QQ′+QR
Step 3: Apply the Complement Law
Using the Complement Law, QQ′=0:
QQ′+QR=0+QR=QR
So, we can rewrite the expression as:
PR+QR(P−QR)
Step 4: Expand the expression
Now we need to expand QR(P−QR):
QR(P−QR)=QRP−QR2
Since QR2=QR (because R⋅R=R), we have:
QR(P−QR)=QRP−QR
Step 5: Combine the terms
Now we can combine the terms:
PR+QRP−QR
Step 6: Factor out common terms
Notice that PR+QRP can be factored:
PR(1+Q)−QR
Since 1+Q=1 (using the Identity Law):
PR−QR
Step 7: Final simplified expression
Thus, the simplified expression is:
PR+QR
Step 8: Construct the switching circuit
To construct the switching circuit:
1. For PR: Connect switch P and switch R in series.
2. For QR: Connect switch Q and switch R in series.
3. Combine both outputs: Use an OR gate to combine the outputs of the two series connections.
Final Circuit Diagram
The circuit will have:
- Two series connections: one for PR and another for QR.
- An OR gate that takes the output from both series connections.
The original expression is:
PR+Q(Q′+R)(P−QR)
First, we simplify Q(Q′+R):
- Q′ is the complement of Q.
- The expression Q(Q′+R) can be expanded using the distributive law:
Q(Q′+R)=QQ′+QR
Using the Complement Law, QQ′=0:
QQ′+QR=0+QR=QR
So, we can rewrite the expression as:
PR+QR(P−QR)
Now we need to expand QR(P−QR):
QR(P−QR)=QRP−QR2
Since QR2=QR (because R⋅R=R), we have:
QR(P−QR)=QRP−QR
Now we can combine the terms:
PR+QRP−QR
Notice that PR+QRP can be factored:
PR(1+Q)−QR
Since 1+Q=1 (using the Identity Law):
PR−QR
Thus, the simplified expression is:
PR+QR
To construct the switching circuit:
1. For PR: Connect switch P and switch R in series.
2. For QR: Connect switch Q and switch R in series.
3. Combine both outputs: Use an OR gate to combine the outputs of the two series connections.
The circuit will have:
- Two series connections: one for PR and another for QR.
- An OR gate that takes the output from both series connections.
Topper's Solved these Questions
- MATHEMATICS - 2014BOOK - ICSECHAPTER - MATHEMATICS - 2014EXERCISE - Section-B
6 Videos
- MATHEMATICS - 2014BOOK - ICSECHAPTER - MATHEMATICS - 2014EXERCISE - Section-C
6 Videos
- MATHEMATICS - 2013BOOK - ICSECHAPTER - MATHEMATICS - 2013EXERCISE - Section-C
6 Videos
- MATHEMATICS -2017BOOK - ICSECHAPTER - MATHEMATICS -2017EXERCISE - Section-B
10 Videos
Similar Questions
A, B and C represent switches in 'on' position and A', B' and C' represent them in 'off' position. Construct a switching circuit representing the polynomial ABC + AB'C + A'B'C. Using Boolean Algebra, prove that the given polynomial can be simplified to C (A + B'). Construct an equivalent switching circuit.
View SolutionConstruct a circuit diagram for the following Boolean Function
(BC+A)(A'B'+C')+A'B'C'
Using laws of Boolean Algebra, simplify the function and draw the simplified circuit.
View SolutionWrite the Boolean function coresponding to the switching circuit given below:

A, B and C represent switches in 'on' position and A', B' and C' represent them in 'off' position. Using Boolean algebra, simplify the function and construct an equivalent switching circuit.
View SolutionThe Boolean expression P+¯¯¯PQ, where P and Q are the inputs of the logic circuit, represents
View SolutionIdentify P, Q, R in the composition of green house gases represented below
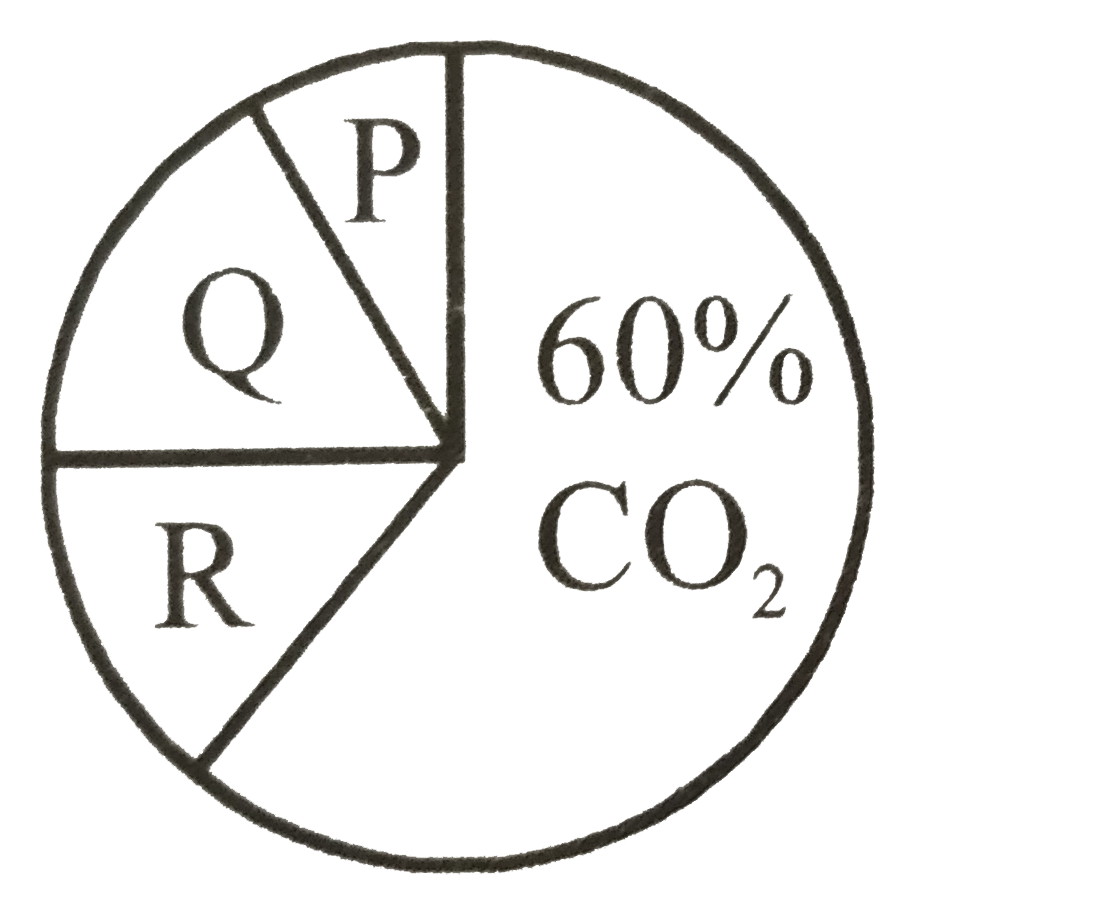
View SolutionIn electrocardiography P-Q interval represents :
View SolutionIf p,q and r are simple propositions such that (p∧q)∧(q∧r) is true, then
View SolutionConstruct the truth table (~p∧~q)∨(p∧~q)
View SolutionConstrust a circuit for the statements (p∧q∧r)∨[(~p)∧(~q)]
View SolutionWrite →PQ+→RP+→QR in the simplified form.
View Solution
