PQRS is a parallelogram. PXandQY are respectively, the perpendiculars from PandQ to SRandRS produced. Prove that PX=QY
"here
PS=QR( opposite sides of parallelogram are equal)
∠PSR+∠QRS=180∘
∠PSR=180∘−∠QRS.....(1)
∠2+∠QRS=180∘
∠2=180∘−∠QRS....(2)
∠2=∠PSR=∠1
In△PXSand △QRY
PS=QR and
∠1=∠2,
∠PXS=∠PYRfrom AAS
△PXS≅△QYR
from CPCT,
PX=QY"
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
PQRS is a parallelogram. PX and QY are respectively, the perpendiculars from P and Q to SR and RS produced. then
APX=QYBSX=RYCBoth A and BDNone - Question 2 - Select One
If r is the geometric mean of p and q, then the line px+qy+r=0
Ahas a fixed directionBpasses thorugh a fixed pointCforms with the axs a triangle of constant areaDsum of its intercepts on the axes is constant
In the adjoining figure, PQRS is a parallelogram. A and B are the mid-points of PQ of SR respectively. If PS= BR, then prove that quadrilateral ADBC is a reactangle.
 View Solution
View SolutionPQRS is a parallelogram. PS is produced to M, so that SM = SR and MR produced meets PQ produced at N. Prove that QN = QR.
View SolutionPQRS is a parallelogram. PS is produced to M, so that SM = SR and MR produced meets PQ produced at N. Prove that QN = QR.
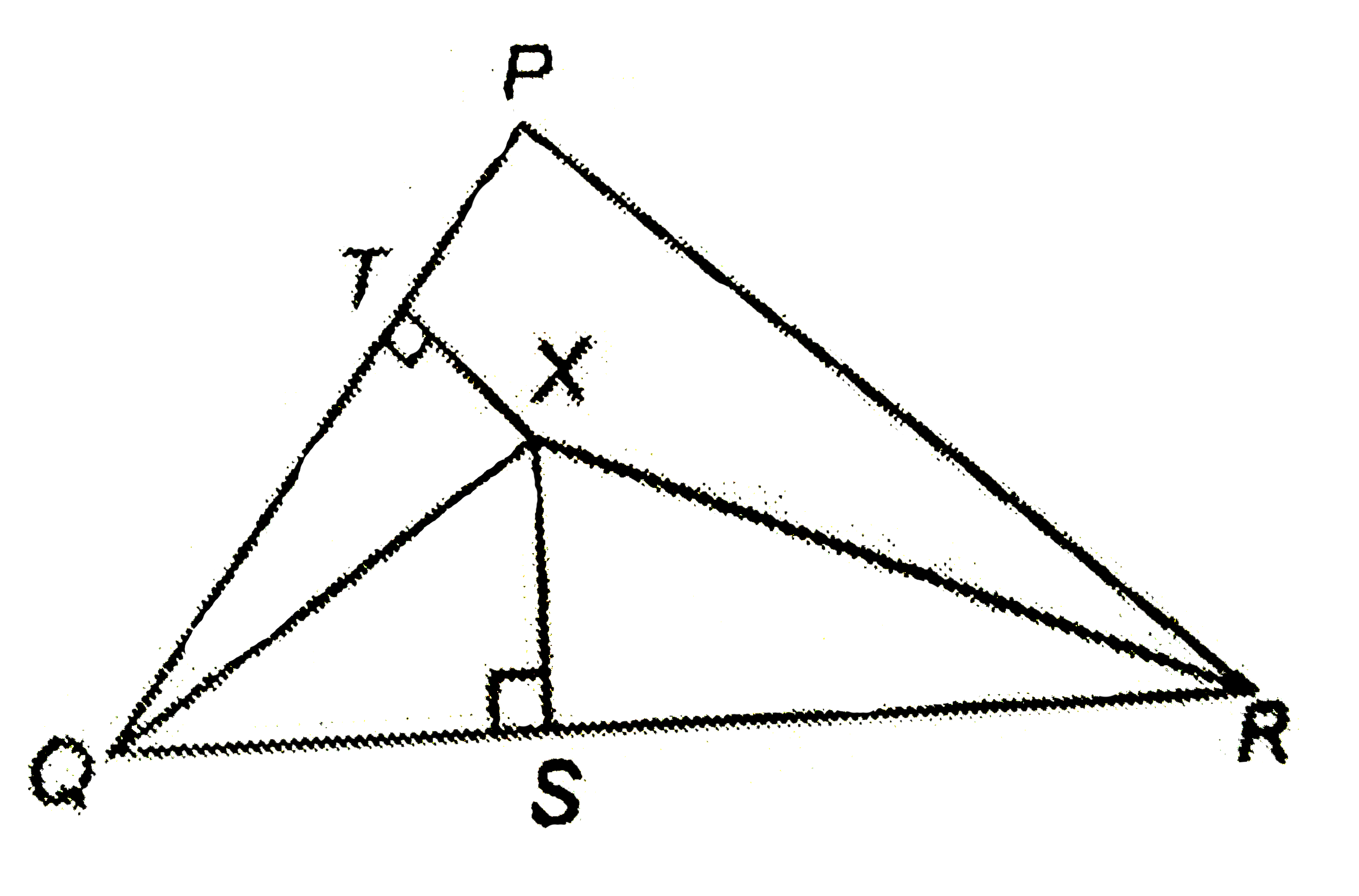
View SolutionIn the adjoining figure, QX and RX are the bisectors of the angles Q and R respectively of the angles Q and R respectively of the triangle PQR. If XS⊥PQ. Prove that :
(i) ΔXTQ≅ΔXSQ
(ii) PX bisects angle P.
 View Solution
View SolutionIn the given figures, if PQRS is a parallelogram and AB||PS, then prove that OC||SR.
View SolutionIf r is the geometric mean of p and q, then the line px + qy + r = 0
View Solution

