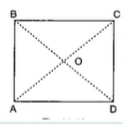
Show that the diagonals of a square are equal and bisect each other at right angles.

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

(i)In a △ A B C and   △ B A D
A B = A B (common line)
B C = A D (opppsite sides of a square)
∠ A B C = ∠ B A D = 90 ∘
△ A B C ≅ △ B A D ( By SAS property)
A C = B D ( by CPCT).
(ii) In a, △ O A D and △ O C B
A D = C B (opposite sides of a square)
∠ O A D = ∠ O C B (transversal AC)
∠ O D A = ∠ O B C (transversal BD)
△ O A D ≅ △ O C B (ASA property)
O A = O C (i)
O B = O D (ii)
From (i) and (ii) AC and BD bisect each other.
Now in a △ O B A and   △ O D A , O B = O D (from (ii))
B A = D A
O A = O A (common line)
△ A O B = △ A O D (iii)(by CPCT)
∠ A O B + ∠ A O D = 180 ∘ (linear pair)
2 ∠ A O B = 180 ∘
∠ A O B = ∠ A O D = 90 ∘
AC and BD bisect each other at right angles.

Updated on:21/07/2023
|