Prove that in two concentric circles, the chord of the larger circle, which touches the smaller circle, is bisected at the point of contact.
To prove that in two concentric circles, the chord of the larger circle, which touches the smaller circle, is bisected at the point of contact, we can follow these steps:
1. Define the Circles and Points:
Let O be the common center of two concentric circles C1 (the smaller circle) and C2 (the larger circle). Let AB be a chord of circle C2 that touches circle C1 at point P.
Hint: Identify the center and the points involved in the problem clearly.
2. Identify the Tangent and Radius:
Since AB is a tangent to the smaller circle C1 at point P, we know that the radius OP (from the center O to the point of contact P) is perpendicular to the tangent AB.
Hint: Recall the property that a tangent to a circle is perpendicular to the radius at the point of contact.
3. Draw the Perpendicular:
Draw the line segment OP. Since OP is perpendicular to AB, it bisects the chord AB at point P.
Hint: Remember that any line drawn from the center of a circle that is perpendicular to a chord bisects that chord.
4. Congruent Triangles:
Now, consider triangles OAP and OBP. We have:
- OA = OB (radii of circle C2)
- OP = OP (common side)
- Angle OAP = Angle OBP = 90 degrees (since OP is perpendicular to AB)
Hint: Use the properties of triangles and the fact that they share a side to establish congruence.
5. Apply RHS Congruence Criterion:
By the RHS (Right angle-Hypotenuse-Side) congruence criterion, triangles OAP and OBP are congruent.
Hint: Congruent triangles have corresponding sides that are equal.
6. Conclude that AP = PB:
Since triangles OAP and OBP are congruent, we can conclude that AP = PB.
Hint: Use the property of congruent triangles to state that corresponding parts are equal.
Final Conclusion:
Thus, we have proved that the chord AB of the larger circle C2, which touches the smaller circle C1 at point P, is bisected at point P, meaning AP = PB.
Let O be the common center of two concentric circles C1 (the smaller circle) and C2 (the larger circle). Let AB be a chord of circle C2 that touches circle C1 at point P.
Since AB is a tangent to the smaller circle C1 at point P, we know that the radius OP (from the center O to the point of contact P) is perpendicular to the tangent AB.
Draw the line segment OP. Since OP is perpendicular to AB, it bisects the chord AB at point P.
Now, consider triangles OAP and OBP. We have:
- OA = OB (radii of circle C2)
- OP = OP (common side)
- Angle OAP = Angle OBP = 90 degrees (since OP is perpendicular to AB)
By the RHS (Right angle-Hypotenuse-Side) congruence criterion, triangles OAP and OBP are congruent.
Since triangles OAP and OBP are congruent, we can conclude that AP = PB.
Thus, we have proved that the chord AB of the larger circle C2, which touches the smaller circle C1 at point P, is bisected at point P, meaning AP = PB.
Topper's Solved these Questions
Similar Questions
Knowledge Check
In two Concentric circles, AB and CD are two chords of the outer circle which touch the inner circle at E and F. Then :
AAB = CDBAB=12CDCAB≠CDDNone of theseTwo concentric circles are of radii 13 cm and 5 cm. The length of the chord of the larger circle which touches the smaller circle is:
दो संकेंद्री वृत्तों की त्रिज्या 13 सेमी तथा 5 सेमी है। बड़े वृत्त की जीवा की लंबाई ज्ञात कीजिए, जो छोटे वृत्त को स्पर्श करती है।A6 cmB7 cmC10 cmD8 cmLet two chords AB and AC of the larger circle having same centre touch the smaller circle having same centre at X and Y. Then XY = ?
ABCB12BCC13BCD14BC
In two concentric circles, a chord of length 8cm of the larger circle touches the smaller circle. If the radius of the larger circle is 5cm then find the radius of the smaller circle.
View SolutionTwo concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
View SolutionTwo concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
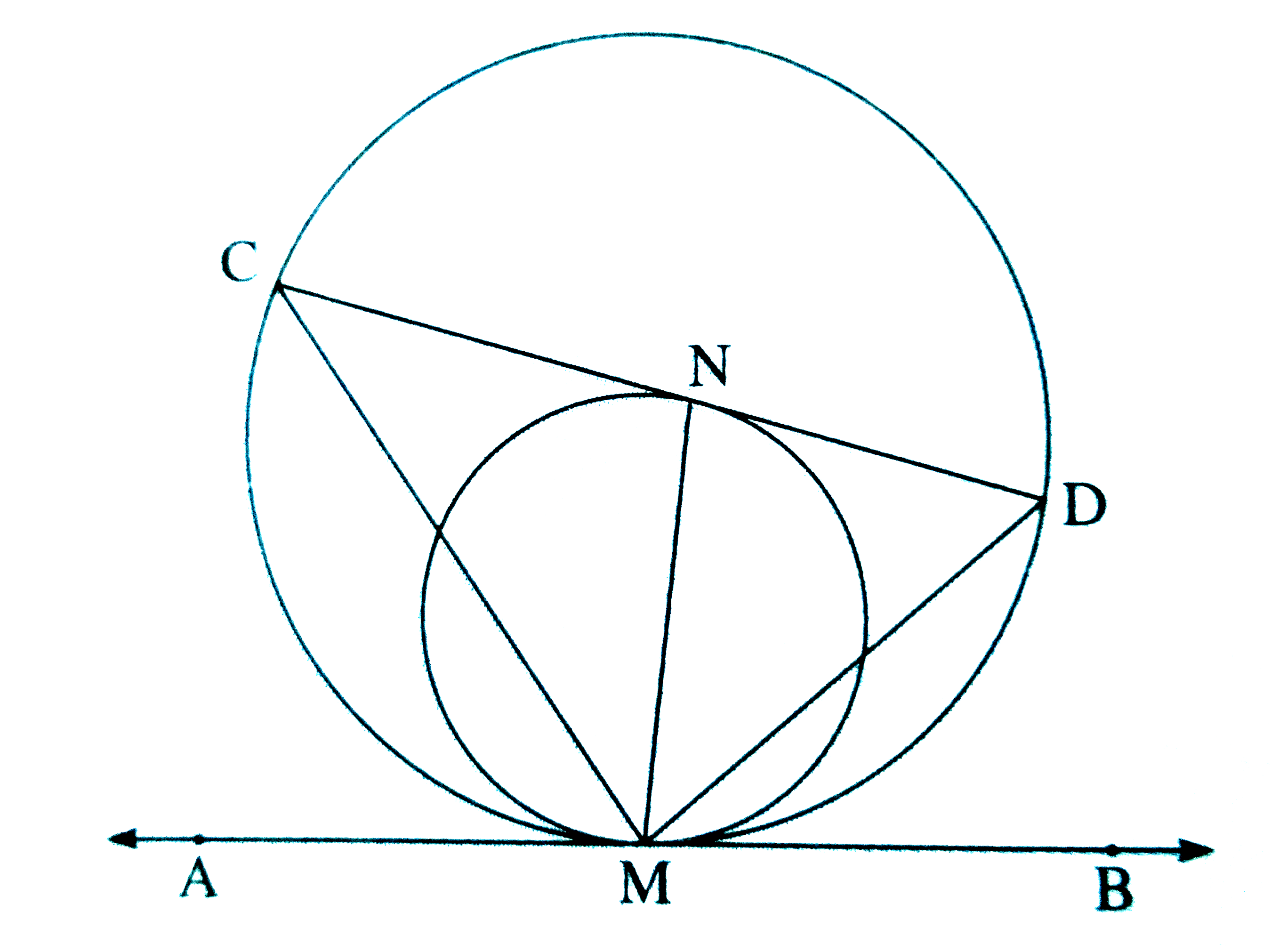
View SolutionIn the figure, M is the point of contact of two internally touching circles. The chord CD of the bigger circle touches the smaller circle at point N. Line AMB is their common tangent. Prove MN bisects ∠CMD
View SolutionTwo concentric circles are of radii 6.5cm and 2.5cm. Find the length of the chord of the larger circle which touches the smaller circle.
View Solution
