In two concentric circle, prove that a chord of larger circle which is tangent to smaller circle is bisected at the point of contact.
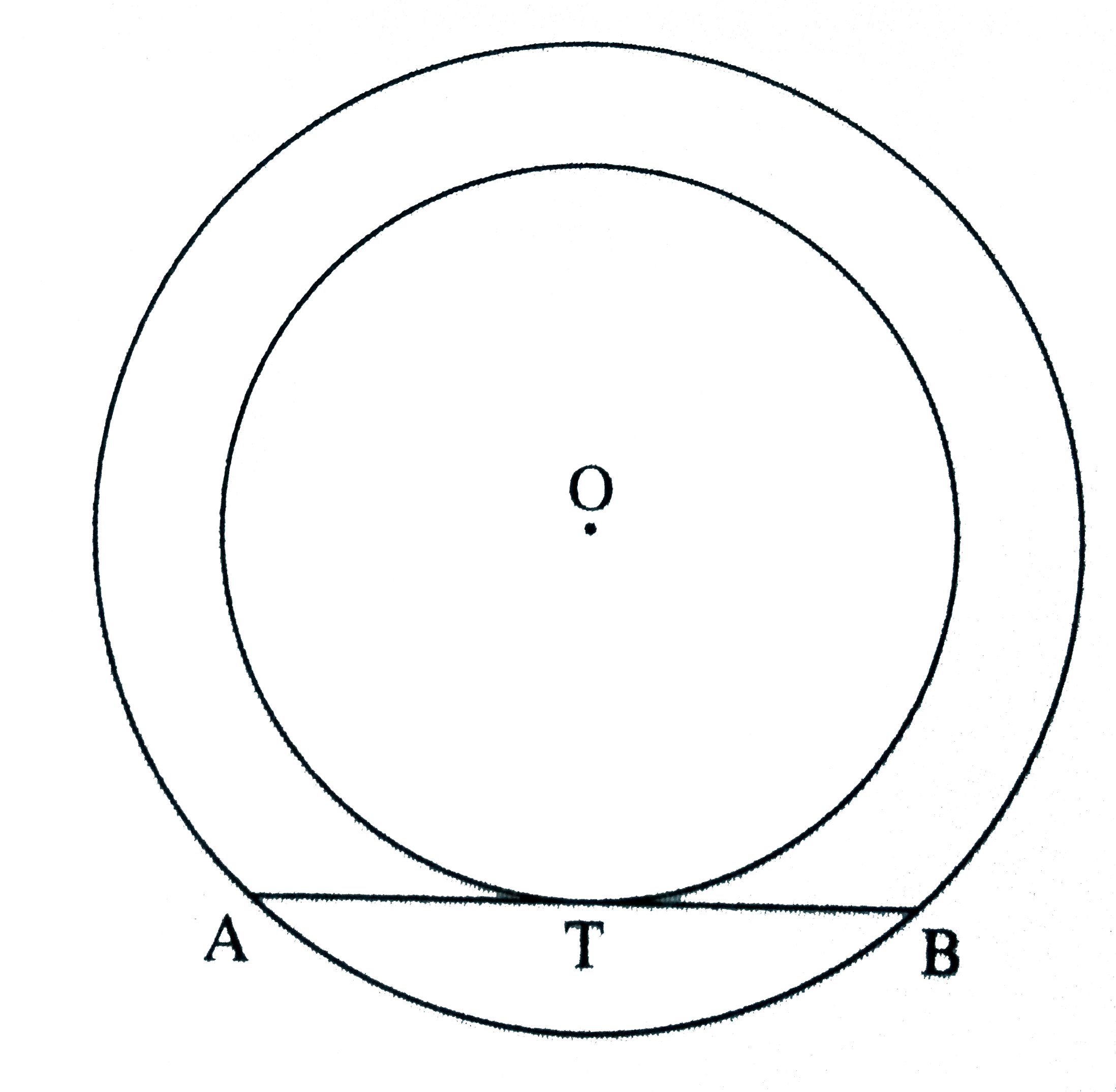
Assume, O be the common centre of two concentric circles, and Assume A B be a chord of the larger circle touching the smaller circle at P.
prove: AB is bisected at P.
Join OP
As OP is the radius of the smaller circle and AB is a tangent to this circle at a point P.
So, OP⊥AB
Given: the perpendicular drawn from the centre of a circle to any chord of the circle, bisects the chord.
So, OP⊥AB
⇒AP=BP
Hence,AB is bisected at P
Topper's Solved these Questions
Similar Questions
Prove that in two concentric circles, the chord of the larger circle which touches the smaller circle is bisected at the point of contact.
View SolutionProve that in two concentric circles, the chord of the larger circle, which touches the smaller circle, is bisected at the point of contact.
View Solution
Knowledge Check
- Question 1 - Select One
The radius of two concentric circles are 9 cm and 15 cm. If the chord of the greater circle be a tangent to the smaller circle, then the length of that chord is
A24 cmB12 cmC30 cmD18 cm - Question 2 - Select One
In two Concentric circles, AB and CD are two chords of the outer circle which touch the inner circle at E and F. Then :
AAB = CDBAB=12CDCAB≠CDDNone of these
In two concentric circles prove that all chords of the outer circle which touch the inner circle are of equal length.
View SolutionIn two concentric circles, a chord of length 24cm of larger circle becomes a tangent to the smaller circle whose radius is 5cm. Find the radius of the larger circle.
View SolutionIf two concentric circles, a chord of length 24 cm of larger circle becomes a tangent to the smaller circle whose radius is 5 cm. Find the radius of the larger circle.
View SolutionIn the figure, two concentric circles are given and line AB is tangent to the smaller circle at T. Show that T is the midpoint of seg AB.
 View Solution
View SolutionIn two concentric circles, a chord of length 8cm of the larger circle touches the smaller circle. If the radius of the larger circle is 5cm then find the radius of the smaller circle.
View SolutionTwo concentric circle of radii 13cm and 12 cm. What is the length of the chord of the larger circle which is tangent to the smaller circle?
View Solution

