Prove that the line joining the mid-points of two parallel chords of a circle passes through the centre.
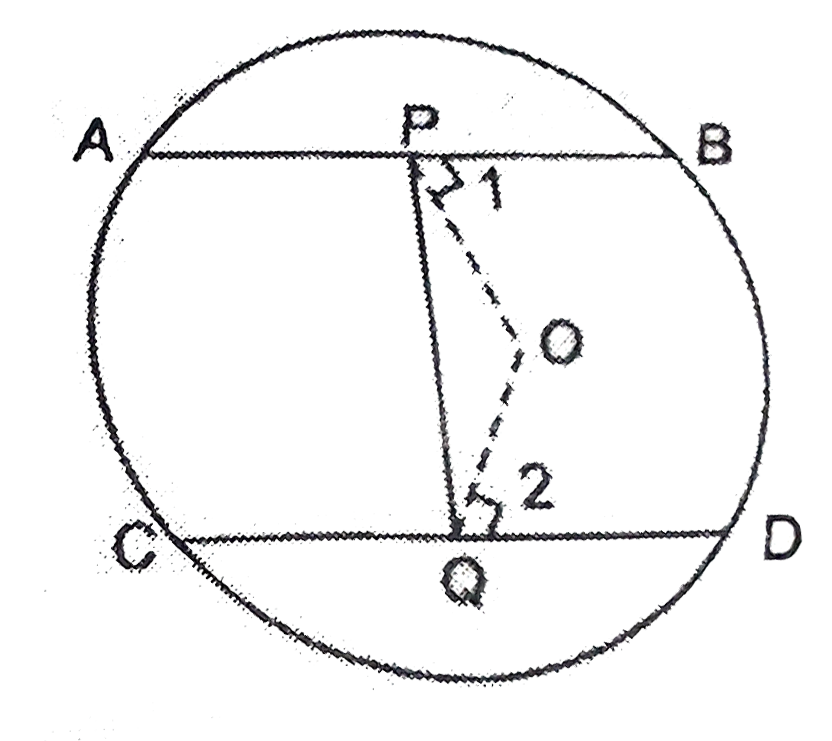
Let AB and CD be two parallel chords having P and Q as their mid-points respectively. Let O be the centre of the circle. Join OP and OQ.
Now, P is the mid-points of AB.
∴OP⊥AB
⇒∠BPO=90∘
Similarly, Q is the mid-point of CD.
⇒OQ⊥CD
⇒∠DQO=90∘.
∴∠BPO+∠DQO=90∘+90∘=180∘
Also, AB∣∣CD (given)
∴POQ must be a transversal i.e. a striaght line.
⇒PQ is a striaght line passing through O i.e. the centre of circle.
Topper's Solved these Questions
- CIRCLE BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLE EXERCISE - Exercise 10a
22 Videos
- CIRCLE BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLE EXERCISE - Exercise 10b
19 Videos
- AREA OF PARALLELOGRAMS AND TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Revision Exercise (long Answer Question)
5 Videos
- CO-ORDINATE GEOMETRY BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CO-ORDINATE GEOMETRY EXERCISE - Exercise
8 Videos
Similar Questions
Prove that the line joining the mid-point of a chord to the centre of the circle passes through the mid-point of the corresponding minor arc.
View Solutionprove that the line joining the mid-point of two equal chords of a circle subtends equal angles with the chord.
View SolutionProve that the line segment joining the points of contact of two parallel tangents passes through the centre.
View SolutionThe line joining the mid-points of two chords of a circle passes through its centre. Prove that the chords are parallel.
View SolutionIf a line segment joining mid-points of two chords of a circle passes through the centre of the circle, prove that the two chords are parallel.
View SolutionIf a line segment joining mid-points of two chords of a circle passes through the centre of the circle, prove that the two chords are parallel.
View SolutionProve that the line joining the mid-points of the two sides of a triangle is parallel to the third side.
View SolutionProve that the perpendicular at the point of contact to the tangent to a circle passes through the centre.
View SolutionProve that the perpendicular at the point of contact to the tangent to a circle passes through the centre.
View SolutionUsing Theorem 6.2, prove that the line joining the mid-point of any two sides of a triangle is parallel to the third side. (Recall that you have done it in class IX).
View Solution
