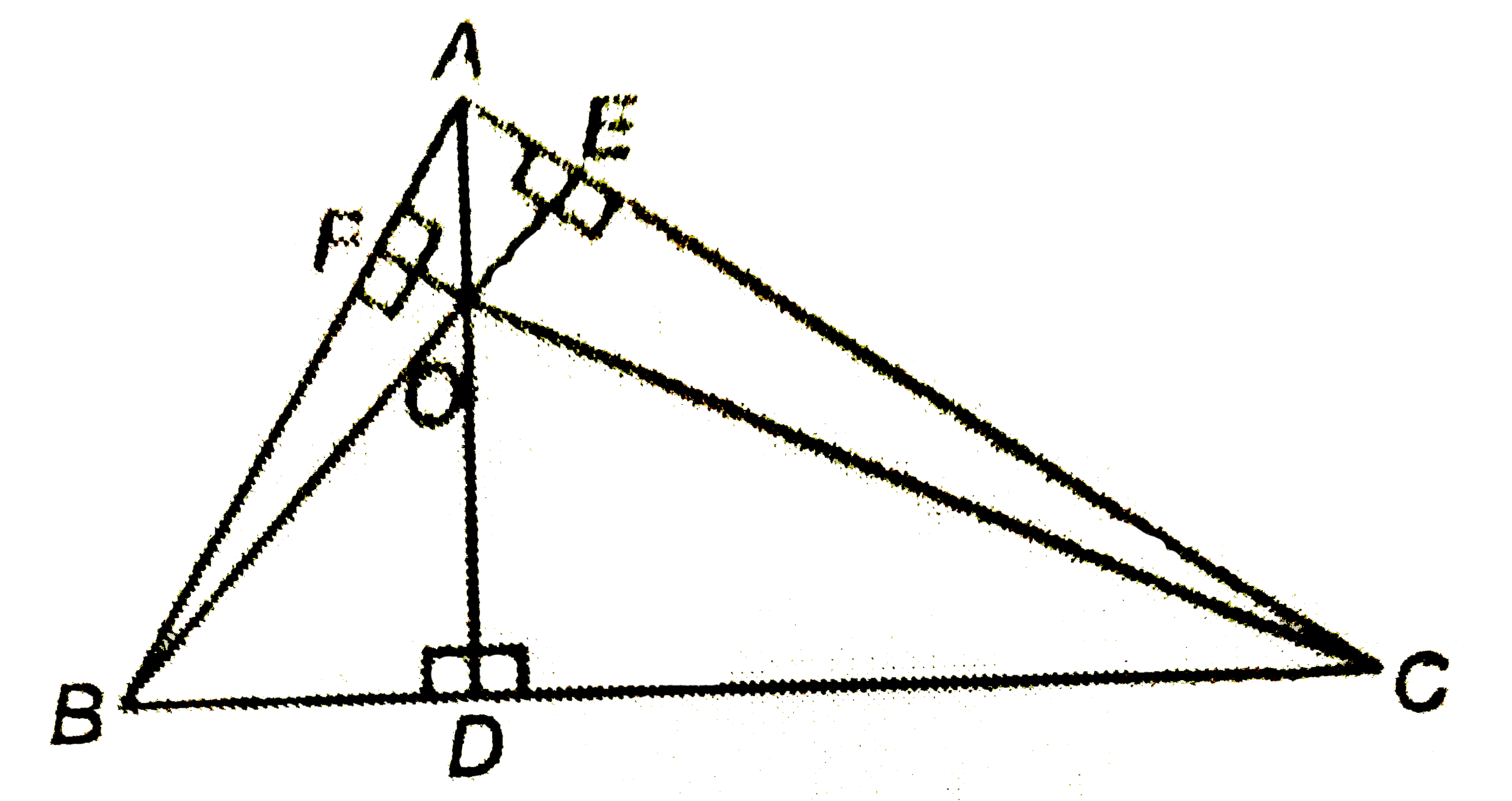
Prove that the perimeter of a triangle is greater than the sum of its altitudes.
Given : ABC is a triangle in which AD, BE and CF are perpendiculars.
To Prove : AB+BC+CA>AD+BE+CF
Proof : Since in a right angled triangle, hypotenuse is the longest side.
∴ In right ΔABD, AB>AD ...(1)
(side opposite to greater angle is longer)
In right ΔBEC, BC>BE ...(2)
(side opposite to greater angle is longer)
In right ΔAFC, AC>CF (side opposite to greater angle is longer) ...(3)
Adding (1), (2) and (3), we get
AB+BC+CA>AD+BE+CF Hence Proved.
Topper's Solved these Questions
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Problems From NCERT/exemplar
5 Videos
- TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLESEXERCISE - Exercise 7a
35 Videos
- SURFACE AREA AND VOLUMEBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SURFACE AREA AND VOLUMEEXERCISE - Revision Exercise (long Answer Questions)
10 Videos
Similar Questions
Prove that the perimeter of a triangle is greater than the sum of its three medians.
View SolutionProve that the perimeter of a triangle is greater than the sum of the three medians. GIVEN : A ABC in which AD,BE and CF are its medians. TO PROVE : AB+BC+AC>AD+BE+CF
View SolutionProve that the perimeter of a right triangle is equal to the sum of the diameter of its incircle and twice the diameter of its circumcircle.
View SolutionWhich of the following statements are true (T) and which are false (F)?
(i) Sum of the three sides of a triangle is less than the sum of its three altitudes.
(ii) Sum of any two sides of a triangle is greater than twice the median drawn to the third side.
(iii) Sum of any two sides of a triangle is greater than the third side.
(iv) Difference of any two sides of a triangle is equal to the third side.
(v) If two angles of a triangle are unequal, then the greater angle has the larger side opposite to it.
(vi) Of all the line segments that can be drawn from a point to a line not containing it, the perpendicular line segment is the shortest one.
View SolutionTwo equal sides of a triangle are each 5 metres less than twice the third side. If the perimeter of the triangle is 55 meters, find the lengths of its sides.
View SolutionIf the perimeter of a triangle is 6, then its maximum area is
View SolutionProve that the altitudes of a triangle are concurrent.
View SolutionProve that in a quadrilateral the sum of all the sides is greater than the sum of its diagonals.
View SolutionProve that in a quadrilateral the sum of all the sides is greater than the sum of its diagonals.
View SolutionProve that sum of any two sides of a triangle is greater than twice the median with respect to the third side.
View Solution
