In the adjoining figure, the diagonals AC and BD of a quadrilateral ABCD intersect point O. Prove that :
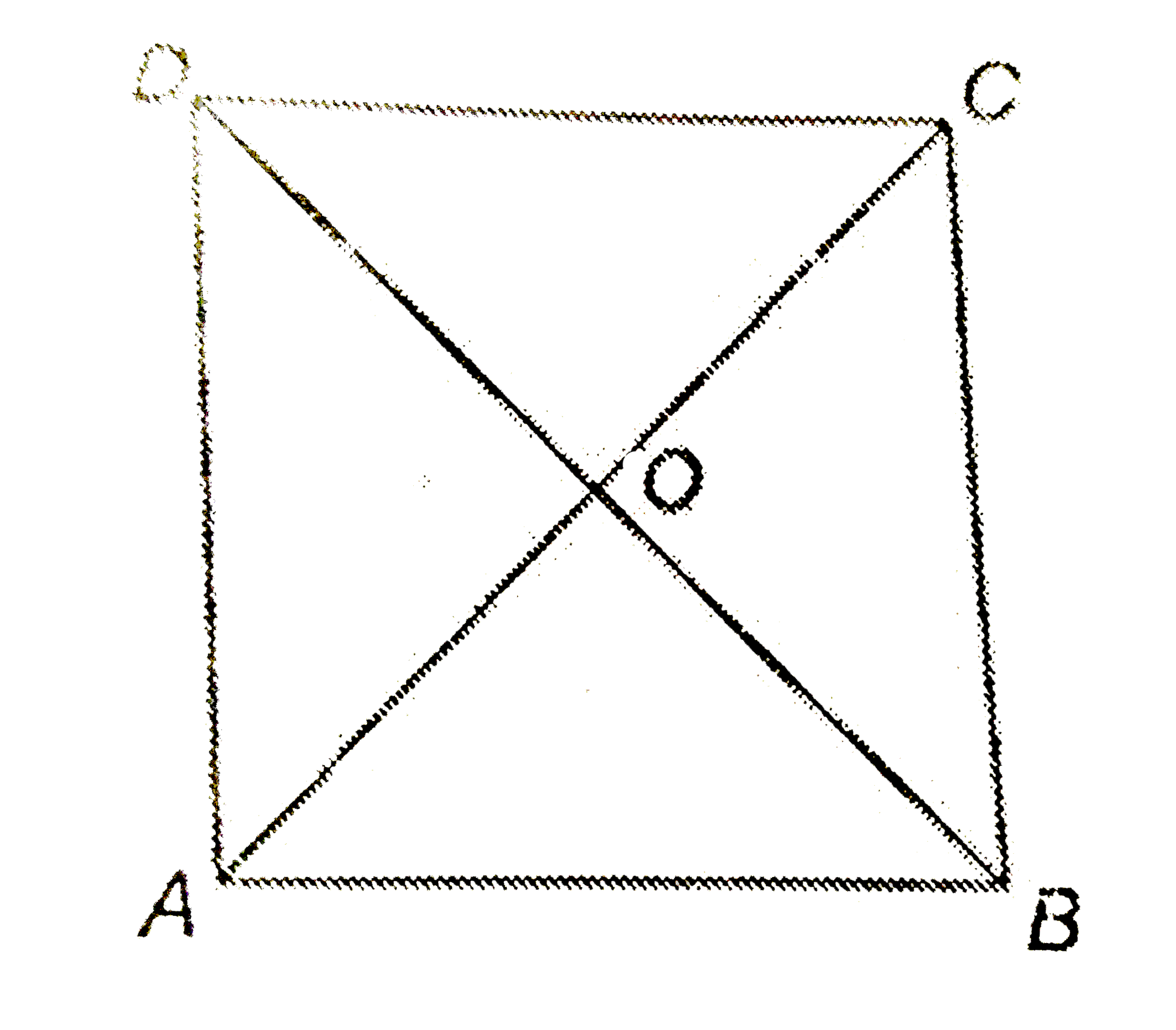
Step by step video & image solution for In the adjoining figure, the diagonals AC and BD of a quadrilateral ABCD intersect point O. Prove that : AB+BC+CD+DA lt 2(AC+BD) by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In the adjoining figure, ABCD is a quadrilateral. Its diagonals AC and BD intersect at point 'O'. Prove that :
(a) AB+BC+CD+DA<2(AC+BD)
(b) AB+BC+CD+DA>(AC+BD)

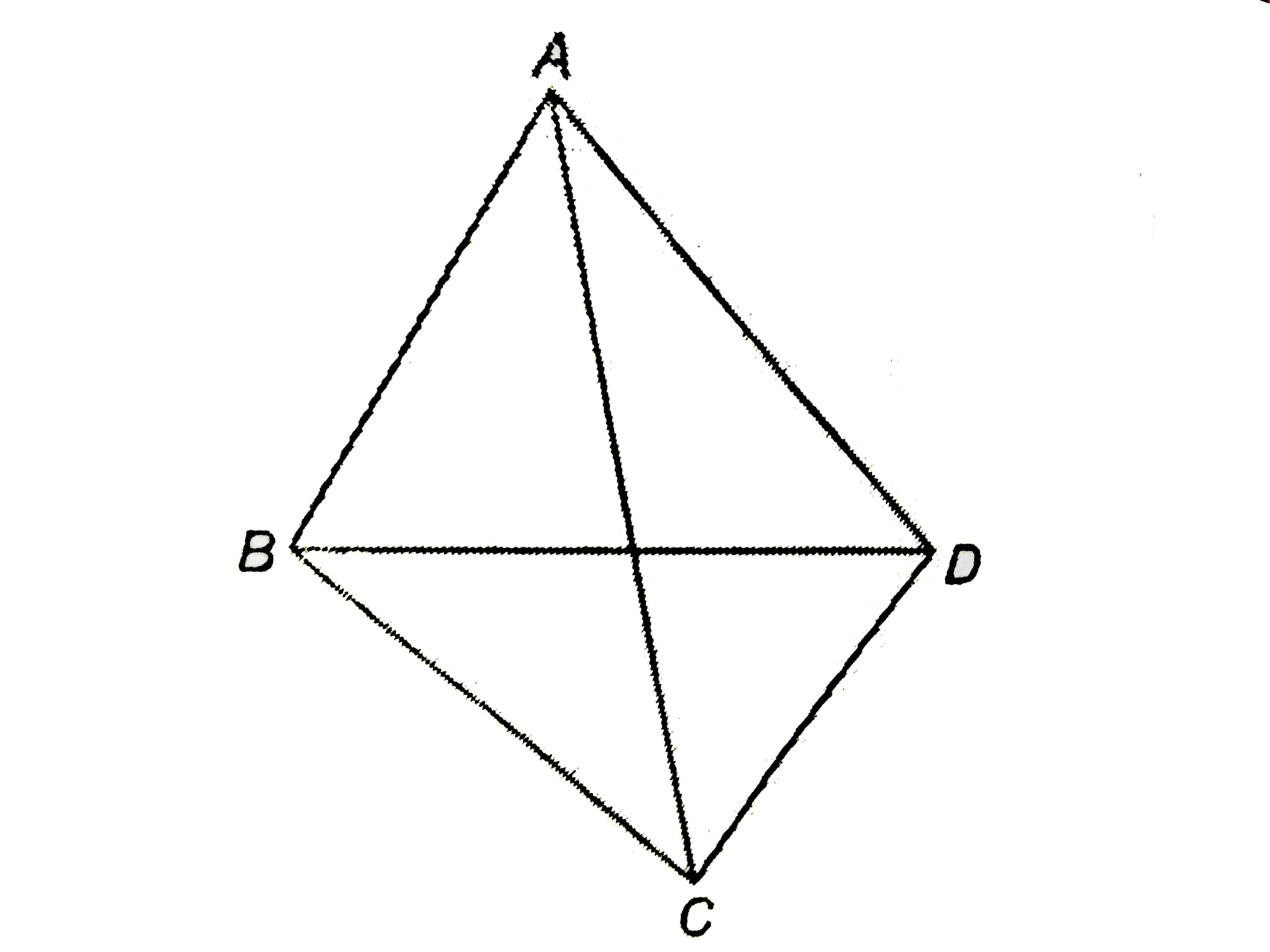
View SolutionThe given figure shows a quadrilateral ABCD. Prove that :
AB+BC+CD+DA>AC+BD
View SolutionIf the diagonal BD of a quadrillateral ABCD bisects both ∠Band∠D. Prove that ABBC=ADCD.
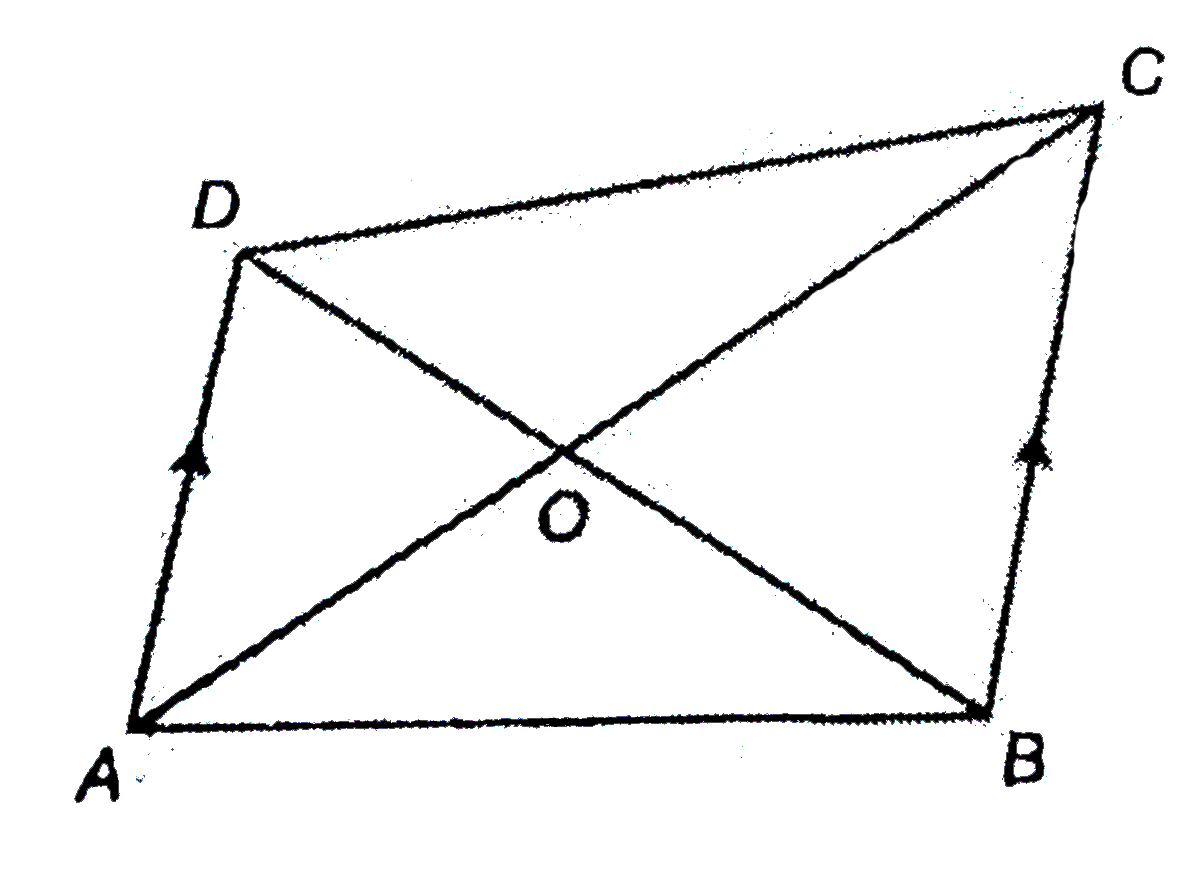
View SolutionIn the adjoining figure, ABCD is a quadrilateral in which AD∣∣BC. AC and BD intersect each other at point 'O'. Prove that:
area of ΔCOD= area of ΔABO
View SolutionIn the following figure AC=10&BD=14, then area of quadrilateral ABCD-
View Solution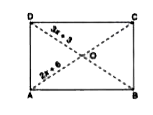
In the given figure, the diagonals AC and BD of a rectangle ABCD intersect each other at point O.
If OA=2x+6 and OD=3x+3, find the value of x.
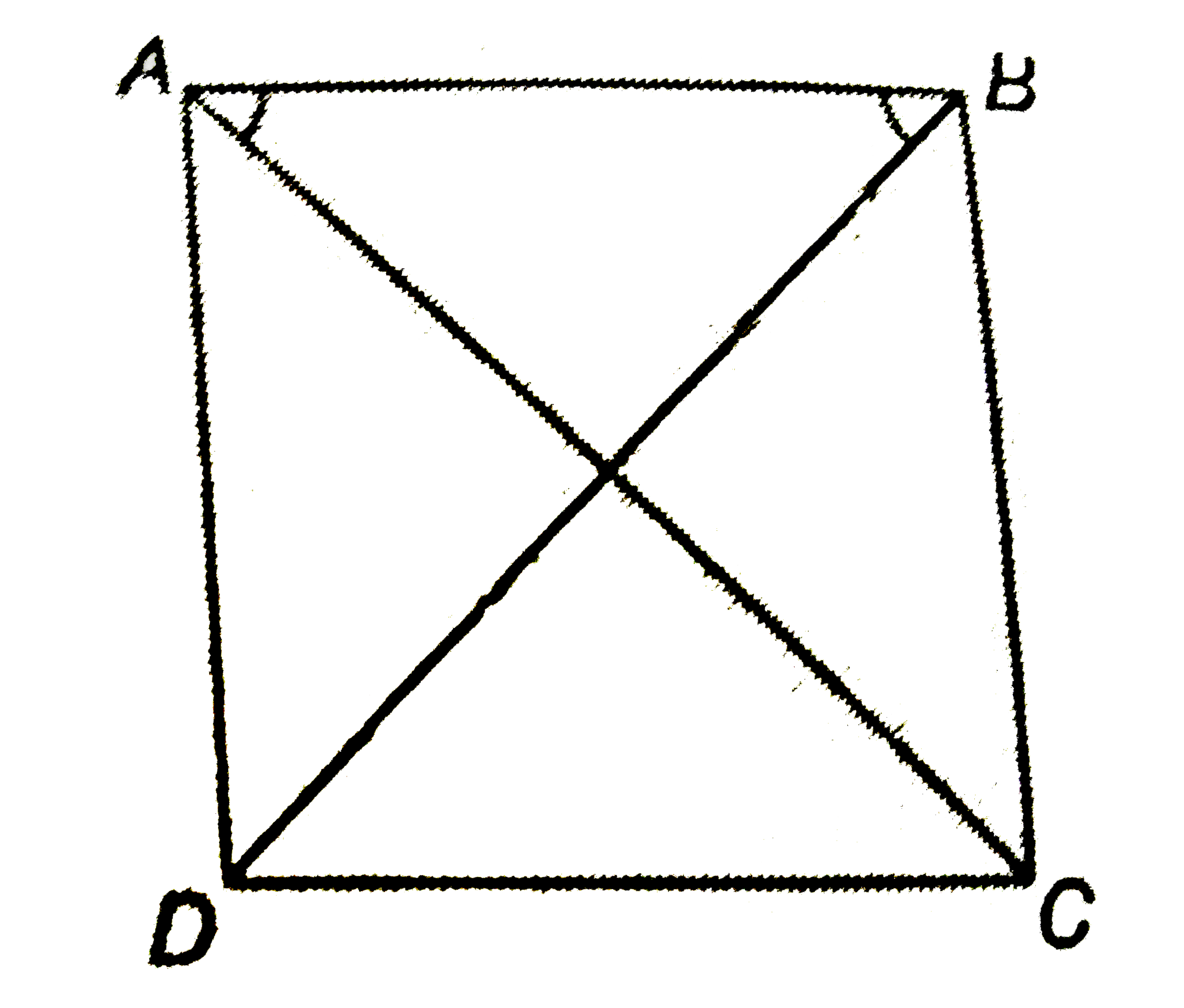
View SolutionIn theadjoining figure, ∠DAB=∠ABC and AD=BC prove that BD=AC.
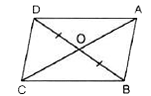
View SolutionIn the given figure, the diagonals AC and BD intersects at point O. If OB=OD and AB//DC, prove that :
(i) Area(ΔDOC)=Area(ΔAOB).
(ii) Area(ΔDCB)=Area(ΔACB).
(iii) ABCD is a parallelogram.
View SolutionABCD is quadrilateral. Is AB+BC+CD+DA<2(AC+BD)?
View SolutionP, Q , R and S are respectively the mid-points of the sides AB, BC, CD and DA of a quadrilateral ABCD in which AC = BD. Prove that PQRS is a rhombus.
View Solution
