Prove that the point (x,√1−x2) is at a distance of 1 unit from the origin .
The correct Answer is:Prove by using area of triangle=0 if points are collinear.
To prove that the point (x,√1−x2) is at a distance of 1 unit from the origin (0,0), we will use the distance formula between two points in a Cartesian coordinate system.
1. Identify the Points:
- The point we are considering is A(x,√1−x2).
- The origin point is O(0,0).
2. Use the Distance Formula:
The distance d between two points A(x1,y1) and B(x2,y2) is given by the formula:
d=√(x1−x2)2+(y1−y2)2
In our case, A is (x,√1−x2) and O is (0,0).
3. Substitute the Points into the Formula:
d=√(x−0)2+(√1−x2−0)2
This simplifies to:
d=√x2+(√1−x2)2
4. Simplify the Expression:
The term (√1−x2)2 simplifies to 1−x2. Therefore, we have:
d=√x2+(1−x2)
5. Combine Like Terms:
d=√x2+1−x2=√1
6. Final Calculation:
d=1
Conclusion:
We have shown that the distance from the point (x,√1−x2) to the origin (0,0) is indeed 1 unit.
- The point we are considering is A(x,√1−x2).
- The origin point is O(0,0).
The distance d between two points A(x1,y1) and B(x2,y2) is given by the formula:
d=√(x1−x2)2+(y1−y2)2
In our case, A is (x,√1−x2) and O is (0,0).
d=√(x−0)2+(√1−x2−0)2
This simplifies to:
d=√x2+(√1−x2)2
The term (√1−x2)2 simplifies to 1−x2. Therefore, we have:
d=√x2+(1−x2)
d=√x2+1−x2=√1
d=1
We have shown that the distance from the point (x,√1−x2) to the origin (0,0) is indeed 1 unit.
Topper's Solved these Questions
- Co-ordinate GeometryBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - Co-ordinate GeometryEXERCISE - PRACTICE-TEST (Coordinate Geometry) SECTION-A
4 Videos
- Co-ordinate GeometryBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - Co-ordinate GeometryEXERCISE - PRACTICE-TEST (Coordinate Geometry) SECTION-B
3 Videos
- Co-ordinate GeometryBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - Co-ordinate GeometryEXERCISE - SHORT ANSWER TYPE QUESTION -II
10 Videos
- CIRCLESBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - CIRCLESEXERCISE - PRACTICE - TEST (SECTION - D)
1 Videos
- CONSTRUCTIONSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - CONSTRUCTIONSEXERCISE - (PRACTICE-TEST) SECTION-C
1 Videos
Similar Questions
Knowledge Check
What is the locus of the points P(x, y) (where xy > 0), which is at a distance of 2 units from the origin?
Ax2+y2=4Bx2+y2=4,x>0,g>0Cx2+y2=4,x<0,y<0DNone of theseAn equation of the plane through the points (1,0,0), (0,2,0) and at a distance 6/7 units from the origin, is
A6x+3y+z−6=0B6x+3y+2z−6=0C6x+3y+z+6=0D6x+3y+2z+6+0A is a point on the positive x-axis at a distance 3 units from the origin and B is a point on the positive y-axis at a distance 4 units from the origin. If P divides AB in the ratio 1 : 2, the coordinates of P are
A(1, 8/3)B(2, 4/3)C(8/3, 1)D(4/3, 2)
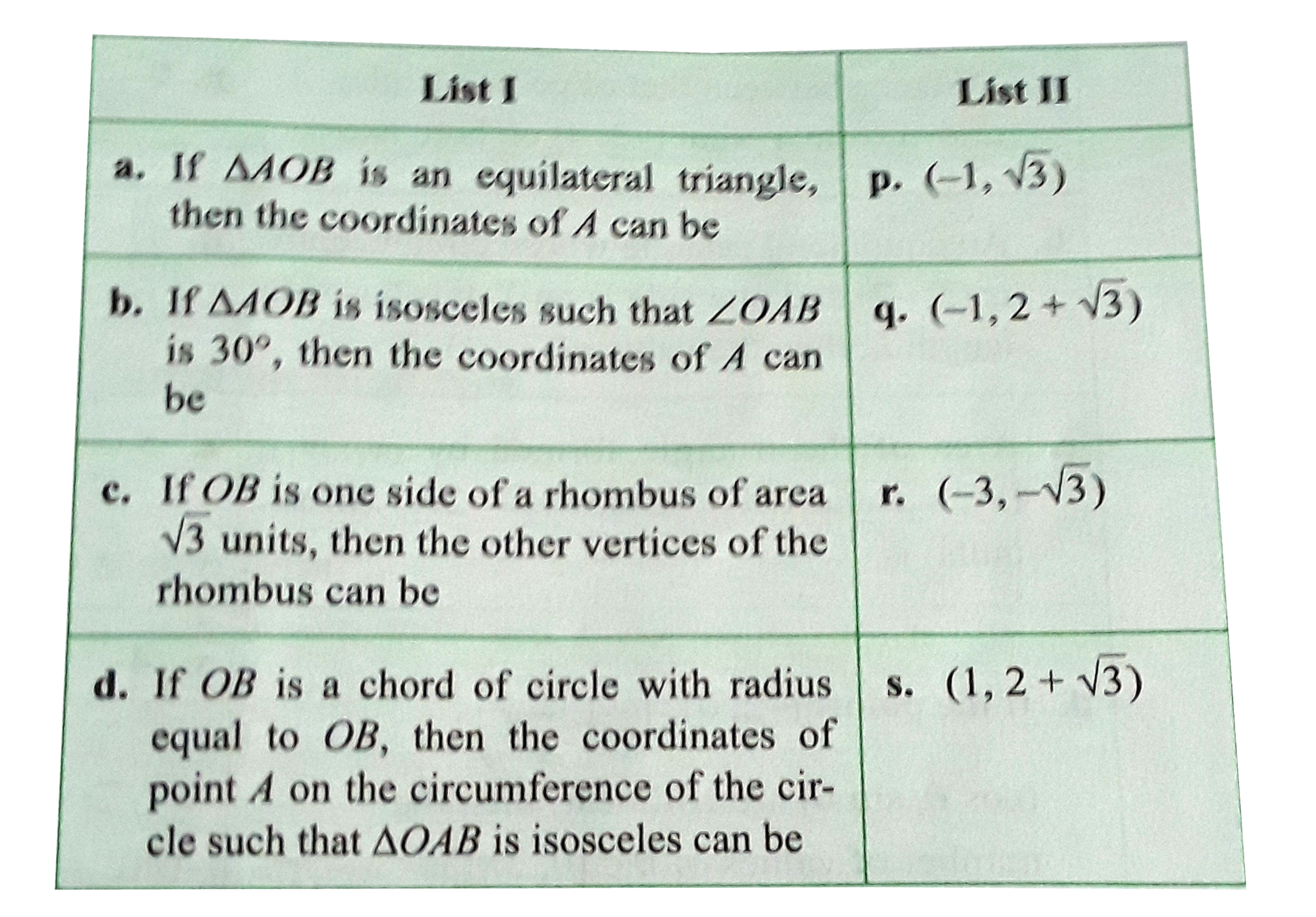
O is the origin and B is a point on the x-axis at a distance of 2 units from the origin. Match the following lists.
View SolutionProve thatsin−1x=cos−1√1−x2
View SolutionFind the equation of a circle:
1)Which touches both the axes at a distance of 6 units from the origin.
2)Which touches x-axis at a distance 5 from the origin and radius 6 units
3)Which touches both the axes and passes through the point (2,1)
4)Passing through the origin, radius 17 and ordinate of the centre is -15.
View SolutionFind the point on y-axis which is at a distance of √10 units from the point(1,2,3).
View SolutionFind the equation of a line parallel to the line x+2y-1=0, which is at a distance of 2√5 units from the point (1,3) .
View Solution
