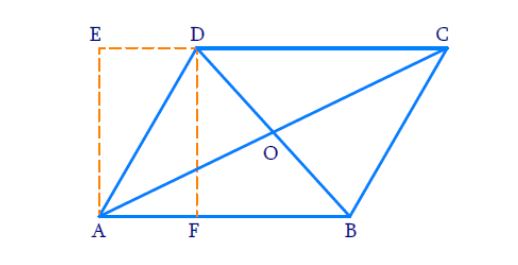
Prove that the sum of the squares of the diagonals of a parallelogram is equal to the sum of the squares of its sides.
Let, ABCD is a parallelograma
AB=CD, AD=BC [Since opposite sides of a parallelogram are equal]
AC and BD are the diagonals of the parallelogram ABCD
Draw AE⊥CD, DF⊥AB
We have to prove that,AC2+BD2=AB2+BC2+CD2+AD2
EA=DF(Perpendiculars drawn between same parallel lines)
According to Pythagoras theorem,
In ΔAEC
AC2=AE2+EC2
AC2=AE2+(ED+DC)2[Since EC = ED + DC]
AC2=AE2+DE2+DC2+2DE.DC [Since (a+b)2=a2+b2+2ab]
AC2=AD2+DC2+2DE.DC .....(i) [Since, AD2=AE2+DE2 in ΔAED]
In ΔDFB
BD2=DF2+BF2
BD2=DF2+(AB−AF)2[Since BF=AB=AF]
BD2=DF2+AB2+AF2−2AB.AF [Since (a−b)2=a2+b2−2ab]
BD2=AD2+AB2−2AB.AF .....(ii) [Since, AD2=DF2+AF2 in ΔAFD]
Adding (i) and (ii)
AC2+BD2=AD2+DC2+2DE.DC+AD2+AB2−2AB.AF
AC2+BD2=BC2+DC2+2AB.AF+AD2+AB2−2AB.AF [Since AD=BC and DE=AF, CD=AB]
Thus, AC2+BD2=AB2+BC2+CD2+AD2
Topper's Solved these Questions
Similar Questions
prove by vector method that the sum of the squares of the diagonals of a parallelogram is equal to the sum of the squares of its sides.
View SolutionProve that the sum of the squares of the diagonals of parallelogram is equal to the sum of the squares of its sides.
View SolutionProve by vector method that the sum of the square of the diagonals of a parallelogram is equal to the sum of the squares of its sides.
View SolutionProve analytically that the sum of square of the diagonals of a rectangle is equal to the sum of squares of its sides.
View SolutionProve that the sum of the squares of the sides of a rhombus is equal to the sum of the squares of its diagonals.
View SolutionProve that the sum of the squares of the sides of a rhombus is equal to the sum of the squares of its diagonals.
View SolutionProve that three times the sum of the squares of the sides of a triangle is equal to four times the sum of the squares of the medians of the triangle.
View SolutionThe square of the diagonal of a square is 50 sq. units. Find the side of the square.
View SolutionProve that the sum of two consecutive angles of a parallelogram is 180∘.
View SolutionProve that in a parallelogram, the sum of the squres of the diagonals is equal otthe four times the sum of the square of three conterminos edges.
View Solution
