Prove using similar triangles, that a line drawn through the mid-point of one side of a triangle parallel to another side, bisects the third side.
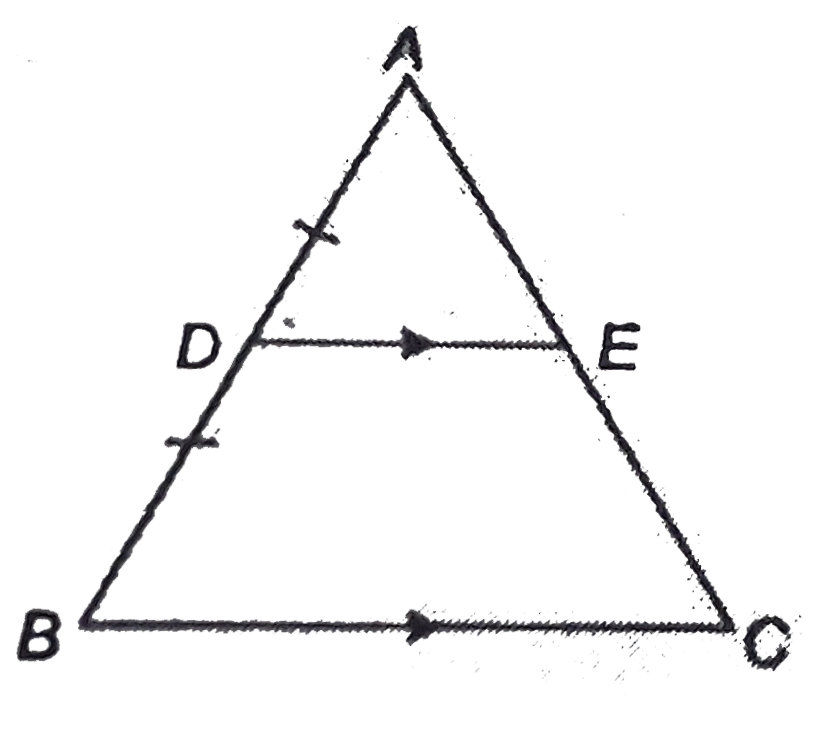
Given : ΔABC in which D is the mid-point of AB and DE is drawn parallel to BC, meeting AC in E.
To prove : AE= EC
proof: Since DE||BC
By basic proportionality theorem, we have
ADDB=AEEC…..(1)
but AD=DB (D is the mid - point )
ADDB=ADAD=1
from (1) and (2),we have
AEEC=1
⇒AE=EC
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Problems From NCERT/ Exemplar14videos
TRIANGLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Exercise 6a24videos
TRIANGLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions4videos
STATISTICS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions5videos
VOLUME AND SURFACE AREA OF SOLIDS
Similar Questions
The line drawn through the mid-point of one side of a triangle, parallel to another side, intersects the third side at its mid-point.
View SolutionUsing Theorem 6.1, prove that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side. (Recall that you have proved it in Class IX).
View SolutionProve that the line joining the mid-points of the two sides of a triangle is parallel to the third side.
View SolutionProve that the line segment joining the mid points of two side of a triangle is parallel to the third side and equal to half of it.
View SolutionProve that the line segment joining the mid points of two side of a triangle is parallel to the third side and equal to half of it.
View SolutionThe line segment joining the mid-points of any two sides of a triangle in parallel to the third side and equal to half of it.
View SolutionProve analytically that the line segment joining the middle points of two sides of a triangle is equal to half of the third side.
View SolutionUsing Theorem 6.2, prove that the line joining the mid-point of any two sides of a triangle is parallel to the third side. (Recall that you have done it in class IX).
View SolutionShow that the difference of any two sides of a triangle is less than the third side.
View SolutionProve that if a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, then the other two sides are divided in the same ratio.
View Solution

