Show that the function f(x) defined by .f(x)=17, for 1≤x≤8 =0, otherwise, is a probability density function for a random variable. Hence find P(3<X<10)
The correct Answer is:57
Step by step video, text & image solution for Show that the function f(x) defined by .f(x) = (1)/(7), for 1 le x le 8 =0, otherwise, is a probability density function for a random variable. Hence find P(3 lt Xlt 10) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROBABILITY DISTRIBUTIONBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - MULTIPLE CHOICE QUESTIONS
9 Videos
- PROBABILITY DISTRIBUTIONBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - MULTIPLE CHOICE QUESTIONS
9 Videos
- PLANE BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PLANE EXERCISE - MULTIPLE CHOICE QUESTIONS
8 Videos
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - BINOMIAL DISTRIBUTION
20 Videos
Similar Questions
Find k so that the function f(x) defined by
f(x)=ke−3x,x>0
= 0 , otherwise.
is a probability density function.
View SolutionFind k if the function f(x) is defined by
f(x)=kx(1−x),for 0<x<1
=0 , otherwise, is the probability density function (p.d.f.) of a random varible (r.v) X. Also find P (X<12)
View Solution
Knowledge Check
Consider the greatest integer function, defined by f(x)=[x],0≤x<2. Then,
Af is derivable at x = 1Bf is not derivable at x = 1Cf is derivable at x = 1DNone of the aboveIf the probability density function of a random variable X is f(x)=x2 in 0≤x≤2, then P(X>1.5∣X>1) is equal to
A716B34C712D2164If the probability density function of a random variable X is f(x)=π2 in 0≤x≤2, then P(X>1.5X>1) is equal to
A716B34C712D2164
If f(x)= kx, 0 lt x lt 2 = 0 otherwise, is a probability density function of a random variable X , then find (i) value of k, (ii) P(1 lt x lt2).
View SolutionIf the probability density function of a random variable X is given as
then F(0) is equal to
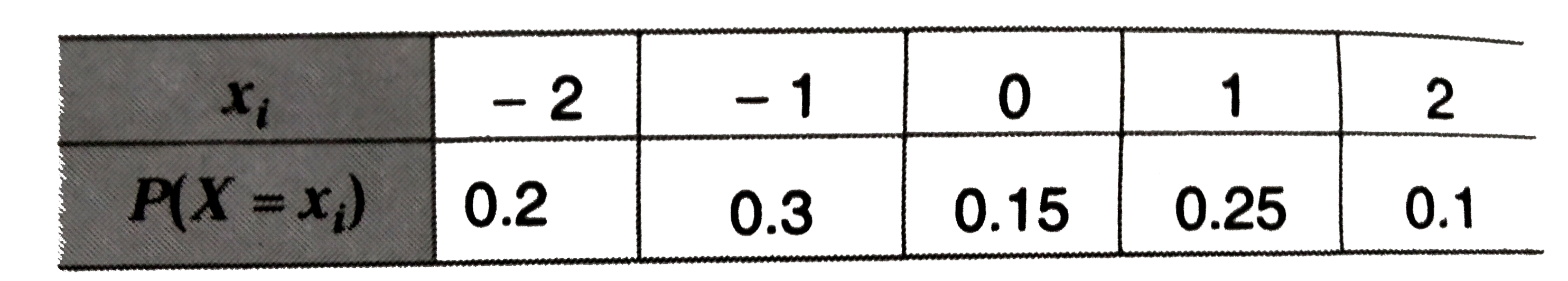
View SolutionFind k, if the function f defined by f(x)=kx,0<x<2 =0 , otherwise is the p.d.f of a random variable X.
View SolutionThe following is the p.d.f of continuous random variable X. f(x)=x8,0<x<4
=0 , otherwise.
Find the expression for c.d.f of X
View SolutionA function is defined as f(x)={ex,x≤0|x−1|,x>0, then f(x) is
View Solution
