Show that the points A(a,b),B(a+α+b,+β),C(a+α+p,b+β+q)andD(a+p,b+q) when joined in order, form a parallelogram. Find the cooditions for which the paralleogram is a(i)rectangle (ii) rhombus.Solution in Bengali
The correct Answer is:(i)aα+βq=0
(ii) α2+β2=p2+q2
Step by step video, text & image solution for Show that the points A(a,b), B(a+alpha+ b,+ beta), C(a+alpha+ p, b+beta+ q) and D(a+p,b+q) when joined in order, form a parallelogram. Find the cooditions for which the paralleogram is a(i)rectangle (ii) rhombus. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - CHHAYA PUBLICATIONCHAPTER - MISCELLANEOUS EXAMPLESEXERCISE - COORDINATE GEOMETRY (THREE DIMENSIONAL COORDINATE GEOMETRY)10videos
MISCELLANEOUS EXAMPLES
- BOOK - CHHAYA PUBLICATIONCHAPTER - MISCELLANEOUS EXAMPLESEXERCISE - CALCULUS84videos
MISCELLANEOUS EXAMPLES
- BOOK - CHHAYA PUBLICATIONCHAPTER - MISCELLANEOUS EXAMPLESEXERCISE - ALGEBRA141videos
MISCELLANEOUS EXAMPLES
- BOOK - CHHAYA PUBLICATIONCHAPTER - METHOD OF SUBSTITUTIONEXERCISE - Sample Questions for Competitive Examination (Assertion-Reason Type)2videos
METHOD OF SUBSTITUTION
- BOOK - CHHAYA PUBLICATIONCHAPTER - ORDER AND DEGREE OF DIFFERENTIAL EQUATION EXERCISE - Sample Questions for Competitive Examination (E Assertion - Reasion Type )2videos
ORDER AND DEGREE OF DIFFERENTIAL EQUATION
Similar Questions
Determine the perpendicular distances of the points (-4,3,4) from the coordinates axes.
04:31View SolutionWithout using graph paper, draw the graph of the function f(x)=sin1x and determine from the graph whether limx→0f(x) exsits or not.
05:10View SolutionSolve the equation z2+|Z|=0 where z is a complex quantity.
02:09View SolutionStatement-I: ∫x+1x(1+xex)2dx=11+xex+log∣∣∣xex1+xex∣∣∣+c where c is a real number.
Statement-II: ddx(xex)=(x+1)ex.06:41View SolutionStatement - I : The differential equation of curves
represented by y=Aex is given by dydx=y
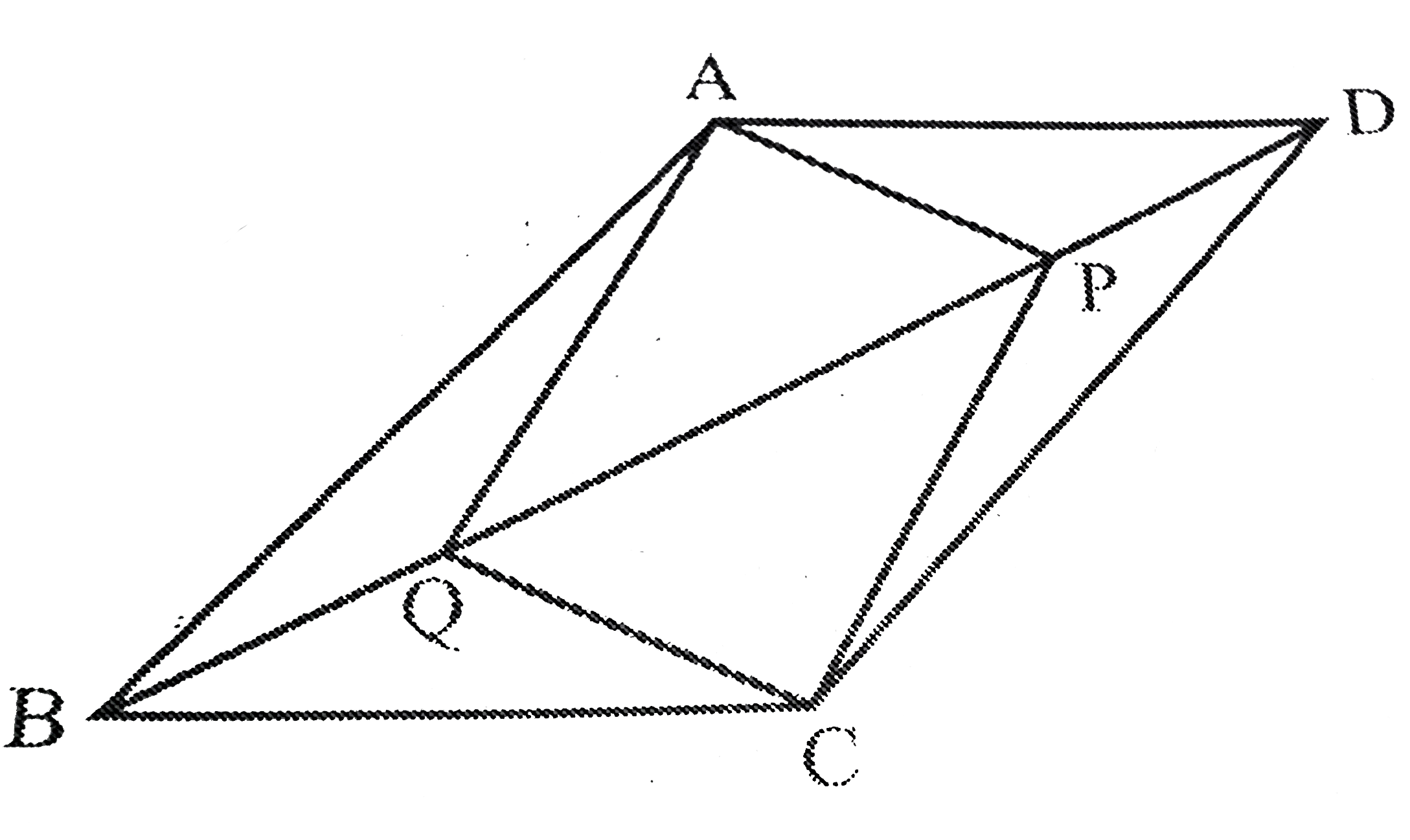
Statement-II: dydx=y is valid for every member of the given family08:17View SolutionIn parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP=BQ. Show that:
(i) ΔAPD≅ΔCQB
(ii) AP=CQ
(iii) ΔABC
(iv) AQ=CP
(v) APCQ is a parallelogram.
 05:14View Solution
05:14View SolutionIf alpha and beta are the roots of the equation x^(2)-p(x+1)-q=0 then the value of (alpha^(2)+2 alpha+1)/(alpha^(2)+2 alpha+q)+(beta^(2)+2 beta+1)/(beta^(2)+2 beta+q) is (A)1(B)2 (C) 3(D)0
02:01View SolutionIf α,β are the roots of ax2+bx+c=0andα+h,β+h are the roots of px2+qx+r=0thenh= −12(ab−pq) b. (ba−qp) c. 12(bq−qp) d. none of these
05:30View SolutionThe circle x^(2)+y^(2)=4 cuts the line joining the points A(1,0) and B(3,4) in two points P and Q. Let B(P)/(P)A=alpha and B(Q)/(Q)A=beta. Then alpha and beta are roots of the quadratic equation
01:58View SolutionIf (a+ib)^(5)+alpha+i beta then (b+ia)^(5) is equal to ( A) beta-i alpha(B)beta+i alpha(C)alpha-i beta(D)-alpha-i beta
02:00View SolutionIf alpha and beta are the zeroes of the quadratic polynomial f(x)=ax^(2)+bx+c then evaluate,(i) (1)/(a alpha+b)+(1)/(a beta+b) (ii) (beta)/(a alpha+b)+(alpha)/(a beta+b)
06:00View SolutionIf A=[abba]andA2=[αββα] then (A) α=a2+b2,β=ab (B) α=a2+b2,β=2ab (C) α=a2+b2,β=a2−b2 (D) α=2ab,β=a2+b2
05:44View SolutionIfasinα=bsinβ then show that ,bcotα+acotβ=(a+b)cotα+β2
02:43View SolutionIf alpha and beta are the roots of the equationx2−ax+b=0,find
Q(a)αβ+βα07:26View SolutionIf alpha and beta are the roots of the equationx2−ax+b=0,find
Q(b)1α+1β08:48View Solution

