Show that the quadrilateral formed by joining the mid-points of the consecutive sides of a rectangle is a rhombus.

Video Solution

Text Solution

Verified by Experts

Solution
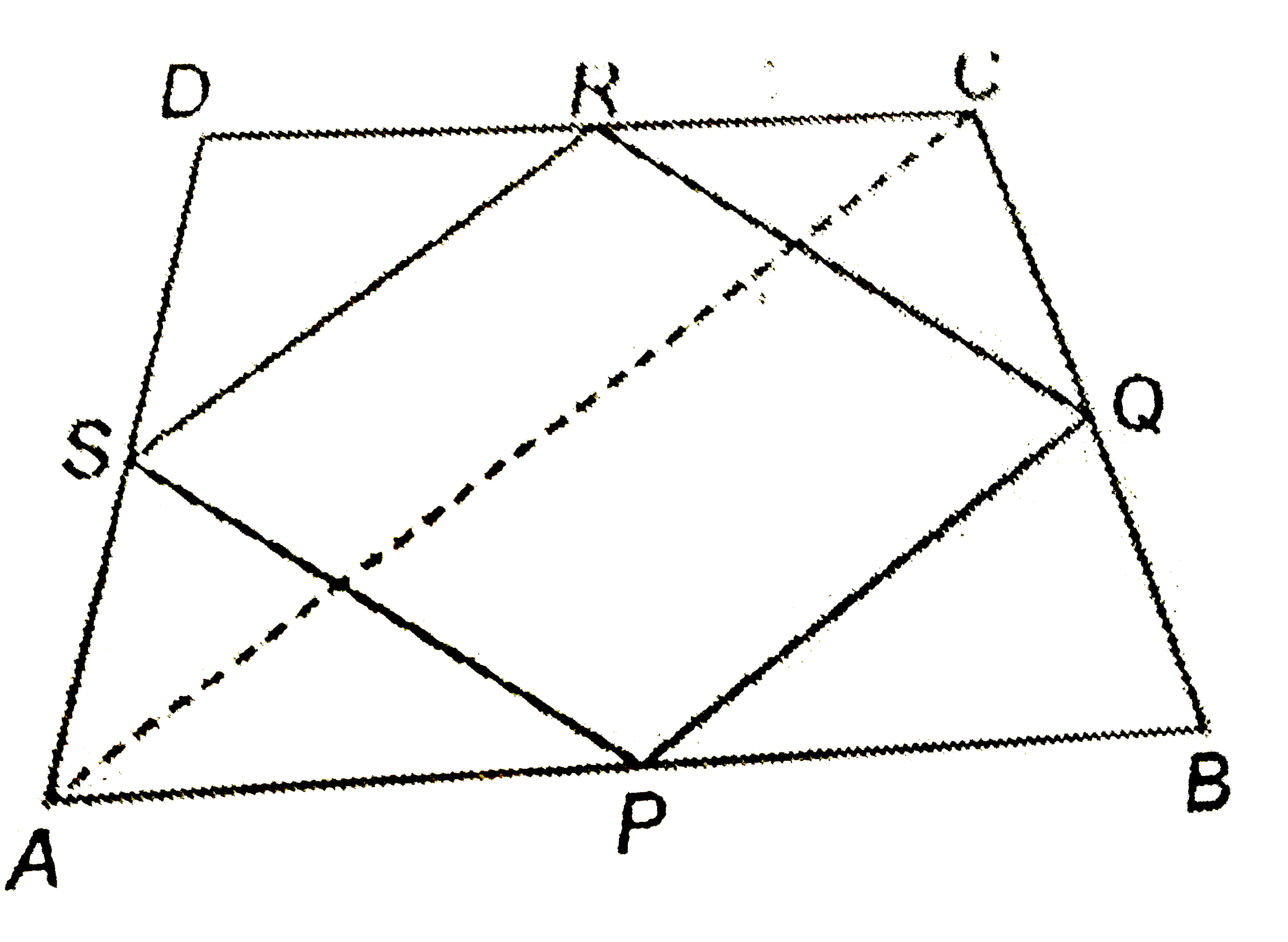
A rectangle $A B C D$ in which $P, Q, R$ and $S$ are the mid-points of sides $A B, B C, C D$ and $D A$ respectively.
$P Q, Q R, R S$ and $S P$ are joined
Join $A C$ . In $△ A B C, P$ and $Q$ are the mid-points of sides $A B$ and $B C$ = respectively.
∴ $P Q ∣ ∣ A C$ and $P Q = \frac{1}{2} A C$ .....(i) (Mid pt. Theorem)
In $' △ A D C, R'$ and ' $S$ ' are the mid-points of sides $C D$ and $A D$ respectively.
∴ $' S R ∣ ∣ A C'$ and $' S R$ = $\frac{1}{2}$
$A C$ ....(ii) (Mid. pt. Theorem)
From (i) and (ii), we have $P Q ∣ ∣ S R$ and $' P Q = S R'$
⇒ $' P Q R S'$ is a parallelogram.
Now $' A B C D'$ is a rectangle.
∴ $A D = B C$
⇒ $\frac{1}{2} A D = \frac{1}{2} B C$
⇒ $A S = B Q$ .....(iii)'
In $△ S, A P S$ and $' B P Q'$ , we have
$A P = B P$ ( $P$ is the mid-point of $A B$ )
$∠ P A S = ∠ P B Q$ (Each is equal to $90^{\circ}$ )
and $A S = B Q$ (From (iii))
∴ $△ A P Q ≅ △ B P Q$ (SAS)
⇒ $P S = P Q$
∴ $P Q R S$ is a parallelogram whose adjacent sides are equal.
⇒ $P Q R S$ is a rhombus.

|

Updated on:21/07/2023

Class 9 MATHS QUADRILATERALS

Topper's Solved these Questions

Similar Questions

RD SHARMA-QUADRILATERALS-All Questions

Home
Ask Doubt
Profile