Question

Show that the reflection of the line $ax+by+c=0$
on the line $x+y+1=0$
is the line $bx+ay+(a+b-c)=0$
where $a\ne b\stackrel{.}{}$

Explanation

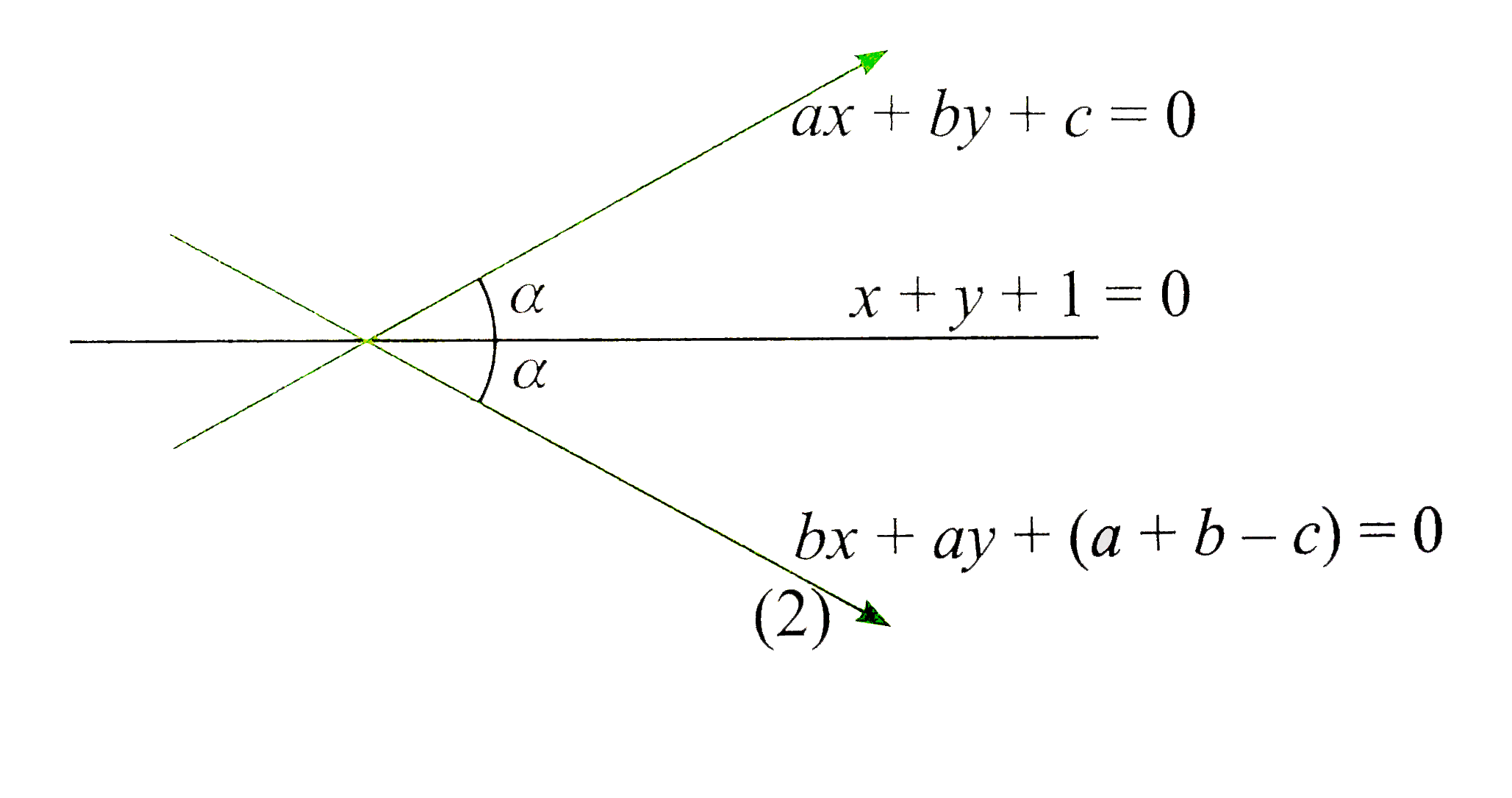
We have lines
`ax+by+c=0 " " (1)`
`x+y+1=0 " " (2)`
`bx+ay+(a+b-c) = 0 " " (3)`
Equations of angle bisectors of (1) and (3) are
`(|ax+by+c|)/(sqrt(a^(2)+b^(2))) = (|bx+ay+a+b-c|)/(sqrt(a^(2)+b^(2)))`
`"or " ax+by+c = +-(bx+ay+a+b-c)`
or x+y+1 =0 and (a-b)x+(b-a)y =a+b-2c
Since x+y+1= 0 is one of the bisectors of lines (1) and (3), reflection of line (1) in line (2) is line (3).