Sketch the region bounded by the curves $y = \sqrt{5 - x^{2}}$ and $y = | x - 1 |$ and find its area.

A

$\frac{5 π}{4} - 2$

B

$\frac{5 π - 2}{4}$

C

$\frac{5 π - 2}{2}$

D

$\frac{π}{2} - 5$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

The graphs of $y = | x - 1 | and y = \sqrt{5 - x^{2}}$ are showing in Fig. 15 and shaded region is the region bounded by th two curves.

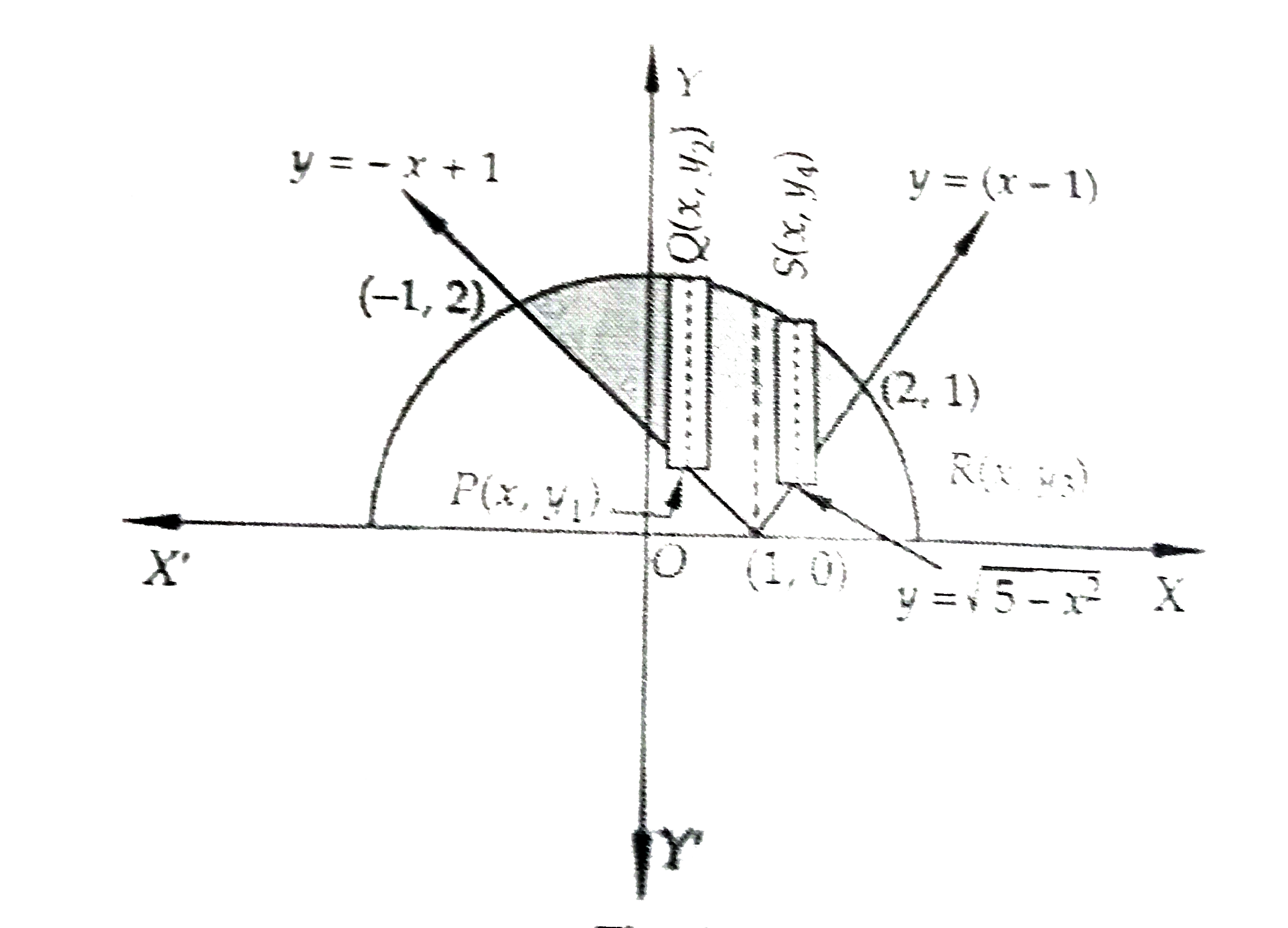
Let A be the area bonded by the given curves. Then.
$A = 1 \int - 1 (y_{2} - y_{1}) d x + 2 \int 1 (y_{4} - y_{3}) d x$
$\Rightarrow A = 1 \int - 1 (\sqrt{5 - x^{2}} + x - 1) d x + 2 \int 1 (\sqrt{5 - x^{2}} - x + 1) d x$
$\Rightarrow A 1 \int - 1 \sqrt{5 - x^{2}} d x + 2 \int 1 \sqrt{5 - x^{2}} d x + 1 \int - 1 (x - 1) d x + 2 \int 1 (- x + 1) d x$
$\Rightarrow A = 2 \int - 1 \sqrt{5 - x^{2}} d x + {[\frac{x^{2}}{2} - x]}_{- 1}^{1} + {[- \frac{x^{2}}{2} + x]}_{1}^{2}$
$\Rightarrow A = {[\frac{1}{2} x \sqrt{5 - x^{2}} + \frac{5}{2} {sin}^{- 1} \frac{x}{\sqrt{2}}]}_{- 1}^{2} - \frac{5}{2}$
$\Rightarrow A = 1 + \frac{5}{2} {sin}^{- 1} \frac{2}{\sqrt{5}} + 1 + \frac{5}{2} {sin}^{- 1} \frac{1}{\sqrt{5}} - \frac{5}{2}$
$\Rightarrow A = - \frac{1}{2} + \frac{5}{2} {sin}^{- 1} (\frac{2}{\sqrt{5}} \times \sqrt{1 - \frac{1}{5}} + \frac{1}{\sqrt{5}} \sqrt{1 - \frac{4}{5}})$
$A = - \frac{1}{2} + \frac{5}{2} {sin}^{- 1} (1) = \frac{5 π}{4} - \frac{1}{2} = \frac{5 π - 2}{4}$

|

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The area bounded by the curves $y = \sqrt{5 - x^{2}}$ and $y = | x - 1 |$ is
A $(\frac{5 π}{4} - 2)$ sq units
B $(\frac{5 π - 2}{4})$ sq units
C $(\frac{5 π - 2}{2})$ sq units
D $(\frac{π}{2} - 5)$ sq units
Question 2 - Select One
The area of the region bounded by the curves $x = y^{2} - 2$ and x=y is
A $\frac{9}{4}$
B9
C $\frac{9}{2}$
D $\frac{9}{7}$
Question 3 - Select One
The area of the region bounded by the curves $y = x^{2} and x = y^{2}$ is
A $\frac{1}{3}$
B $\frac{1}{2}$
C $\frac{1}{4}$
D3

OBJECTIVE RD SHARMA-AREAS OF BOUNDED REGIONS-Chapter Test

Home
Ask Doubt
Profile