Solve the inequalities and represent the solution graphically on number line. 5x+1>−24,5x−1<24.
Video Solution
Text Solution
Given,
5x+1>−24
⇒5x>−25
⇒x>−5 ....(1)
⇒5x−1<24
⇒5x<25
⇒x<5 ....(2)
From (1) and (2), we get, −5<x<5
Hence, it can be concluded that the solution set for the given system of inequalities is (−5,5)
The solution of the given system of inequalities can be represented on the number line as
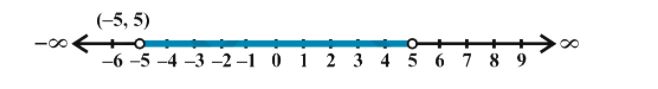
|
Topper's Solved these Questions
- LINEAR INEQUALITIESBOOK - NCERTCHAPTER - LINEAR INEQUALITIESEXERCISE - EXERCISE 6.1
26 Videos
- LINEAR INEQUALITIESBOOK - NCERTCHAPTER - LINEAR INEQUALITIESEXERCISE - EXERCISE 6.3
15 Videos
- LIMITS AND DERIVATIVESBOOK - NCERTCHAPTER - LIMITS AND DERIVATIVESEXERCISE - EXERCISE 13.3
8 Videos
- MATHEMATICAL REASONINGBOOK - NCERTCHAPTER - MATHEMATICAL REASONINGEXERCISE - EXERCISE 14.3
4 Videos
Similar Questions
Knowledge Check
Solve each of the following inequations and represent the solution set on the number line.
2x−112−x−13≤3x+14, where x∈R.A(0,∞)B(−∞,0)CRDNone of these
Solve the inequalities and represent the solution graphically on number line. 5(2x−7)−3(2x+3)≤0,2x+19≤6x+47.
View SolutionSolve the inequalities for real x and represent solution on number line
x−3x−5>2
View SolutionSolve the inequalities for real x and represent solution on number line
x+3x−2>0ξnR
View SolutionSolve the inequalities for real x and represent solution on number line
2x+3x−3≤4
View Solutionsolve the inequation 2x+4x−1≥5 and represent this solution on the number line.
View SolutionSolve the inequalities and show the graph of the solution in each case on number line : 5x−3≥3x−5
View SolutionSolve each of the following inequations and represent the solution set on the number line.
5x−83≥4x−72 where x∈R.
View Solution
