Statement-1 P(A)∩P(B)=P(A∩B), where P(A) is power set of set A.
Statement-2 P(A)∪P(B)=P(A∪B).Solution in Punjabi
Statement-1 is true, Statement-2 is true, Statement-2 is a correct explanation for Statement-1
Statement-1 is true, Statement-2 is true, Statement-2 is not a correct explanation for Statement-1
Statement-1 is true, Statement-2 is false
Statement-1 is false, Statement-2 is true
The correct Answer is:C
Let x∈P(A∩B)
⇔x⊆(A∩B)
⇔x⊆Aandx⊆B
⇔x∈P(A)andx∈P(B)
⇔x∈P(A)∩P(B)
∴P(A∩B)⊆P(A)∩P(B)
and P(A)∩P(B)⊆P(A∩B)
Hence, P(A)∩P(B)=P(A∩B)
Now, consider sets A = {1}, B = {2} implies A∪B = {1, 2}
∴P(A)={ϕ,{1}},P(B)={ϕ,{2}}
and P(A∪B)={ϕ{1},{2},{1,2}≠P(A)∪P(B)}
Hence, Statement-1 is true and Statement-2 is false.
Topper's Solved these Questions
- SETS, RELATIONS AND FUNCTIONS BOOK - ARIHANT MATHSCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise (Subjective Type Questions)
15 Videos
- SETS, RELATIONS AND FUNCTIONS BOOK - ARIHANT MATHSCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
12 Videos
- SETS, RELATIONS AND FUNCTIONS BOOK - ARIHANT MATHSCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise (Matching Type Questions)
2 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHSCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
- THE STRAIGHT LINESBOOK - ARIHANT MATHSCHAPTER - THE STRAIGHT LINESEXERCISE - The Straight Lines Exercise 8 : (Questions Asked in Previous 13 years Exams)
1 Videos
Similar Questions
If A = {x : x is a natural number}, B = {x : x is an even natural number}, C = {x : x is an odd natural number} and D = {x : x is a prime number}, Find :
(i) A∩B
(ii) A∩C
(iii) A∩D
(iv) B∩C
(v) B∩D
(vi) C∩D.
View SolutionLet R={(3,3),(6,6),(9,9),(6,12),(3,9),(3,12),(12,12),(3,6)} is a relation on set A={3,6,9,12} then R is a) an equivalence relation b) reflexive and symmetric only c) reflexive and transitive only d) reflexive only
View SolutionThe functions defined have domain R
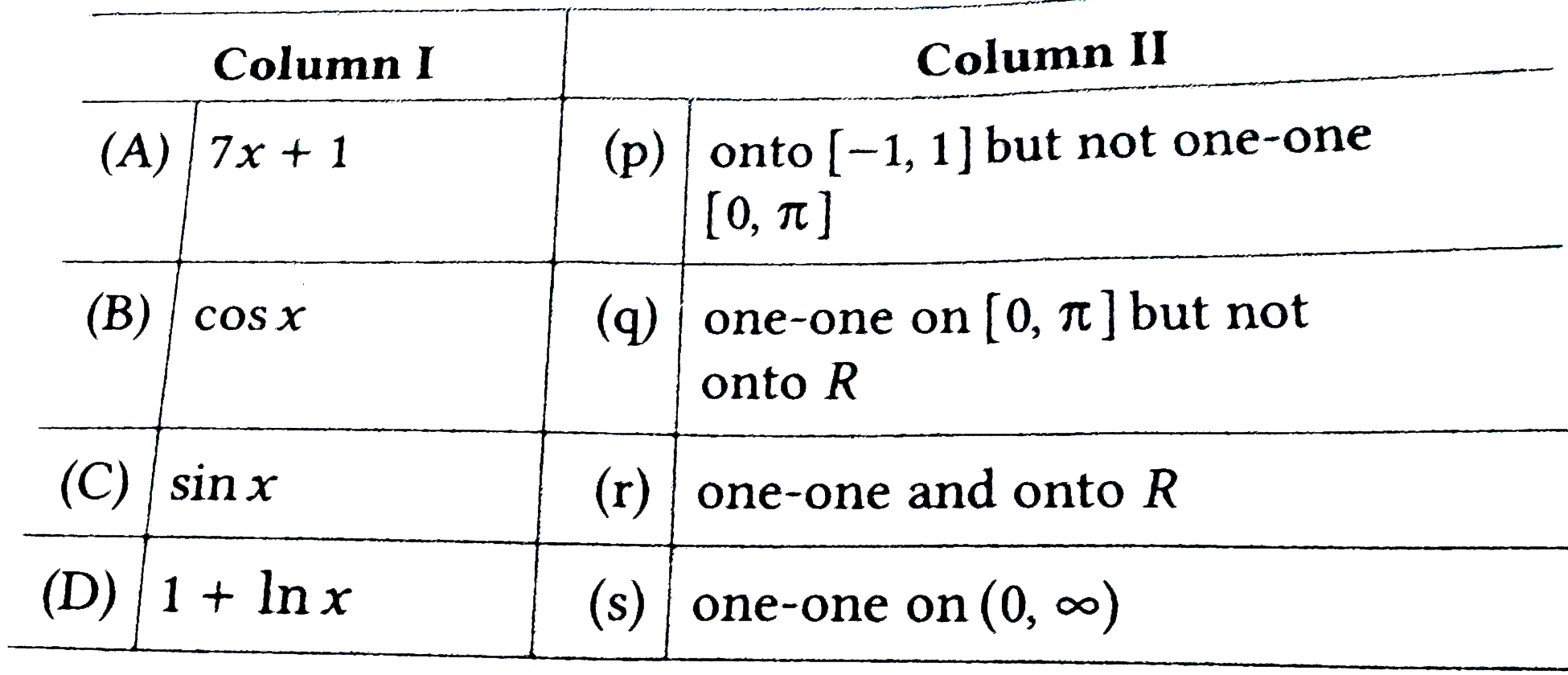
View SolutionLet a,b,c be in A.P. and |a|<1,|b|<1|c|<1.ifx=1+a+a2+....to ∞,y=1+b+b2+....to ∞and,z=1+c+c2+...to ∞, then x,y,z are in
View SolutionSolve the following system of inequalities graphically
x+5y≤25,2x+2y≥2,2x−2y≤0,x≥0,y≥0
View SolutionFor a set A, consider the following statements:
1.A∪P(A)=P(A) 2.{A}∩P(A)=A
3. P(A)−{A}=P(A)
where P denotes power set.
Which of the statements given above is/are correct?
View Solutionकिन्ही भी समुच्चयों A तथा B के लिए P(A)∪P(B)=P(A∪B) की सत्यता की जाँच कीजिए।
View Solutionसमुच्चय A और B के लिए क्या निम्नलिखित सत्य है?
P(A)∪P(B)=P(A∪B)
अपने उत्तर का औचित्य बताइए।
View Solutionयदि P(A)=13,P(B)=12 तथा A एवं B परस्पर अपवर्जी हो, तो P(A∪B) तथा P(A∩B) निकाले ।
View SolutionP(A∪B)=P(A)−P(B)+P(A∩B)
View SolutionFor any two sets A and B, prove that
P(A∩B)=P(A)∩P(B)
where P(A) is the power set of A.
View SolutionP(A∪B)=P(A∩B) होगा यदि और केवल यदि P(A) और P(B) के मध्य संबंध ……………………………. है।
View SolutionP(A)=23, P(B)=12 এবং P(A∩B)=16 হলে P(A∪B)-এর মান নির্ণয় করো।
View SolutionP(A)=13,P(B)=12,P(A∩B)=14 হলে P(Bc),P(A∩Bc),P(A∪B),P(B/A),P(Ac∩Bc),P(A∪Bc) -এর মান নির্ণয় করো। পরস্পর পৃথক কি না বলো।
View SolutionP(A)=13,P(B)=12,P(A∩B)=14 হলে P(Bc),P(A∩Bc),P(A∪B),P(B/A),P(Ac∩Bc),P(A∪Bc) -এর মান নির্ণয় করো। স্বাধীন কি না বলো ।
View Solutionदिया है, P(A∪B)=56,P(A∩B)=13 तथा P(B)=12, तब P(A) का मान है
View Solution
