Suppose $A = \int \frac{d x}{x^{2} + 6 x + 25}$ and $B = \int \frac{d x}{x^{2} - 6 x - 27}$ .
If $12 (A + B) = λ \cdot {tan}^{- 1} (\frac{x + 3}{4}) + μ \cdot ln ∣ ∣ ∣ \frac{x - 9}{x + 3} ∣ ∣ ∣ + C$ , then the value of $(λ + μ)$ is ... .Solution in Punjabi

Video Solution

Text Solution

Verified by Experts

The correct Answer is:4

Answer

Step by step video & image solution for Suppose A=int (dx)/(x^(2)+6x+25) and B=int(dx)/(x^(2)-6x-27). If 12(A+B)=lambda*tan^(-1)((x+3)/(4))+mu* ln |(x-9)/(x+3)|+C, then the value of (lambda+mu) is ... . by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS INDEFINITE INTEGRALS

Topper's Solved these Questions

BOOK - ARIHANT MATHSCHAPTER - INDEFINITE INTEGRAL EXERCISE - Exercise (Subjective Type Questions)
15videos
INDEFINITE INTEGRAL
BOOK - ARIHANT MATHSCHAPTER - INDEFINITE INTEGRAL EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
8videos
INDEFINITE INTEGRAL
BOOK - ARIHANT MATHSCHAPTER - INDEFINITE INTEGRAL EXERCISE - Exercise 5 ( Matching Type Questions )
2videos
INDEFINITE INTEGRAL
BOOK - ARIHANT MATHSCHAPTER - HYPERBOLAEXERCISE - Hyperbola Exercise 11 : Questions Asked in Previous 13 Years Exams
3videos
HYPERBOLA
BOOK - ARIHANT MATHSCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
8videos
INVERSE TRIGONOMETRIC FUNCTIONS

Similar Questions

The value of
$\int e^{(x sin x + cos x)} . (\frac{x^{4} {cos}^{3} x - x sin x + cos x}{x^{2} {cos}^{2} x}) d x$ , is equal to
03:18
View Solution
The integral $\int \frac{{sec}^{2} x}{{(sec x + tan x)}^{\frac{9}{2}}} d x$ equals (for some arbitrary constant $K) .$
03:18
View Solution
Find the mean marks of the following cummulative frequency table :

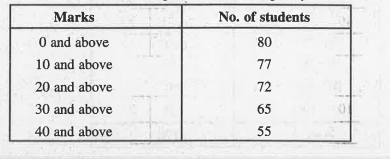
06:40
View Solution
which term of the following sequence : $3^{\frac{1}{2}}, 3, 3^{\frac{3}{2}}$ .....is 81?
05:09
View Solution
If $α = 3 {sin}^{- 1} (\frac{6}{11} and β = 3 {cos}^{- 1} (\frac{4}{9})$ , where the inverse trigonometric functions take only the principal values, then the correct options (s) is (are)
02:50
View Solution
int(2)/((2-x)^(2))((2-x)/(2+x))^(1/3){dx=lambda((2+x)/(2-x))^(mu)+c where lambda and mu are rational number in its simplest form then (lambda+(1)/(mu)) is equal to
03:19
View Solution
int(2)/((2-x)^(2))((2-x)/(2+x))^(1/3)dx=lambda((2+x)/(2-x))^(mu)+c where lambda and mu are rational number in its simplest form then (lambda+(1)/(mu)) is equal to
View Solution
If $\int \frac{1}{x^{3} + x^{4}} d x = \frac{A}{x^{2}} + \frac{B}{x} + log ∣ ∣ ∣ \frac{x}{x + 1} ∣ ∣ ∣ + C$ , then
02:45
View Solution
If $\int \frac{e^{x} (2 - x^{2})}{(1 - x) \sqrt{1 - x^{2}}} d x = μ e^{x} {(\frac{1 + x}{1 - x})}^{λ} + C$ , then $2 (λ + μ)$ is equal to ..... .
05:13
View Solution
Suppose $A = \int \frac{d x}{x^{2} + 6 x + 25}$ and $B = \int \frac{d x}{x^{2} - 6 x - 27}$ .
If $12 (A + B) = λ \cdot {tan}^{- 1} (\frac{x + 3}{4}) + μ \cdot ln ∣ ∣ ∣ \frac{x - 9}{x + 3} ∣ ∣ ∣ + C$ , then the value of $(λ + μ)$ is ... .
05:33
View Solution
If $\int \frac{6 x + 7}{{(x + 2)}^{2}} d x = A log | x + 2 | + \frac{B}{x + 2} + c$ then, find the values of A and B.
09:16
View Solution
$\begin{matrix} स्तम्भ (A) & स्तम्भ(B) 1 . & \int cot x d x & a . & \frac{1}{2} log x + c 2 . & \int tan x d x & b . & \frac{1}{2} tan x + c 3 . & \int \frac{d x}{1 + cos 2 x} & c . & log (sin x) + c 4 . & \int (1 + {tan}^{2} x) d x & d . & log (sec x) + c 5 . & \int cos e c x d x & e . & tan x + c f . & log (tan . \frac{x}{2}) + c \end{matrix}$
03:22
View Solution
$\int \frac{x - 1}{(x - 3) (x - 2)} d x = A ln (x - 3) + B ln (x - 2) + c$ , then find the value of $A + B$
View Solution
If the integral $I = \int \frac{d x}{x^{10} + x} = λ ln (\frac{x^{9}}{1 + x^{μ}}) + C$ , (where, C is the constant of integration) then the value of $\frac{1}{λ} + μ$ is equal to
05:33
View Solution
(a) $\int x^{3} {(log x)}^{2} d x$ (b) $\int x^{3} e^{x^{2}} d x$
02:51
View Solution
If $\int \frac{e^{x} (2 - x^{2})}{(1 - x) \sqrt{1 - x^{2}}} d x = μ e^{x} {(\frac{1 + x}{1 - x})}^{λ} + C$ , then $2 (λ + μ)$ is equal to ..... .
03:58
View Solution

ARIHANT MATHS-INDEFINITE INTEGRAL -Exercise (Single Integer Answer Type Questions)

If int((2x+3)dx)/(x(x+1)(x+2)(x+3)+1)=C-(1)/(f(x)) where f(x) is of th...
02:36
Let F(x) be the primitive of (3x+2)/(sqrt(x-9)) w.r.t. x. If F(10)=60,...
03:18
Let u(x) and v(x) be differentiable functions such that (u(x))/(v(x))=...
01:40
If int(1)/((x^(2)-1))ln((x-1)/(x+1))dx=6A[ln ((x-1)/(x+1))]^(2)+C, the...
03:05
If int(e^(x)(2-x^(2)))/((1-x)sqrt(1-x^(2)))dx=mu e^(x)((1+x)/(1-x))^(l...
05:30
Let f:R→R be a function as f(x)=(x-1)(x+2)(x-3)(x-6)-100. If g(x) is a...
01:38
Suppose A=int (dx)/(x^(2)+6x+25) and B=int(dx)/(x^(2)-6x-27). If 12(...
03:58
If int (x^(2020)+x^(804)+x^(402))(2x^(1608)+5x^(402)+10)^(1//402)dx=(1...
06:42
"If " int e^(x^(3)+x^(2)-1)(3x^(4)+2x^(3)+2x)dx=f(x)+C, " then the val...
05:41

Home
Ask Doubt
Profile

Suppose A=∫dxx2+6x+25 and B=∫dxx2−6x−27. If 12(A+B)=λ⋅tan−1(x+34)+μ⋅ln∣∣∣x−9x+3∣∣∣+C, then the value of (λ+μ) is ... .Solution in Punjabi

The correct Answer is:4

Topper's Solved these Questions

Similar Questions

ARIHANT MATHS-INDEFINITE INTEGRAL -Exercise (Single Integer Answer Type Questions)

Suppose $A = \int \frac{d x}{x^{2} + 6 x + 25}$ and $B = \int \frac{d x}{x^{2} - 6 x - 27}$ .
If $12 (A + B) = λ \cdot {tan}^{- 1} (\frac{x + 3}{4}) + μ \cdot ln ∣ ∣ ∣ \frac{x - 9}{x + 3} ∣ ∣ ∣ + C$ , then the value of $(λ + μ)$ is ... .Solution in Punjabi