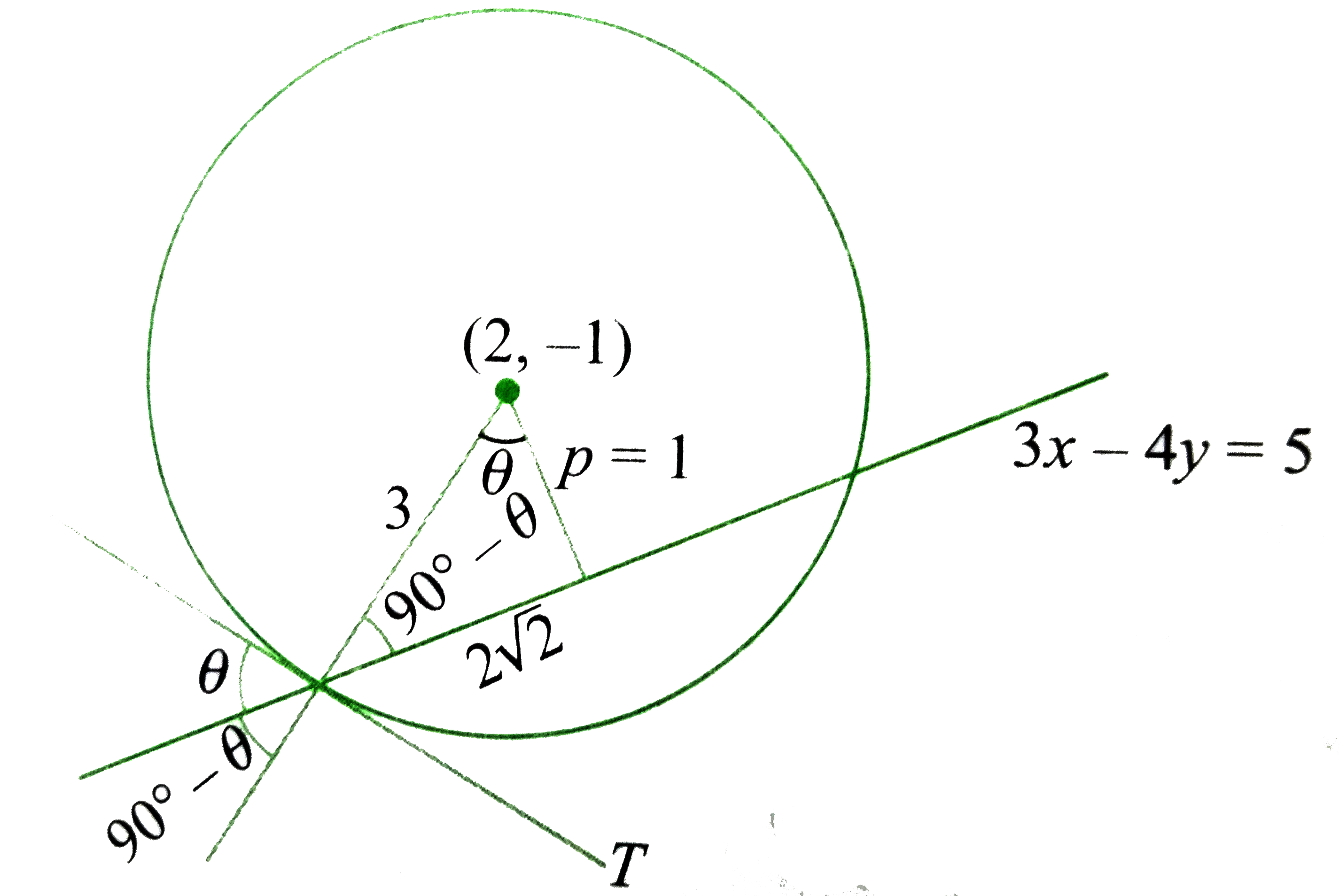
The acute angle between the line 3x−4y=5 and the circle x2+y2−4x+2y−4=0 is θ . Then 9cosθ=Solution in Bengali
The correct Answer is:3
θ is the angle between the tangent and the line
The circle has center (2,−1) and radius 3.
The length of perpendicular from the center on 3x−4y=5 is
p=∣∣∣6+4−55∣∣∣=1
or sin(90−θ)=13
or cosθ=13
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - ARCHIVES (JEE MAIN)1videos
CIRCLE
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - Single Correct Answer Type9videos
CIRCLE
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - MATRIX MATCH TYPE7videos
CIRCLE
- BOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension11videos
BINOMIAL THEOREM
- BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Comprehension Type8videos
CIRCLES
Similar Questions
If P and Q are the points of intersection of the circles x2+y2+3x+7y+2p=0 and x2+y2+2x+2y−p2=0 then there is a circle passing through P,Q and (1,1) for
02:42View SolutionThree distinct points A, B and C are given in the 2aedimensional coordinate plane such that the ratio of the distance of any one of them from the point (1, 0) to the distance from the point (–1, 0) is equal to 13.Then the circumcentre of the triangle ABC is at the point :
02:31View SolutionMatch the following lists.
 02:40View Solution
02:40View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' isView Solution
In the diagram as shown, a circle is drawn with centre C(1,1) and radius 1 and a line L. The line L is tangent to the circle at Q. Further L meets the y-axis at R and the x-axis at P in such a way that the angle OPQ equals θ where 0<θ<π2.
Coordinate of Q is05:41View SolutionThe acute angle between the line 3x−4y=5 and the circle x2+y2−4x+2y−4=0 is θ . Then 9cosθ=
05:11View SolutionThe line 4y−3x+λ=0 touches the circle x2+y2−4x−8y−5=0 then λ=
05:33View SolutionIf the acute angle between the lines ax2+2hxy+by2=0 is congruent to the acute angle between the lines 3x2−7xy+4y2=0, then
02:28View SolutionFor the circles x2+y2+4x+3y+2y=4=0,x2+y2+4x−2y+4=0 the line 3x+4y+5=0 is a
08:51View SolutionThe equation of circle through the intersection of circles: x2+y2−3x−6y+8=0 and x2+y2−2x−4y+4=0 and touching the line x+2y=5 is :
05:11View SolutionAcute angle between the line x−52=y+1−1=z+41 and the plane 3x – 4y – Z + 5 = 0 is
06:44View SolutionThe acute angle between the line 3x−4y=5 and the circle x2+y2−4x+2y−4=0 is θ . Then find the value of 9cosθ.
03:12View SolutionThe acute angle between the line 3x−4y=5 and the circle x2+y2−4x+2y−4=0 is θ . Then 9cosθ=
04:46View SolutionAcute angle between the line x−52=y+1−1=z+41 and the plane 3x−4y−z+5=0 is
04:23View SolutionIf θ is acute angle between the pair of lines x2−3xy+2y2+3x−5y+2=0 then sinθ=
04:24View Solutionयदि रेखा 3x−4y=5 तथा वृत
x2+y2−4x+2y−4=0 के मध्य न्यून कोण
का मान θ है, तो sinθ का मान होगा
(i) √53 (ii) 13 (iii) √23 (iv) 2√2301:31View Solution

