Question

about to only mathematics

Answer

5

Explanation

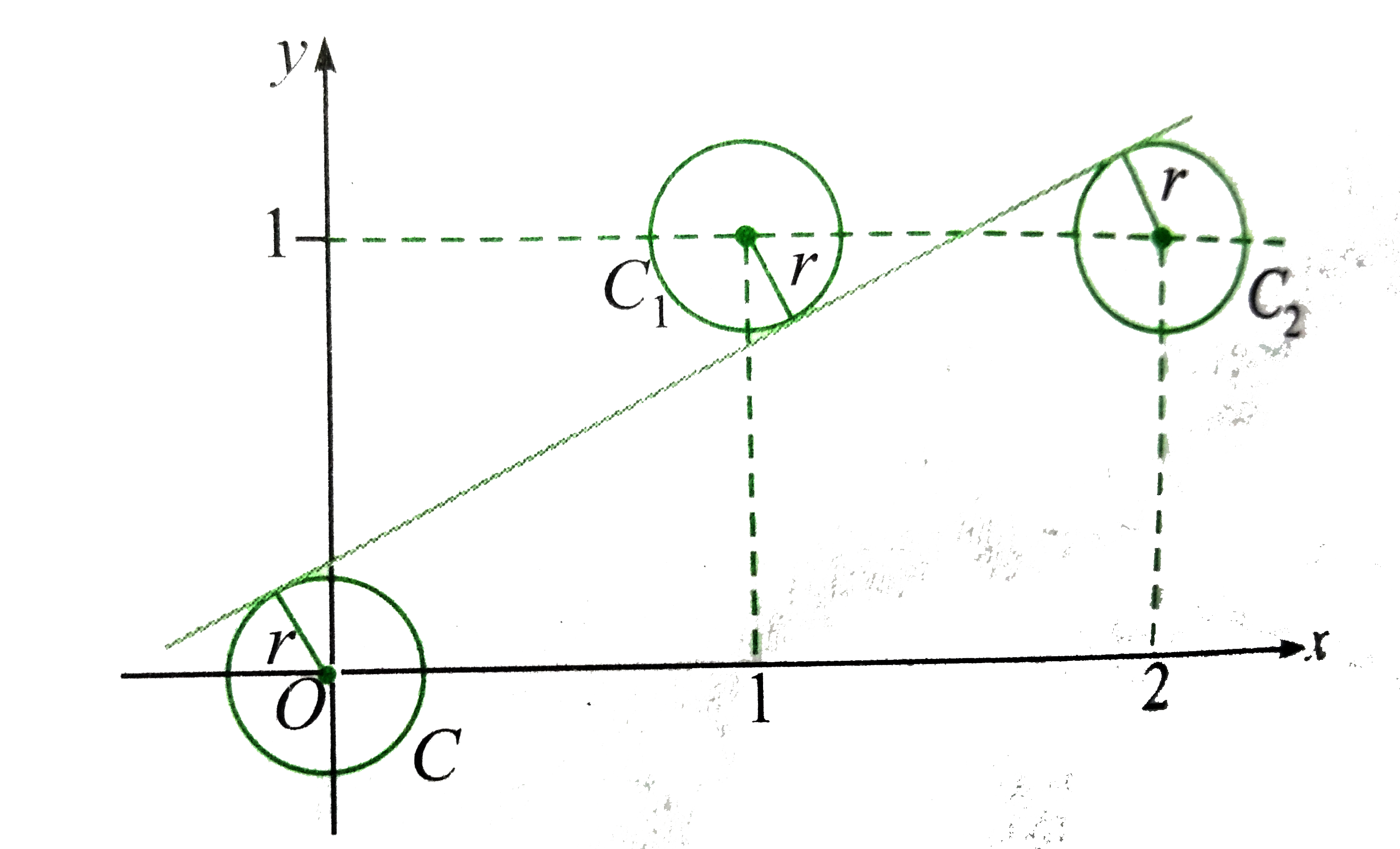
The equation of the line joining the origin and the center of circle `C_(2), (2,1)` , is
`y=(x )/(2)`
or `x-2y=0`
Let the equation of common tangent be
`x-2y+c=0` (1)
`:. ` Perpendicular distance from (0,0) on this line
`=` Perpendicular distance from `(1,1)`
or `|(c )/(sqrt(5))|=|(c-1)/(sqrt(5))|`
or `c=1-c` or `c=(1)/(2)`
The equation of common tangent is
`x-2y+(1)/(2) =0` or `2x-4y+1=0` (2)
The length of perpendicular from `(2,1)` on the line (2) is
`r= | (4-4+1)/(sqrt(20))|=(1)/(2sqrt(5))=(sqrt(5))/(10)`