The angle of elevation of the top of a tower from a point on the ground is 60∘. which is 25 m away from the foot of the tower. Find the height of the tower
The correct Answer is:25√3m
To find the height of the tower given the angle of elevation and the distance from the tower, we can follow these steps:
1. Understand the Problem:
We have a tower (let's denote it as AB) and a point on the ground (point C) that is 25 meters away from the foot of the tower (point B). The angle of elevation from point C to the top of the tower (point A) is 60 degrees.
2. Draw a Diagram:
Sketch a right triangle where:
- AB is the height of the tower (unknown).
- BC is the distance from the point on the ground to the foot of the tower, which is 25 meters.
- AC is the line of sight from point C to the top of the tower (point A).
- The angle ∠ACB is 60 degrees.
3. Identify the Trigonometric Ratio:
In this right triangle, we can use the tangent function because we have the opposite side (height of the tower AB) and the adjacent side (distance BC).
tan(θ)=OppositeAdjacent
Here, θ=60∘, Opposite = AB, and Adjacent = BC.
4. Set Up the Equation:
Using the tangent function:
tan(60∘)=ABBC
We know that tan(60∘)=√3 and BC=25 meters. So we can write:
√3=AB25
5. Solve for AB:
Rearranging the equation to find AB:
AB=25⋅√3
6. Calculate the Height:
The height of the tower (AB) is:
AB=25√3 meters
Final Result:
The height of the tower is 25√3 meters.
---
We have a tower (let's denote it as AB) and a point on the ground (point C) that is 25 meters away from the foot of the tower (point B). The angle of elevation from point C to the top of the tower (point A) is 60 degrees.
Sketch a right triangle where:
- AB is the height of the tower (unknown).
- BC is the distance from the point on the ground to the foot of the tower, which is 25 meters.
- AC is the line of sight from point C to the top of the tower (point A).
- The angle ∠ACB is 60 degrees.
In this right triangle, we can use the tangent function because we have the opposite side (height of the tower AB) and the adjacent side (distance BC).
tan(θ)=OppositeAdjacent
Here, θ=60∘, Opposite = AB, and Adjacent = BC.
Using the tangent function:
tan(60∘)=ABBC
We know that tan(60∘)=√3 and BC=25 meters. So we can write:
√3=AB25
Rearranging the equation to find AB:
AB=25⋅√3
The height of the tower (AB) is:
AB=25√3 meters
The height of the tower is 25√3 meters.
Topper's Solved these Questions
- SOME APPLICATIONS OF TRIGONOMETRYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Revision Exercise (short Answer Questions)
5 Videos
- SOME APPLICATIONS OF TRIGONOMETRYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Revision Exercise (long Answer Questions)
1 Videos
- SOME APPLICATIONS OF TRIGONOMETRYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Long Answer Questions
5 Videos
- REAL NUMBERSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - REAL NUMBERSEXERCISE - Revision Exercise Long Answer Questions
5 Videos
- STATISTICS BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions
4 Videos
Similar Questions
The angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View Solution
Knowledge Check
The angle of elevation of the top of a tower. from a point on the ground and at a distance of 160 m from its foot, is find to be 60∘ . Find the height of the tower .
A277.12 mB377.12 mC477.12 mDNone
The angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower is 30∘ . Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View Solution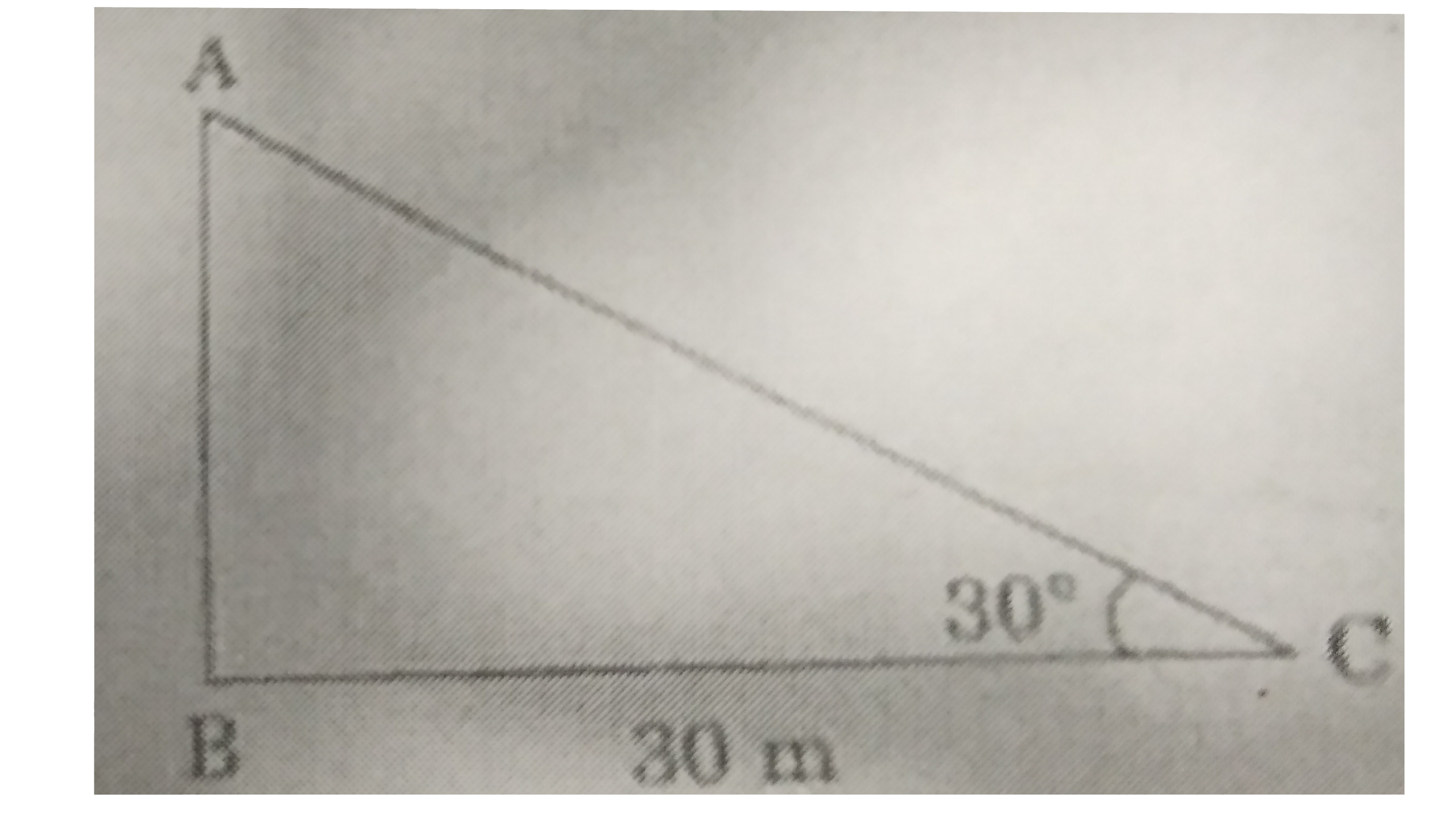
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.

View SolutionIn figure , the angle of elevation of the top of a tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m, Find the distance of the point from the foot of the power .
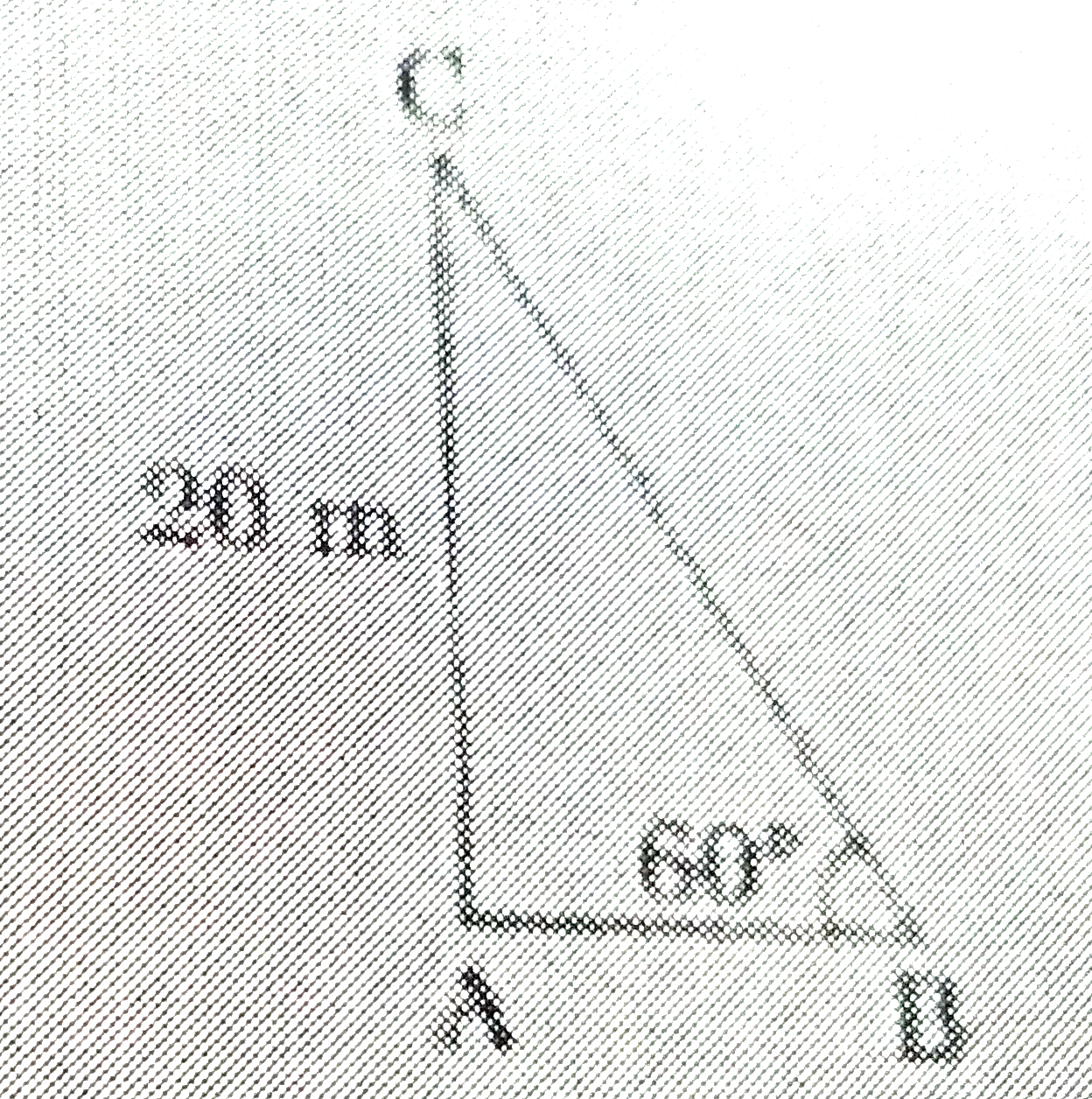
View SolutionIn the figure-2, the angle of elevation of the top of tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m. find the distance of the point from the foot of the tower.
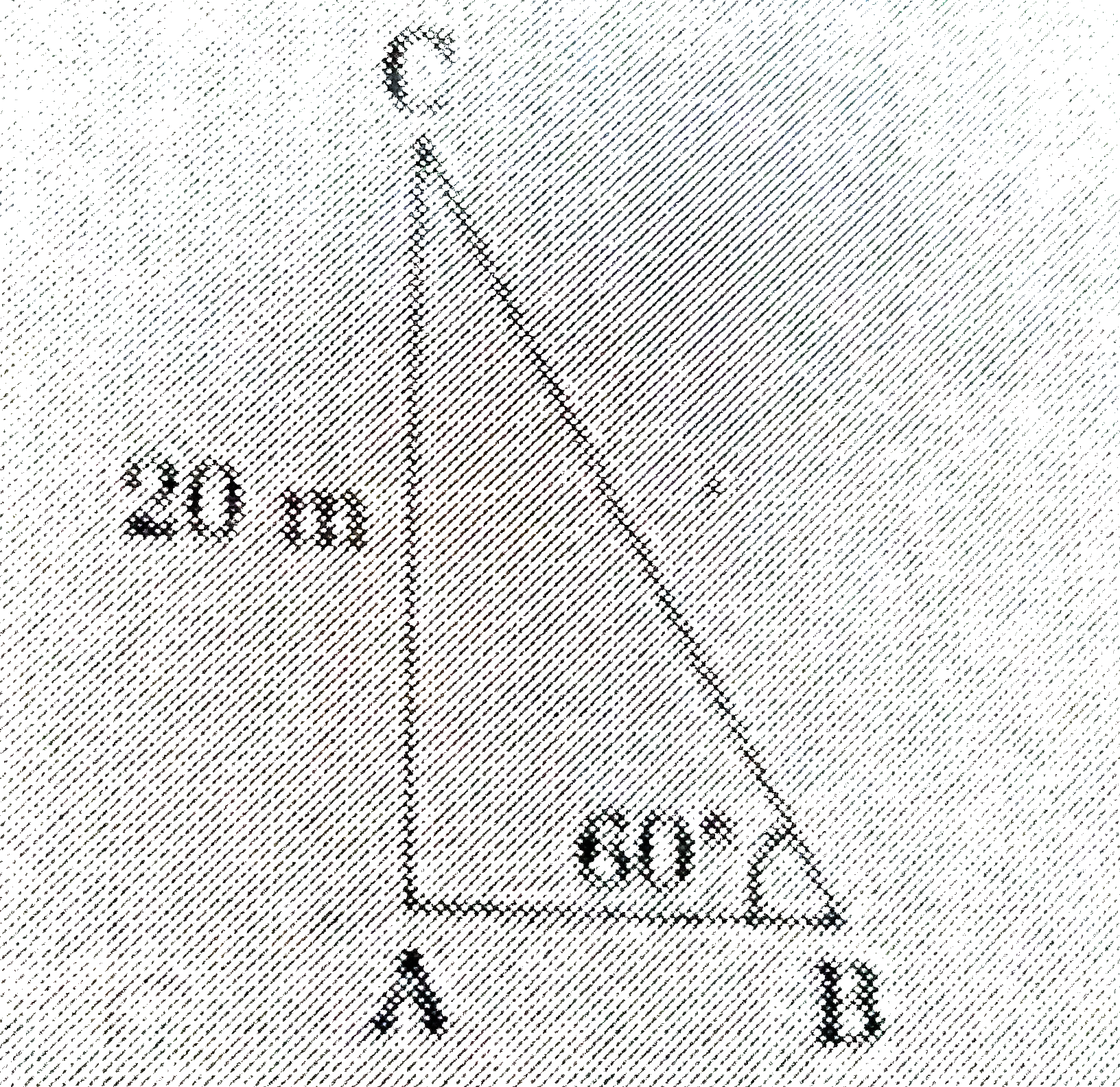
View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View Solution
