The angle of elevation of top of the tower from a point P on the ground is 30∘ . If the points is 45 m away from the foot of the tower , then the height of the tower is
45 m
15 m
15√3
20√3m
The correct Answer is:C
To find the height of the tower based on the given information, we can follow these steps:
Step 1: Understand the problem
We have a point P on the ground from which the angle of elevation to the top of the tower is given as 30∘. The distance from point P to the foot of the tower is 45 meters.
Step 2: Draw a diagram
Visualize the scenario by drawing a right triangle where:
- The height of the tower is the perpendicular side (let's call it h).
- The distance from point P to the foot of the tower is the base (45 m).
- The angle of elevation from point P to the top of the tower is 30∘.
Step 3: Set up the trigonometric relationship
In a right triangle, the tangent of an angle is defined as the ratio of the opposite side (height of the tower) to the adjacent side (distance from point P to the foot of the tower). Therefore, we can write:
tan(30∘)=h45
Step 4: Substitute the value of tan(30∘)
We know that:
tan(30∘)=1√3
Substituting this into the equation gives:
1√3=h45
Step 5: Solve for h
To find h, we can rearrange the equation:
h=45⋅1√3=45√3
Step 6: Rationalize the denominator
To express h in a more standard form, we can rationalize the denominator:
h=45√3⋅√3√3=45√33
Step 7: Simplify
Now, simplify the fraction:
h=15√3
Final Answer
Thus, the height of the tower is:
h=15√3 meters
---
We have a point P on the ground from which the angle of elevation to the top of the tower is given as 30∘. The distance from point P to the foot of the tower is 45 meters.
Visualize the scenario by drawing a right triangle where:
- The height of the tower is the perpendicular side (let's call it h).
- The distance from point P to the foot of the tower is the base (45 m).
- The angle of elevation from point P to the top of the tower is 30∘.
In a right triangle, the tangent of an angle is defined as the ratio of the opposite side (height of the tower) to the adjacent side (distance from point P to the foot of the tower). Therefore, we can write:
We know that:
To find h, we can rearrange the equation:
To express h in a more standard form, we can rationalize the denominator:
Now, simplify the fraction:
Thus, the height of the tower is:
Topper's Solved these Questions
- BOOK - TARGET PUBLICATIONCHAPTER - TRIGONOMETRY EXERCISE - Additional Problems for Practice (Based on practice Set 6.1)27videos
TRIGONOMETRY
- BOOK - TARGET PUBLICATIONCHAPTER - TRIGONOMETRY EXERCISE - Based on Practice Set 6.213videos
TRIGONOMETRY
- BOOK - TARGET PUBLICATIONCHAPTER - TRIGONOMETRY EXERCISE - Activities for practice3videos
TRIGONOMETRY
- BOOK - TARGET PUBLICATIONCHAPTER - STATISTICSEXERCISE - Problem Set-621videos
STATISTICS
Similar Questions
If the angle of elevation of top of a tower from a point on the ground which is 20√3 m away from the foot of the tower is 30∘, then find the height of the tower.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The angle of elevation of the top of a tower from a point on the ground which is 30 m away from the foot of the tower , is 30∘ . Then , the height ( in m) of the tower is .
A10√3B30√3C10√3D10 - Question 2 - Select One
Assertion (A): The angle of elevation of the top of the tower from a point on the ground, which is 30 m away from the foot of the tower, is 30∘. The height of the tower is 10 m.
Reason (R): The angle of depression from B to A and Angle of elevation from A to B are equal.ABoth A and R are true and R is the correct explanation of A.BBoth A and R are true and R is not correct explanation of A.CA is true but R is false.DA is false but R is true.
The angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 25 m away from the foot of between the ship and tower.
View Solution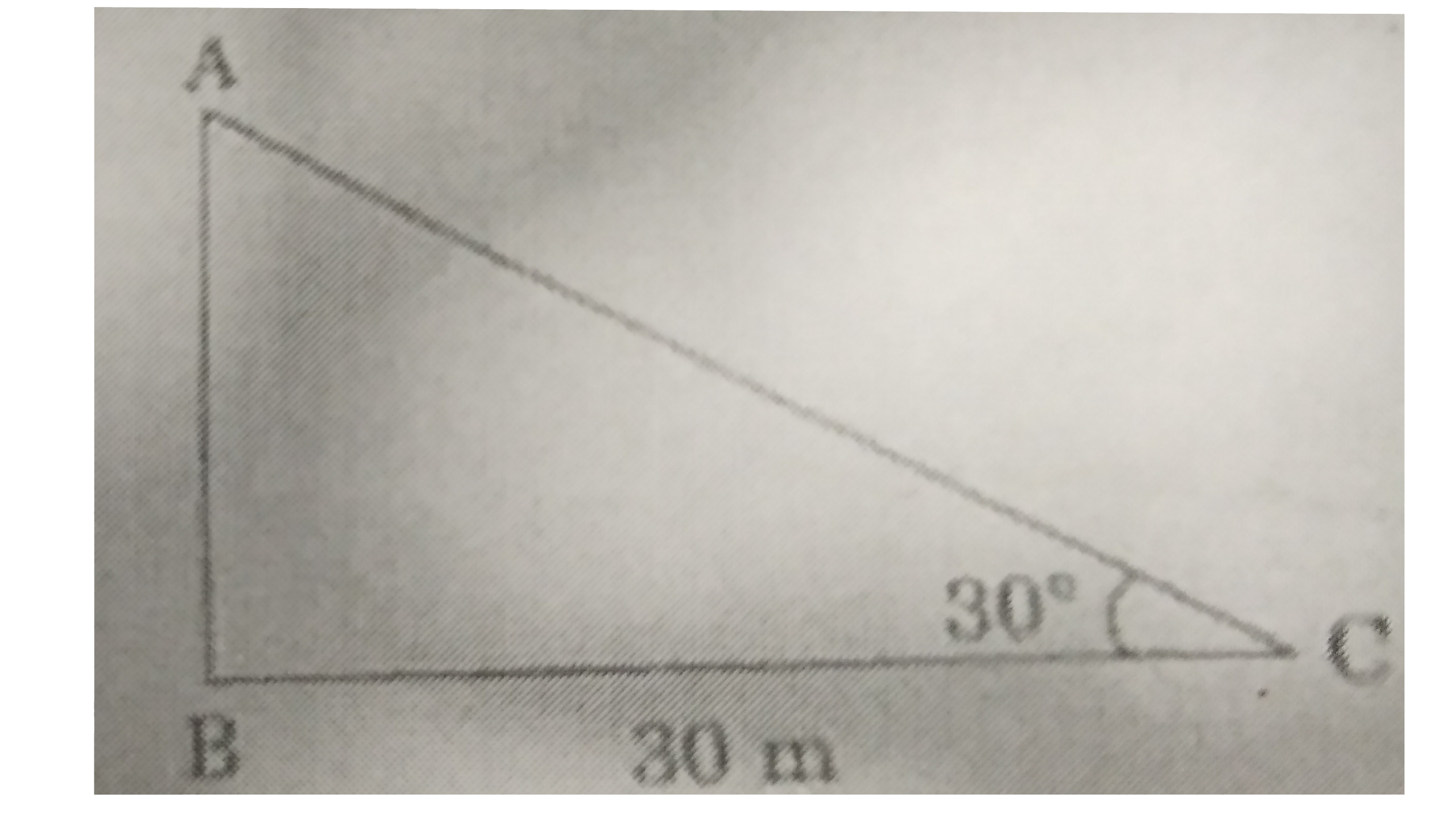
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View Solution

