The area between the curve y=2x4−x2, the x-axis, and the ordinates of the two minima of the curve isSolution in Tamil
11/60 sq. units
7/120 sq. units
1/30 sq. units
7/90 sq. units
The correct Answer is:B
The curve is y=2x4−x2=x2(2x2−1)
The curve is symmetrical about the axis of y.
Also, it is a polynomial of 4 degree having roots 0,0,±1√2.x=0 is a repeated root. Hence graph touches at (0,0).
The curve intersects the axes at O(0,0),A(−1/√2,0)andB(1/√2,0).
Thus, the graph of the curve is as shown in the figure.
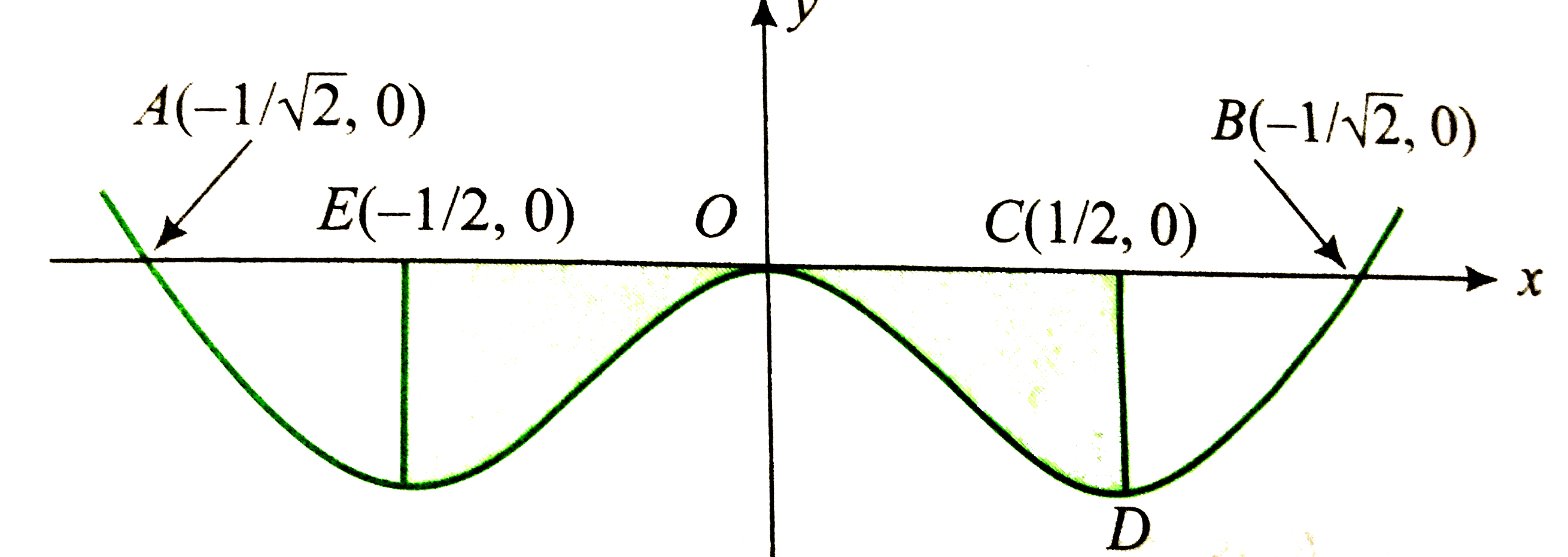
Here, y≤0, as x varies from x=−1/2 to x=1/2
∴ required area =2 Area OCDO
=2∣∣∣∫1/20ydx∣∣∣
=2∣∣∣∫1/20(2x4−x2)dx∣∣∣
=7/120 sq. units
Topper's Solved these Questions
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Multiple)
10 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Comprehension)
21 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.3
7 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGECHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Subjective Type
2 Videos
- AREA UNDER CURVESBOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank
10 Videos
Similar Questions
Let A(k) be the area bounded by the curves y=x2−3 and y=kx+2 The range of A(k) is (10√53,∞) The range of A(k) is (20√53,∞) If function k→A(k) is defined for k∈[−2,∞ ), then A(k) is many-one function. The value of k for which area is minimum is 1.
View SolutionLet Ar be the area of the region bounded between the curves y2=(e−kr)x(where k>0,r∈N) and the line y=mx(where m≠0), k and m are some constants
A1,A2,A3,… are in G.P. with common ratio
View SolutionFind the continuous function f where (x4−4x2)≤f(x)≤(2x2−x3) such that the area bounded by y=f(x),y=x4−4x2. then y-axis, and the line x=t, where (0≤t≤2) is k times the area bounded by y=f(x),y=2x2−x3,y−aξs, and line x=t(where0≤t≤2).
View SolutionProve that for λ>1, the equation xlogx+x=λ has least one solution in [1,λ].
View SolutionThe area of the region (s) enclosed by the curves y=x2 and y=√|x| is
View SolutionThe area between the curve y=2x4−x2, the axis, and the ordinates of the two minima of the curve is 11/60 sq. units (b) 7/120 sq. units 1/30 sq. units (d) 7/90 sq. units
View SolutionThe area between the curves y=2x^(4)-x^(2) the x -axis and the ordinates of two minima of the be curve is (A) (7)/(240) (B) (7)/(120) (C) (7)/(60) (D) None of these
View SolutionFind the area under the curve y=(x^(2)+2)^(2)+2x between the ordinates x=0 and x=2
View SolutionShow that the area between the curve y=ce2x, the x-axis and any two ordinates is proportional to the difference between the ordinates, c being constant.
View Solution(i) Find the area bounded by x2+y2−2x=0 and y=sin'πx2 in the upper half of the circle.
View SolutionThe area between the curve y=2x4−x2, the x-axis, and the ordinates of the two minima of the curve is
View SolutionThe area bounded by the curve y=x4−2x3+x2+3 with x-axis and ordinates corresponding to the minima of y, is
View SolutionThe area between the curve y=2x4−x2,the x, axis and the ordiantes of two minimum of curve is
View SolutionIf the area between the curves y=2x4−x2, the x-axis and the ordinates of two minima of the curve is k, then 120 k is _______
View SolutionThe area between the curve y=2x4−x2, the X-axis and the ordinates of two minima of the curve is
View SolutionThe area between the curve y=2x4−x2, the x-axis, and the ordinates of the two minima of the curve is
View Solution
