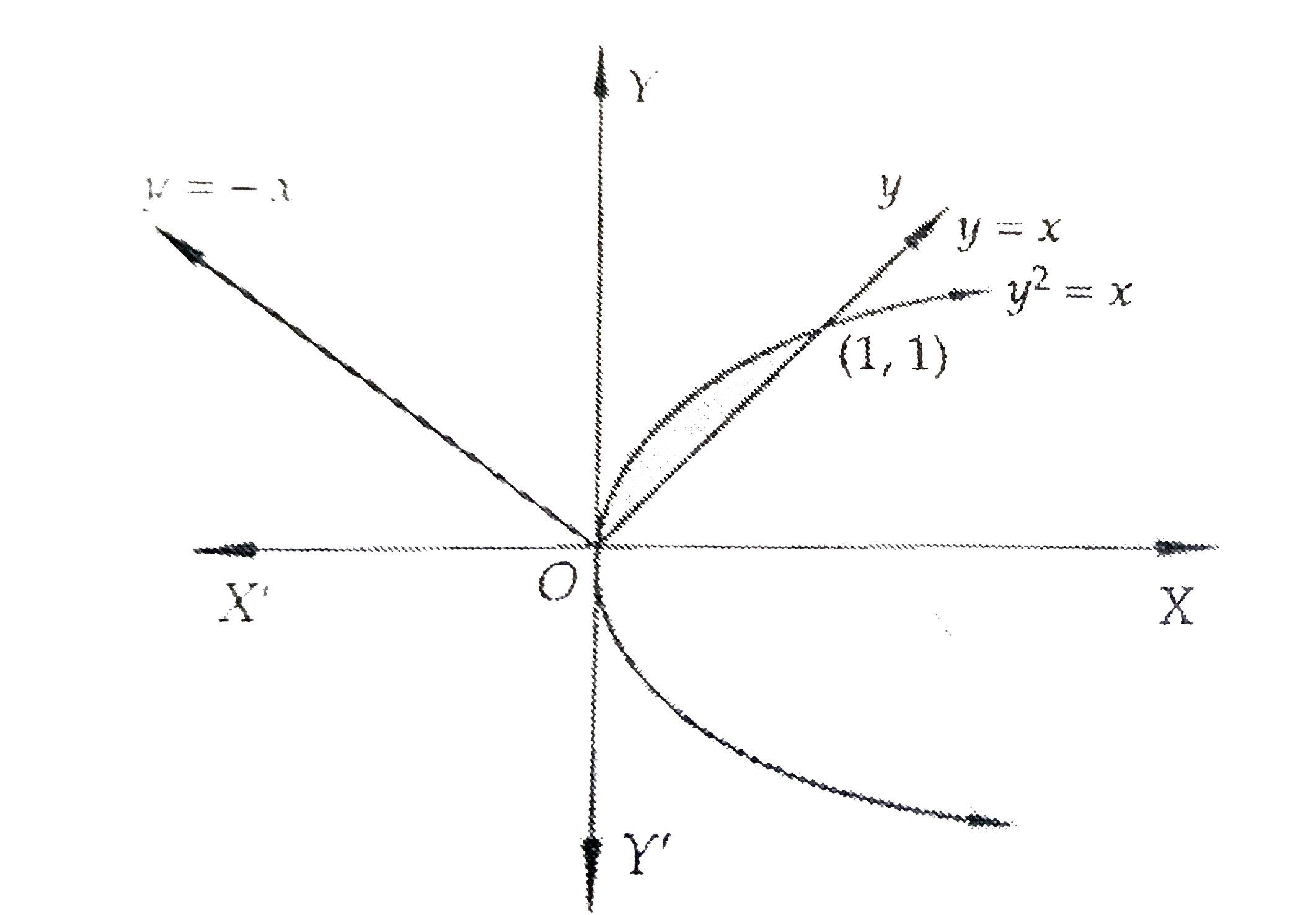
The area enclosed between the curves $y^{2} = x and y = | x |$ is

A

$\frac{1}{6}$

B

$\frac{1}{3}$

C

$\frac{2}{3}$

D

1

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Let A be the area enclosed between the given curves, then
$A = (\sqrt{x} - x) d x {[\frac{2}{3} x^{3 / 2} - \frac{x^{2}}{2}]}_{0}^{3 / 2} = \frac{1}{3}$

|

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMACHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Exercise
62 Videos
AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMACHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Chapter Test
60 Videos
APPLICATION OF DERIVATIVESBOOK - OBJECTIVE RD SHARMACHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Illustration
6 Videos
CARTESIAN PRODUCT OF SETS AND RELATIONS BOOK - OBJECTIVE RD SHARMACHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test
31 Videos

Similar Questions

Knowledge Check

The area enclosed between the curves $y^{2} = x and y = | x |$ is :
A $2 / 3$
B1
C $1 / 6$
D $1 / 3$
The area enclosed between the curves $y = x^{3}$ and $y = \sqrt{x}$ is [in sq unit]
A44319
B44320
C44328
D44535
The area enclosed between the curves $y = x^{3}$ and $y = \sqrt{x}$ is
A $\frac{5}{3}$ sq units
B $\frac{5}{4}$ sq units
C $\frac{5}{12}$ sq unit
D $\frac{12}{5}$ sq units

OBJECTIVE RD SHARMA-AREAS OF BOUNDED REGIONS-Chapter Test

Home
Ask Doubt
Profile