The area of a quadrilateral is and the perpendiculars drawn to one of the diagonal from the opposite vertices are 10m and 8m. Find the length of the diagonal.
The correct Answer is:40m
To find the length of the diagonal of the quadrilateral given the area and the lengths of the perpendiculars from the opposite vertices, we can follow these steps:
Step 1: Understand the Problem
We are given:
- Area of the quadrilateral = 360 m²
- Perpendiculars from opposite vertices to the diagonal:
- Length of perpendicular from vertex B (BK) = 10 m
- Length of perpendicular from vertex D (DM) = 8 m
We need to find the length of the diagonal AC.
Step 2: Set Up the Area Equation
The area of a quadrilateral can be expressed as the sum of the areas of two triangles formed by the diagonal. Thus, we can write:
Area of Quadrilateral=Area of △ABC+Area of △ADC
Step 3: Calculate the Areas of the Triangles
The area of a triangle can be calculated using the formula:
Area=12×base×height
For both triangles, the base is the length of the diagonal AC. Therefore, we can express the areas as:
Area of △ABC=12×AC×BK=12×AC×10
Area of △ADC=12×AC×DM=12×AC×8
Step 4: Combine the Areas
Now we can combine these areas:
360=12×AC×10+12×AC×8
Step 5: Factor Out the Common Terms
Factoring out 12×AC:
360=12×AC×(10+8)
This simplifies to:
360=12×AC×18
Step 6: Solve for AC
To isolate AC, multiply both sides by 2:
720=AC×18
Now, divide both sides by 18:
AC=72018
Calculating this gives:
AC=40m
Conclusion
The length of the diagonal AC is 40 meters.
---
We are given:
- Area of the quadrilateral = 360 m²
- Perpendiculars from opposite vertices to the diagonal:
- Length of perpendicular from vertex B (BK) = 10 m
- Length of perpendicular from vertex D (DM) = 8 m
The area of a quadrilateral can be expressed as the sum of the areas of two triangles formed by the diagonal. Thus, we can write:
The area of a triangle can be calculated using the formula:
Now we can combine these areas:
Factoring out 12×AC:
To isolate AC, multiply both sides by 2:
The length of the diagonal AC is 40 meters.
Topper's Solved these Questions
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - HERON'S FORMULAEXERCISE - PART D3videos
HERON'S FORMULA
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - HERON'S FORMULAEXERCISE - PRACTICE TEST8videos
HERON'S FORMULA
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - HERON'S FORMULAEXERCISE - PART B8videos
HERON'S FORMULA
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - CONSTRUCTIONSEXERCISE - QUESTIONS7videos
CONSTRUCTIONS
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - INTRODUCTION TO EUCLID'S GEOMETRYEXERCISE - PRACTICE TEST8videos
INTRODUCTION TO EUCLID'S GEOMETRY
Similar Questions
A diagonal of a quadrilateral is 26 cm and the perpendiculars drawn to it from the opposite vertices are 12.8 cm and 11.2 cm. Find the area of the quadrilateral.
View SolutionA diagonal of a quadrilateral is 30.5m long. The perpendiculars to the diagonal from the opposite vertices are of lengths 8.5m and 7.5m.Find the area of the quadrilateral
View Solution
Knowledge Check
- Question 1 - Select One
The diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on its from the remaining opposite vertices are 8m and 13m. The area of the field is
A252m2B156m2C96m2D1152m2 - Question 2 - Select One
The diagonal of a quadrilateral is 20 cm in length and the lengths of perpendiculars on it from the opposite vertices are 8.5 cm and 11.5 cm. The area of the quadrilateral is
A400cm2B200cm2C300cm2D240cm2 - Question 3 - Select One
The area of a square is 16200m2. Find the length of its diagonal.
A170mB180mC160mD280m
The diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on it from the remaining opposite vertices are 8 m and 13 m. Find the area of the field.
View SolutionOne diagonal of a quadrilateral is 20 m long and the perpendiculars to it from the opposite vertices are 8.5 m and 11 m. Find the areaof the quadrilateral.
View SolutionThe diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on it from the remaining opposite vertices are 8 m and 13 m. Find the area of the field.
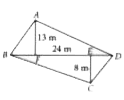 View Solution
View SolutionA diagonal of a quadrilateral is 30 cm in length and the lengths of perpendiculars to it from the opposite vertices are 6.8 cm and 9.6 cm. Find the area of the quadrilateral.
View SolutionThe area of a square is 784m2, find the length of the diagonal.
View Solution

