The area of the circle centred at (1,2) and passing through (4,6) is
A
5π
B
10π
C
25π
D
none of these
Video Solution
Text Solution
The correct Answer is:C
Given that, centre of the pcircle is (1,2)
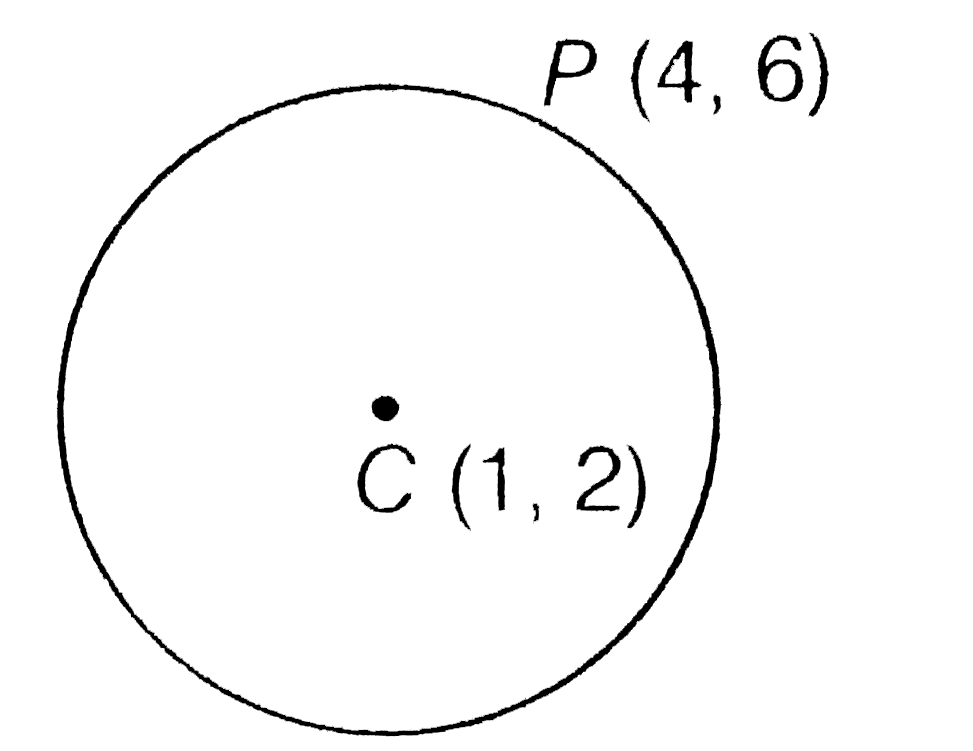
∵ CP=√9+16=5=Radius of the circle
∴ Required area=πr2=25π
|
Topper's Solved these Questions
- CONIC SECTIONSBook:NCERT EXEMPLARChapter:CONIC SECTIONSExercise:Fillers
Explore 6 Videos
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBook:NCERT EXEMPLARChapter:COMPLEX NUMBERS AND QUADRATIC EQUATIONSExercise:OBJECTIVE TYPE QUESTIONS
Explore 16 Videos
- INTRODUCTION TO THREE DIMENSIONAL GEOMETRYBook:NCERT EXEMPLARChapter:INTRODUCTION TO THREE DIMENSIONAL GEOMETRYExercise:Fillers
Explore 16 Videos
Similar Questions
Knowledge Check
The area of circle centred at (1,2) and passing through (4,6) is
A5πB10πC25πDnone of theseThe area of circle centred at (1,2) and passing through (4,6) is
A5πB10πC25πDNone of theseEquation of circle centred at (1,-1) , and passing through (3,2) , is
Ax2+y2−2x−2y−11=0Bx2+y2−2x+2y−11=0Cx2+y2+2x+2y−11=0Dx2+y2−2x+2y+11=0
Let C be the circle with centre at (1, 1) and radius 1 If Tis the circle centred at (0, y), passing through origin and touching the circle C externally, then the radius of T is equal to V3 (a) 4
View SolutionFind the equation of a circle with centre (2, 2) and passes through the point (4, 5).
View SolutionEquation of circle centred at (2,-3) , and passing through (-1,2) , is
View SolutionEquation of the circle with centre at (1,-2) , and passing through the centre of the circle x2+y2+2y−3=0 , is
View SolutionEquation of circle centred on Y-axis , and passing through
(4,6) and (6,11), is
View Solution
