The arithmetic mean and mode of a data are 24 and 12 respectively, then its median is (a) 25 (b) 18 (c) 20 (d) 22
To find the median of the data given that the arithmetic mean is 24 and the mode is 12, we can use the relationship between mean, median, and mode.
1. Identify the given values:
- Mean (M) = 24
- Mode (Mo) = 12
2. Use the formula relating mode, median, and mean:
The formula is:
Mode=3×Median−2×Mean
3. Substitute the known values into the formula:
12=3×Median−2×24
4. Simplify the equation:
- First, calculate 2×24:
2×24=48
- Substitute this back into the equation:
12=3×Median−48
5. Rearrange the equation to isolate the median:
- Add 48 to both sides:
12+48=3×Median
60=3×Median
6. Solve for the median:
- Divide both sides by 3:
Median=603=20
7. Conclusion:
Therefore, the median of the data is 20.
Final Answer:
The median is 20, which corresponds to option (c).
- Mean (M) = 24
- Mode (Mo) = 12
The formula is:
Mode=3×Median−2×Mean
12=3×Median−2×24
- First, calculate 2×24:
2×24=48
- Substitute this back into the equation:
12=3×Median−48
- Add 48 to both sides:
12+48=3×Median
60=3×Median
- Divide both sides by 3:
Median=603=20
Therefore, the median of the data is 20.
The median is 20, which corresponds to option (c).
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
If the mean and the mode of a moderately skewed frequency distribution are 90 and 96 respectively, then the median is
A93B92C98D88 - Question 2 - Select One
If the median and mean of moderately asymmetrical frequency distribution are 72 and 74 respectively, then the mode is
A68B76C75D70
If mode and mean of data are found 28 and 24 respectively, find median using empirical formula.
View SolutionWhat is the arithmetic mean of 4, 8, 12, 16,20, and 24 ?
View SolutionFind mean, median and mode of the following data :
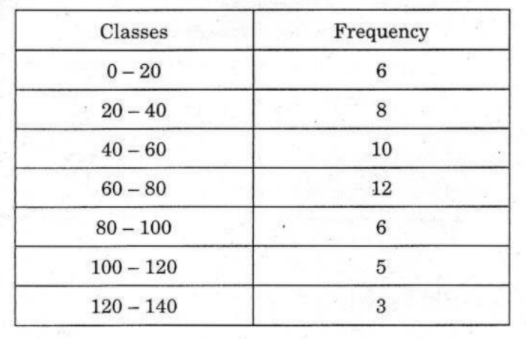 View Solution
View SolutionFind the mean, median and mode of the following data :
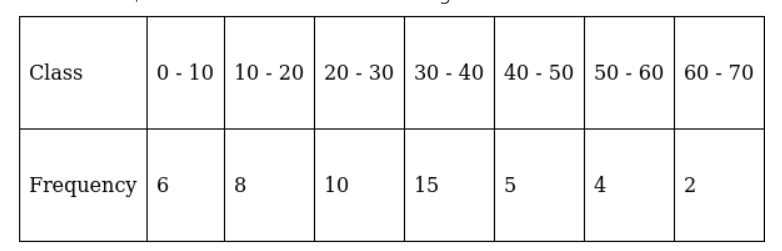 View Solution
View SolutionFind the mean, median and mode of the following data
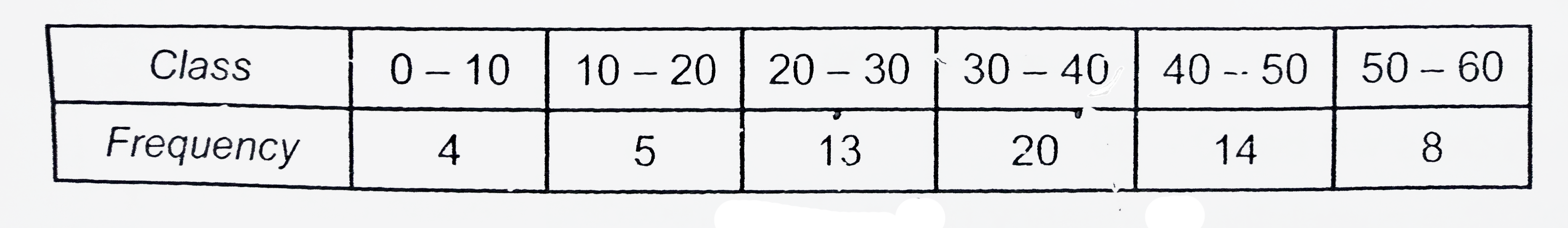 View Solution
View SolutionIf the difference of mode and median of a data is 24, then the difference of median and mean is (a) 12 (b) 24 (c) 8 (d) 36
View Solution

