The arithmetic mean of the following data is 25, find the value of . : 5 15 25 35 45 : 3 3 6 2
To find the value of k given that the arithmetic mean of the data is 25, we can follow these steps:
Step 1: Write down the given data
We have the following values for xi and fi:
- xi: 5, 15, 25, 35, 45
- fi: 3, k, 3, 6, 2
Step 2: Calculate the sum of frequencies
The sum of frequencies ∑fi can be expressed as:
∑fi=3+k+3+6+2=14+k
Step 3: Calculate the sum of xifi
Now, we calculate ∑(xifi):
∑(xifi)=(5×3)+(15×k)+(25×3)+(35×6)+(45×2)
Calculating each term:
- 5×3=15
- 15×k=15k
- 25×3=75
- 35×6=210
- 45×2=90
Now, summing these values:
∑(xifi)=15+15k+75+210+90=390+15k
Step 4: Use the formula for the arithmetic mean
The formula for the arithmetic mean ¯x is given by:
¯x=∑(xifi)∑fi
Substituting the known values:
25=390+15k14+k
Step 5: Cross-multiply to solve for k
Cross-multiplying gives:
25(14+k)=390+15k
Expanding the left side:
350+25k=390+15k
Step 6: Rearrange the equation
Now, we rearrange the equation to isolate k:
350+25k−15k=390
350+10k=390
Subtracting 350 from both sides:
10k=40
Step 7: Solve for k
Dividing both sides by 10:
k=4
Final Answer
The value of k is 4.
---
We have the following values for xi and fi:
- xi: 5, 15, 25, 35, 45
- fi: 3, k, 3, 6, 2
The sum of frequencies ∑fi can be expressed as:
∑fi=3+k+3+6+2=14+k
Now, we calculate ∑(xifi):
∑(xifi)=(5×3)+(15×k)+(25×3)+(35×6)+(45×2)
Calculating each term:
- 5×3=15
- 15×k=15k
- 25×3=75
- 35×6=210
- 45×2=90
∑(xifi)=15+15k+75+210+90=390+15k
The formula for the arithmetic mean ¯x is given by:
¯x=∑(xifi)∑fi
Substituting the known values:
25=390+15k14+k
Cross-multiplying gives:
25(14+k)=390+15k
Expanding the left side:
350+25k=390+15k
Now, we rearrange the equation to isolate k:
350+25k−15k=390
350+10k=390
Subtracting 350 from both sides:
10k=40
Dividing both sides by 10:
k=4
The value of k is 4.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
If the arithmetic mean of the following data is 7, then a + b =
xi4679fia4b5A4B2C3Dcannot be determined
The arithmetic mean of the following frequency distribution is 53. Find the value of k.
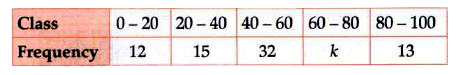 View Solution
View SolutionThe arithmetic mean of the following frequency distribution is 53. Find the value of k.
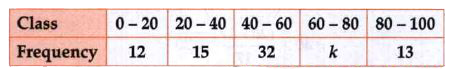 View Solution
View SolutionIf the mean of the following data is 18.75. Find the value of p . xi : 10 15 p 25 30 fi : 5 10 7 8 2
View SolutionThe mean of the following data is 20.6. Find the value of 10 15 p 25 35 3 10 25 7 5
View SolutionIf the mean of the following data is 20.6. Find the value of p . x : 10 15 p 25 35 f : 3 10 25 7 5
View SolutionIf the mean of the following data is 15, find p . x : 5 10 15 20 25 f : 6 p 6 10 5
View SolutionIf 3k−7 is the arithmetic mean of 3k+4 and k+12 then the value of 2k+5
View Solution


