The corner points of the feasible region determined by the following system of linear inequalities:
2x+y≥10,x+3y≤15,x,y≥0 are (0,0),(5,0),(3,4) and (0,5). Let Z=px+qy, where p,q≥0. Condition on p and q so that the maximum of Z occurs at both (3,4) and (0,5) is:
(a) p=q (b) p=2q (c) p=eq (d) q=3p
Since, maximum of Z=px+qy occurs at both points (3,4) and (0,5)
Hence value of Z=px+qy must be equal at both the points.
∴p×3+q×4=p×0+q×5
⇒3p+4q=5q
⇒3p=q
So, q=3p is the correct answer.
Topper's Solved these Questions
- LINEAR PROGRAMMINGBOOK - NCERTCHAPTER - LINEAR PROGRAMMINGEXERCISE - SOLVED EXAMPLES
11 Videos
- LINEAR PROGRAMMINGBOOK - NCERTCHAPTER - LINEAR PROGRAMMINGEXERCISE - Miscellaneous Exercise
10 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - NCERTCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - MISCELLANEOUS EXERCISE
17 Videos
- MATRICESBOOK - NCERTCHAPTER - MATRICESEXERCISE - EXERCISE 3.3
12 Videos
Similar Questions
The corner points of the feasible region of the system of linear inequations x+y≥2,x+y≤5,x−y≥0,x,y≥0 are :
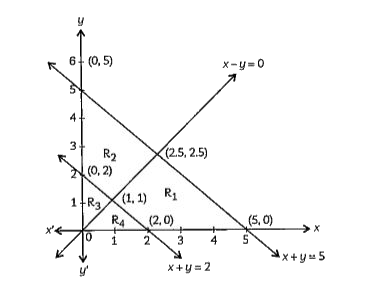
View SolutionThe corner points of a feasible region determined by a system of linear inequations are (0,0),(4,0),(5,2),(2,2) and (0, 1). If the objective function is Z = x + y, then maximum of Z occurs at:
View Solution
Knowledge Check
The carner points of the feasible region formed by the system of linear inequations x−y≥−1,−x+y≥0,x+y≤2andx,y≥0, are
A(0,0),(−1,0),(0,1)B(0,0),(2,0),(1,1)C(0,1),(1,1),(0,0)D(0,0),(1,1),(12,32),(0,1)The corner points of the feasible region determined by the system of linear constraints are (0, 10), (5, 5) (15, 15), (0, 20). Let Z = px + qy , where p,q>0. Then, the condition on p and q so that the maximum of Z occurs at both the points (15, 15) and (0, 20), is
Ap=qBp=2qCq=2pDq=3pThe corner points of the feasible region determined by the system of linear constraints are (0,10) , (5,5) (25,20),(0,30) Let z = px + qy , where p,q>0 Condition on p and q so that te maximum of z occurs at both the points (25,20 ) and (0,30) is ………
A5p = 2qB2p=5qCp = 2qDq = 3p
The vertex of the linear inequalities 2x+3y≤6,x+4y≤4,x≥0,y≥0 is
View SolutionCorner points of the feasible region determined by the system of linear constraints are (0,3), (1,1), and (3,0). Let Z=px+qy. Where p,q<0 Condition on p and q, so that the minimum of Z occurs at (3,0) and (1,1) is
View SolutionThe corner points of the feasible region determined by a system of linear inequations are (0,0),(4,0),(2,4) and (0,5). If the minimum value of Z=ax+by,a, b>0 occurs at (2,4) and (0,5) ,then :
View SolutionThe feasible region of the system of linear inequations x+2y≤120,x+y≥60,x−2y≥0,x,y≥0:
View SolutionThe corner points of the feasible region of a system of linear inequations are (0, 0), (5, 0), (6,5), (6, 8), (4, 10) and (0,8). If Z = 3x - 4y, then the minimum value of Z occurs at:
View Solution
