The diagonal BD of a parallelogram ABCD bisects angles B and D. Prove that ABCD is a rhombus.
To prove that the parallelogram ABCD is a rhombus given that the diagonal BD bisects angles B and D, we can follow these steps:
1. Understand the Given Information:
We know that BD is the diagonal of parallelogram ABCD and it bisects angles B and D. This means:
∠ABD=∠CBD(1)
∠ADB=∠CDB(2)
2. Use the Properties of Parallelograms:
In a parallelogram, opposite angles are equal. Therefore, we have:
∠ABC=∠ADC(3)
3. Relate the Angles:
From equations (1) and (3), we can express:
∠ABD=∠CBD=12∠ABC(4)
Similarly, from equation (2) and (3):
∠ADB=∠CDB=12∠ADC(5)
4. Set Up Equalities:
Since ∠ABC=∠ADC from (3), we can write:
∠ABD=∠ADB(6)
and
∠CBD=∠CDB(7)
5. Conclude Side Lengths:
From (6) and (7), we see that:
△ABD≅△CDB(by Angle-Angle criterion)
This implies that:
AB=CDandAD=BC(8)
6. Use Parallelogram Properties:
Since ABCD is a parallelogram, we also have:
AB=CDandAD=BC(9)
7. Show All Sides are Equal:
From (8) and (9), we can conclude:
AB=AD=BC=CD(10)
8. Conclude ABCD is a Rhombus:
Since all sides of parallelogram ABCD are equal, it satisfies the definition of a rhombus. Therefore, we can conclude:
ABCD is a rhombus.
Final Statement:
Thus, we have proved that if the diagonal BD of a parallelogram ABCD bisects angles B and D, then ABCD is a rhombus.
---
We know that BD is the diagonal of parallelogram ABCD and it bisects angles B and D. This means:
∠ABD=∠CBD(1)
∠ADB=∠CDB(2)
In a parallelogram, opposite angles are equal. Therefore, we have:
∠ABC=∠ADC(3)
From equations (1) and (3), we can express:
∠ABD=∠CBD=12∠ABC(4)
Similarly, from equation (2) and (3):
∠ADB=∠CDB=12∠ADC(5)
Since ∠ABC=∠ADC from (3), we can write:
∠ABD=∠ADB(6)
and
∠CBD=∠CDB(7)
From (6) and (7), we see that:
△ABD≅△CDB(by Angle-Angle criterion)
This implies that:
AB=CDandAD=BC(8)
Since ABCD is a parallelogram, we also have:
AB=CDandAD=BC(9)
From (8) and (9), we can conclude:
AB=AD=BC=CD(10)
Since all sides of parallelogram ABCD are equal, it satisfies the definition of a rhombus. Therefore, we can conclude:
ABCD is a rhombus.
Thus, we have proved that if the diagonal BD of a parallelogram ABCD bisects angles B and D, then ABCD is a rhombus.
Topper's Solved these Questions
- RECTILINEAR FIGURESBOOK - ICSECHAPTER - RECTILINEAR FIGURESEXERCISE - QUADRILATERALS AND ITS PROPERTIES - 4 MARKS QUESTIONS
7 Videos
- RECTILINEAR FIGURESBOOK - ICSECHAPTER - RECTILINEAR FIGURESEXERCISE - POLYGONS - 4 MARKS QUESTIONS
4 Videos
- RATIONAL AND IRRATIONAL NUMBERS BOOK - ICSECHAPTER - RATIONAL AND IRRATIONAL NUMBERS EXERCISE - EXERCISE 1 (D)
21 Videos
- SIMULTANEOUS EQUATIONSBOOK - ICSECHAPTER - SIMULTANEOUS EQUATIONSEXERCISE - EXERCISE 6 (G)
13 Videos
Similar Questions
If the diagonal BD of a quadrillateral ABCD bisects both ∠Band∠D. Prove that ABBC=ADCD.
View SolutionTwo points X and Y lie on the diagonal BD of a parallelogram ABCD such that DX = BY. Prove that □AXCY is a parallelogram.
View SolutionPoints M and N are taken on the diagonal AC of a parallelogram ABCD such that AM= CN. Prove that BMDN is a parallelogram.
View SolutionIn Figure, diagonal AC of a quadrilateral ABCD bisects the angles A and C . Prove that AB=AD and CB=CD.
View SolutionIn the figure, diagonal AC of a quadrilateral ABCD bisects the angles A and C . Prove that AB=AD and CB=CD
View SolutionProve that the diagonals of a parallelogram bisect each other.
View SolutionIf the diagonals of a parallelogram ABCD are along the lines x+5y=7 and 10x−2y=9, then ABCD must be a
View SolutionABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
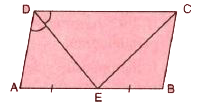
BC= BE
View Solutionif diagonals of a parallelogram bisect each other,prove that its a rhombus
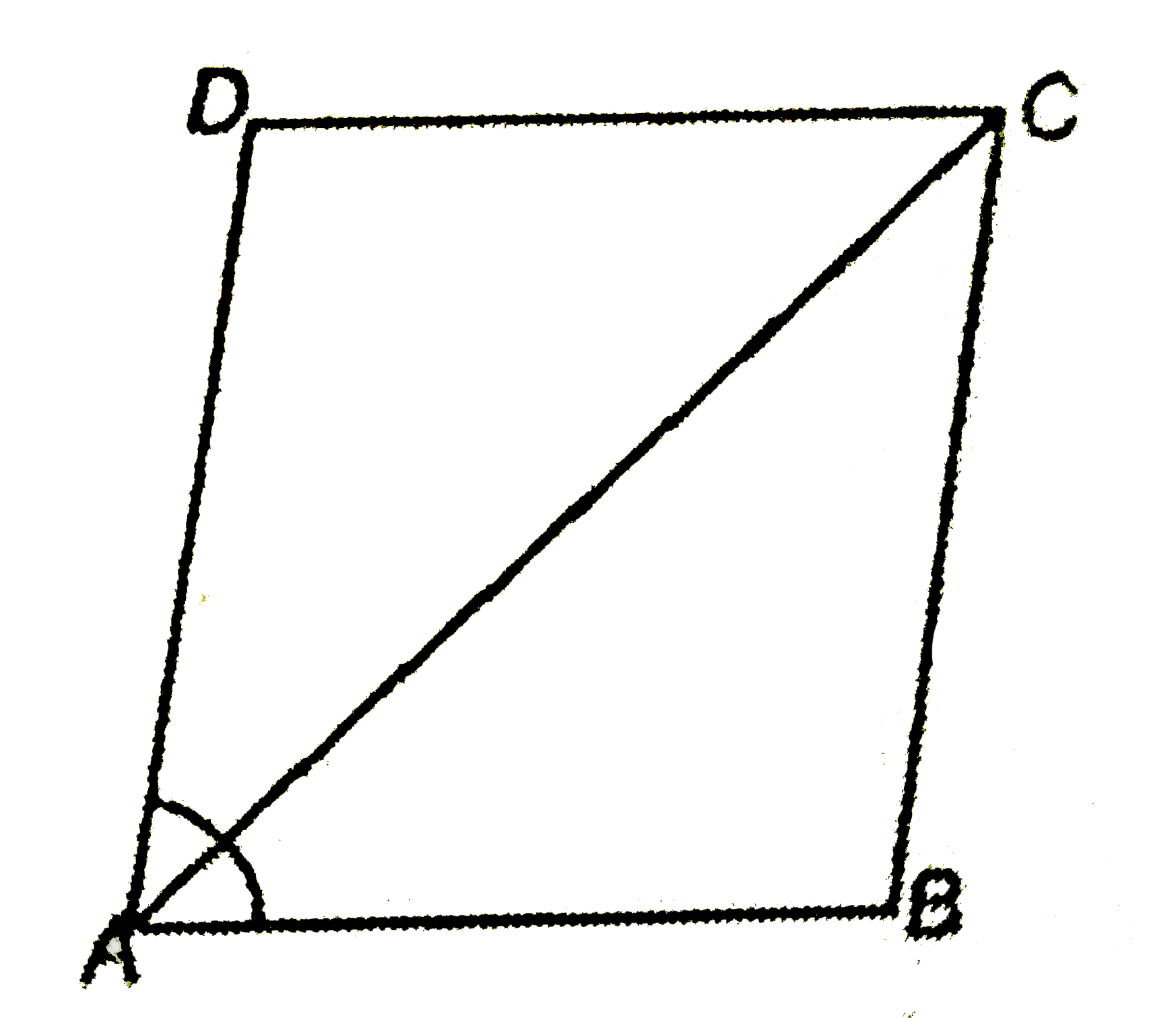
View SolutionDiagonal AC of a paraleligram ABCD bisects ∠A (sec figure). Show that:
(i) it bisects ∠C also (ii) ABCD is a rhombus.
View Solution
