The diagonals of a quadrilateral ABCD intersect each other at point'O' such that AOBO=CODO. Prove that ABCD is a trapezium.Solution in Telugu
Step by step video & image solution for The diagonals of a quadrilateral ABCD intersect each other at point'O' such that (AO)/(BO)=(CO)/(DO). Prove that ABCD is a trapezium. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMILAR TRIANGLES BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - SIMILAR TRIANGLES EXERCISE - TRY THIS
21 Videos
- SIMILAR TRIANGLES BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - SIMILAR TRIANGLES EXERCISE - EXERCISE 8.1
9 Videos
- SETS BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - SETS EXERCISE - CREATIVE BITS OF CCE MODEL EXAMINATION
116 Videos
- STATISTICSBOOK - VGS PUBLICATION-BRILLIANTCHAPTER - STATISTICSEXERCISE - EXERCISE
146 Videos
Similar Questions
E and F are points on the sides PQ and PR respectively of ΔPQR. For each of the following state whether EF∣∣QR or not?
PE=3.9 cm ,EQ=3cm,
PF=3.6 cm and FR=2.4 cm.
View SolutionIn ΔPQR,ST is a line such that PSSQ=PTTR and also ∠PST=∠PRQ. Prove that ΔPQR is an isosceles Triangle.
View SolutionLet A={1,2,{1},{1,2},3,4}, then which of the following is true?
View SolutionThe mean value can be calculated from both ungrouped and grouped date . Which one you think is more accurate ? Why ?
View SolutionThe diagonals of a quadrilateral ABCD intersect each other at the point O such that AOBO=CODO. Show that ABCD is a trapezium.
View SolutionABCD is a trapezium in which AB||DC and its diagonals intersect each other at the point O. Show that AOBO=CODO.
View Solutionएक चतर्भुज ABCD के विकर्ण परस्पर बिन्दु O पर इस प्रकार प्रतिच्छेद करते हैं कि AOBO=CODO है दर्शाइए कि ABCD एक समलम्ब है ।
View Solutionचतुर्भुज ABCD के विकर्ण एक -दूसरे को बिन्दु O पर इस प्रकार प्रतिच्छेद करते है कि AOBO=CODO तो सिद्ध कीजिए कि ABCD एक समलम्ब चतुर्भुज है ।
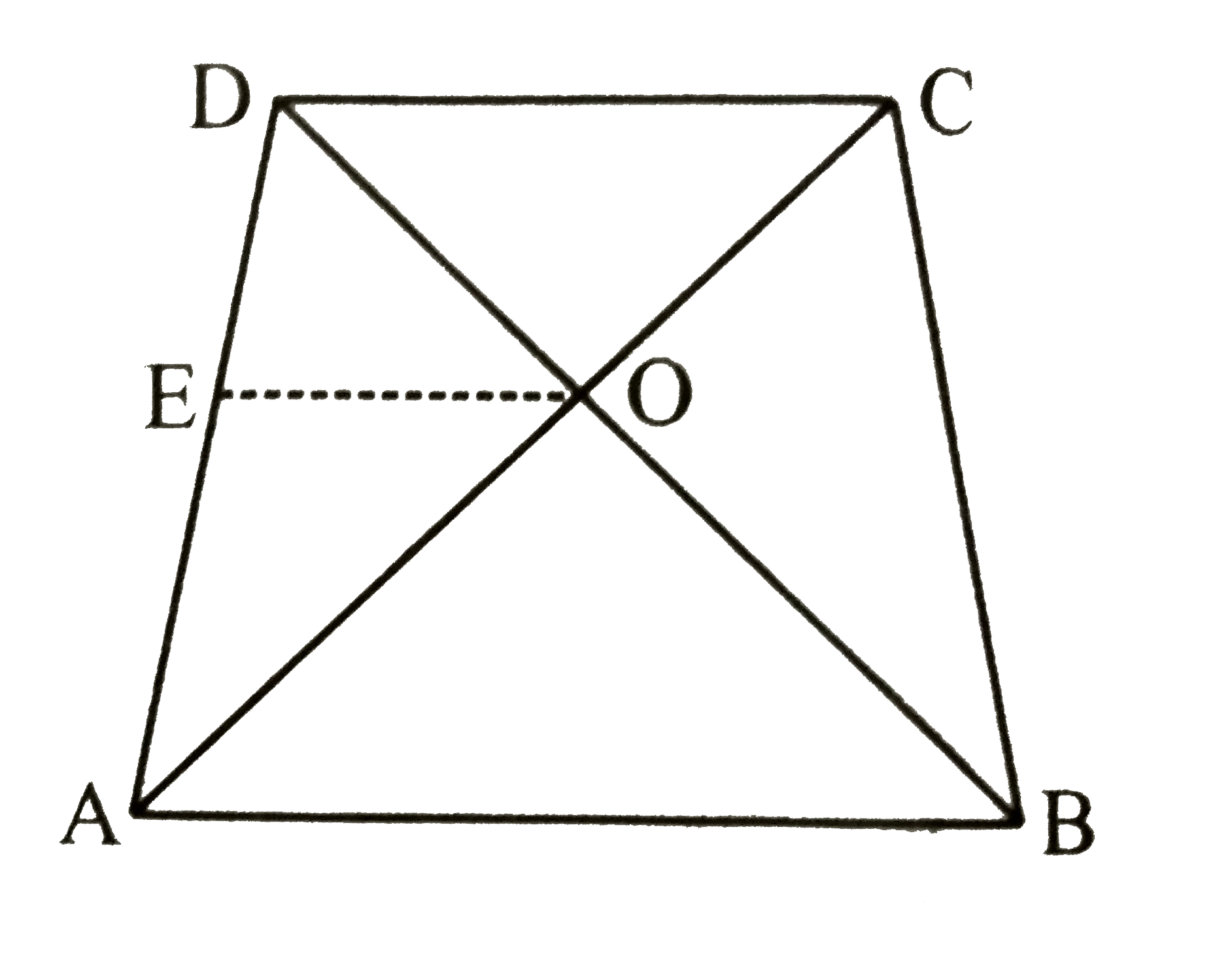
View SolutionThe diagonals of the quadrilateral ABCD intersect each other at O in such a way that AOBO=CODO. Prove that ABCD is a trapezium.
View SolutionABCD is a trapezium in which AB∣∣DC and its diagonal intersect each other at point 'O'. Show that AOBO=CODO.
View SolutionThe diagonals of a quadrilateral ABCD intersect each other at the point O such that AOBO=CODO show that ABCD is a trapezium.
View SolutionABCD is a trapezium in which AB||DC and its diagonals intersect each other as the point O. Show that AOBO=CODO.
View SolutionThe diagonal of a quadrilateral ABCD intersect each other at the point D such that AOBO=CODO. Show that ABCD is a trapezium.
View Solutionఒక చతుర్భుజము ABCD లో కర్ణములు 'O' బిందువు వద్ద ఖండించుకొనుము మరియు AOBO=CODO అయిన అది ఒక ట్రెపీజియం అని చూపండి.
View SolutionThe diagonals of a quadrilateral ABCD intersect each other at point ‘O’ such that AOBO=CODO. Prove that ABCD is a trapezium
View Solution
