The diameter of the front and the rear wheels of a tractor are 63 cm and 1.54 m respectively. The rear wheel is rotating at 24611 revolutions per minute. Find :
the distance travelled by the tractor in 40 minutes.
The correct Answer is:4.752 km
To solve the problem, we will follow these steps:
Step 1: Convert the diameters to the same unit
The diameter of the front wheel is given as 63 cm, and the diameter of the rear wheel is given as 1.54 m. We need to convert the diameter of the rear wheel into centimeters.
1. Conversion:
1.54m=1.54×100cm=154cm
Step 2: Calculate the radius of both wheels
The radius is half of the diameter.
1. Front Wheel Radius:
Rfront=632=31.5cm
2. Rear Wheel Radius:
Rrear=1542=77cm
Step 3: Calculate the circumference of both wheels
The circumference C of a circle is given by the formula:
C=2πr
1. Circumference of Front Wheel:
Cfront=2×227×31.5=198cm
2. Circumference of Rear Wheel:
Crear=2×227×77=484cm
Step 4: Calculate the distance traveled by the rear wheel in 40 minutes
The rear wheel is rotating at 24611 revolutions per minute. First, we convert this mixed number into an improper fraction:
1. Convert to Improper Fraction:
24611=24×11+611=264+611=27011revolutions per minute
2. Total Revolutions in 40 Minutes:
Total revolutions=27011×40=1080011≈981.82revolutions
3. Distance Traveled by Rear Wheel:
Distance=Total revolutions×Crear=1080011×484cm
To convert this distance into meters:
Distance=10800×48411cm=4752m
Final Answer
The distance traveled by the tractor in 40 minutes is 4752 meters.
---
The diameter of the front wheel is given as 63 cm, and the diameter of the rear wheel is given as 1.54 m. We need to convert the diameter of the rear wheel into centimeters.
1.54m=1.54×100cm=154cm
The radius is half of the diameter.
Rfront=632=31.5cm
2. Rear Wheel Radius:
Rrear=1542=77cm
The circumference C of a circle is given by the formula:
C=2πr
Cfront=2×227×31.5=198cm
Crear=2×227×77=484cm
The rear wheel is rotating at 24611 revolutions per minute. First, we convert this mixed number into an improper fraction:
24611=24×11+611=264+611=27011revolutions per minute
Total revolutions=27011×40=1080011≈981.82revolutions
Distance=Total revolutions×Crear=1080011×484cm
Distance=10800×48411cm=4752m
The distance traveled by the tractor in 40 minutes is 4752 meters.
Topper's Solved these Questions
Similar Questions
The diameter of the front and the rear wheels of a tractor are 63 cm and 1.54 m respectively. The rear wheel is rotating at 24611 revolutions per minute. Find :
the revolutions per minute made by the front wheel.
View SolutionThe diameters of front and rear wheels of a tractor are 80 cm and 2 m, respectively. Find the number of revolutions that rear wheel will make in covering a distance in which the front wheel makes 1400 revolutions .
View Solution
Knowledge Check
A rod 6 inches long is pivoted at a one end. If the free end of the 165 revolutions per minute, what is the total distance, in inches, traveled by the end of the rod in one seconds?
A14.5πB16.5πC29πD33π
The diameters of front and rear wheels of a tractor are 80 cm and 2m respectively. Find the number of revolutions that rear wheel will make in covering a distance in which the front wheel makes 1400 revolutions.
View SolutionA circular wheel if radius 28 cm makes 300 revolutions per minute. Find the speed of the wheel in kilometre per hour.
View SolutionIf the wheel of a bus with 50 cm radius makes 280 revolutions per minute, find the speed of the bus in km/h
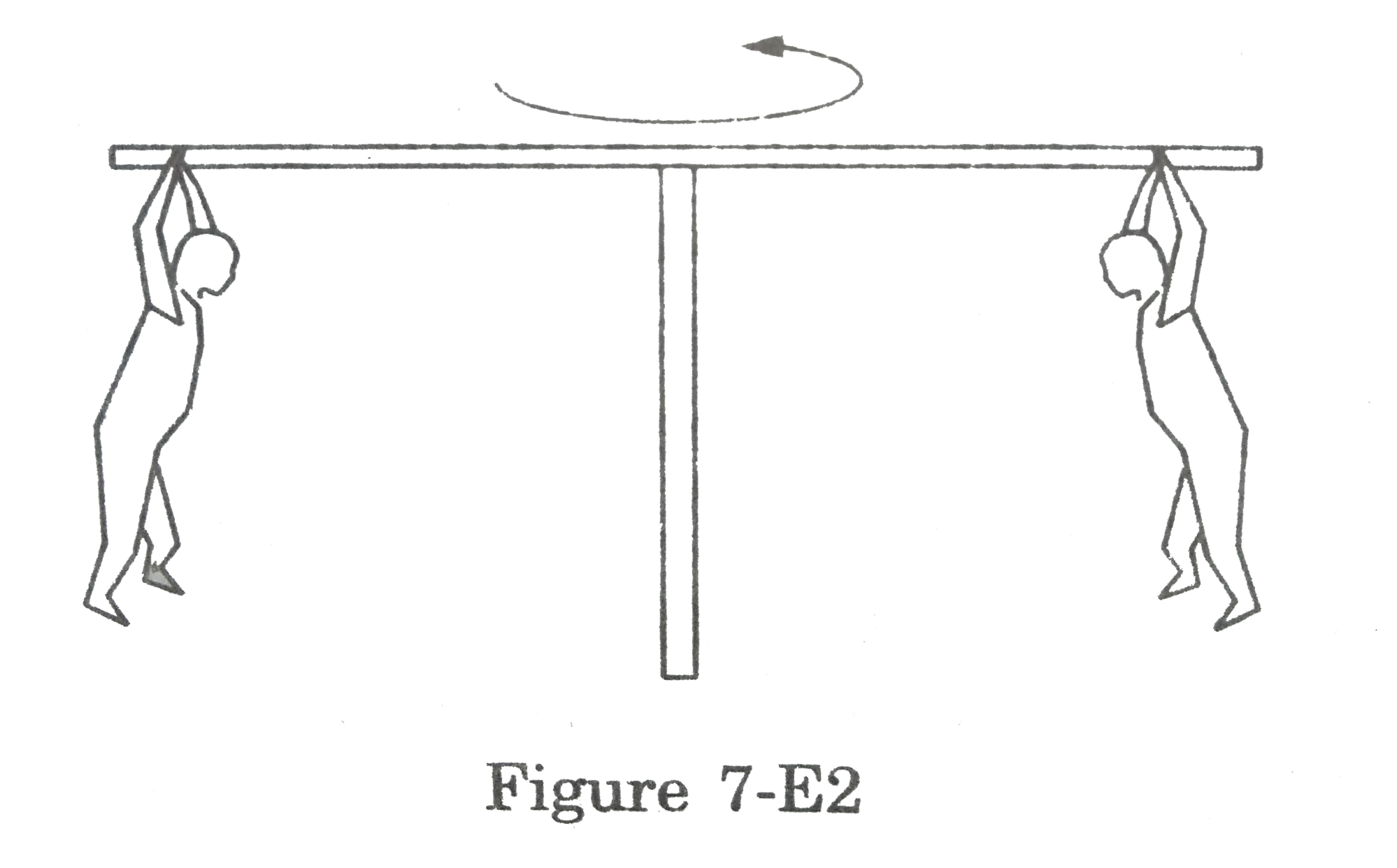
View SolutionIn a children's park a heavy rod is pivoted at the centre and is made to rotate about the pivot so that the rod always remains horizontal. Two kids hold the rod near the ends and thus rotate with the rod figure. Let the mas of each kid be 15 kg, the distance between the points of the rod where the two kids hold it be 3.0 m and suppose that the rod rotates at the rate of 20 revolutions per minute. Find the force of friction exerted by the rod on one of the kids.
View SolutionFind the magnitude of the centripetal acceleration of a particle on the tip of a blade, 0.30 metre in diameter, rotating at 1200 revolution per minute.
View SolutionThe diameter of a wheel of a car is 63 cm. Find the distance travelled by the car during the period, the wheel makes 1000 revolutions.
View SolutionThe wheel of a car is rotating at the rate of 1200 revolutions per minute. On pressing the accelerator for 10 seconds, it starts rotating at 4500 revolutions per minute. The angular acceleration of the wheel is
View Solution
