The equation of circle which touches X and Y-axes at the points (1,0) and (0,1) respectively is
A
x2+y2−4y+3=0
B
x2+y2−2y−2=0
C
x2+y2−2x−2y+2=0
D
x2+y2−2x−2y+1=0
Video Solution
Text Solution
The correct Answer is:D
Since , circle touches X- axis and Y-axis at points (1,0) and (0,1) respectively 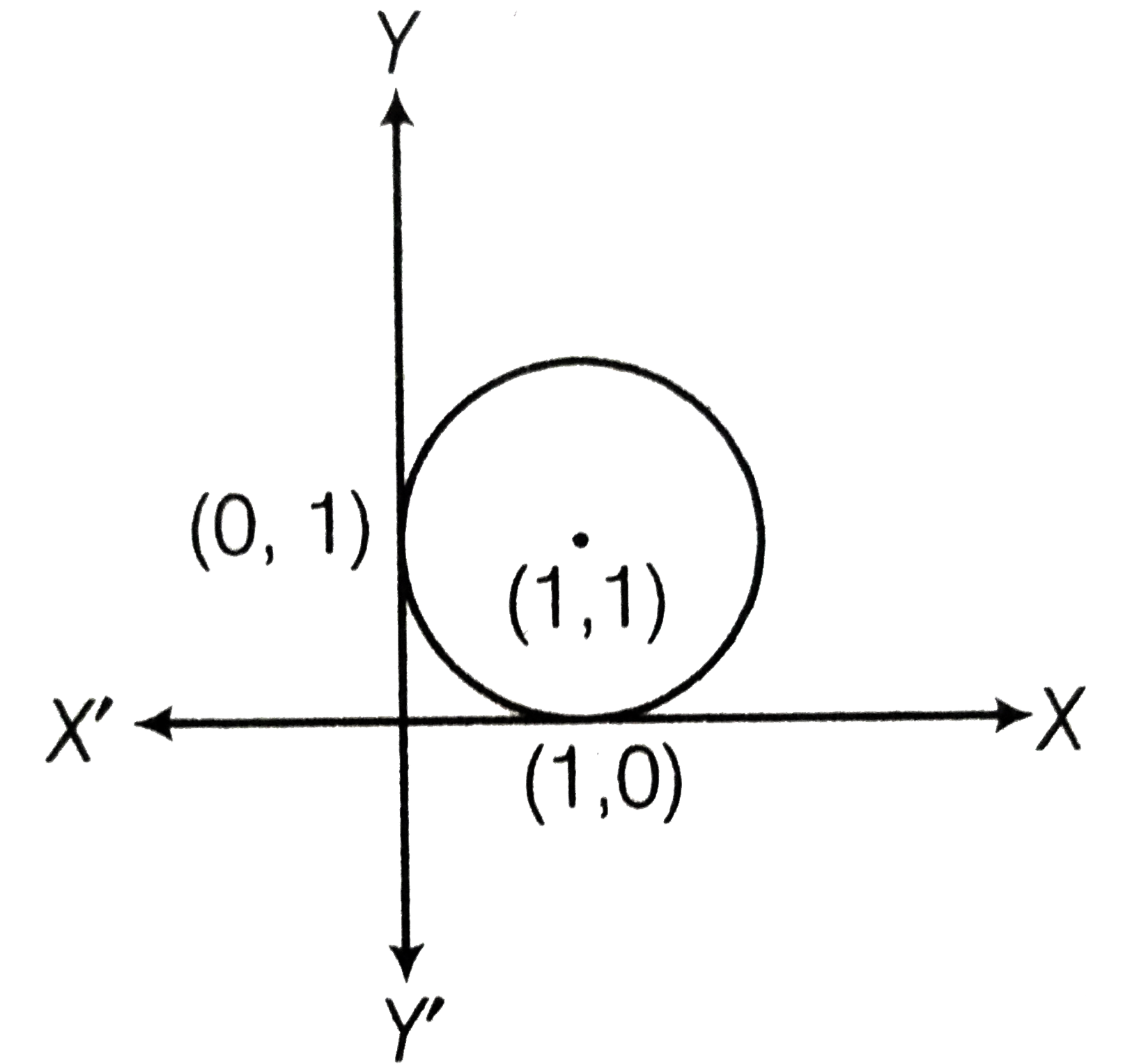 Hence equations of circle is
Hence equations of circle is
(x−12)+(y−1)2=12
⇒x2+y2−2x−2y+1=0
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The equation of the circle which touches both axes and whose centre is (x1,y1) is
Ax2+y2+2x1(x+y)+x21=0Bx2+y2−2x1(x+y)+x21=0Cx2+y2=x21+y21Dx2+y2+2xx1+2yy1=0What is the equation of circle which touches the lines x=0 , y = 0 and x=2 ?
Ax2+y2+2x+2r+1=0Bx2+y2−4x−4y+1=0Cx2+y2−2x−2y+1=0DNone of theseThe length of the radius of the circle which touches the x - axis at the point (1, 0) and passes through the point (2, 3) is
A103 unitsB35 unitsC65 unitsD53 units
Find the equation of the circle which touches the axis of: (1) x pass through the point (1,1) and have line x+y=3 as diameter
View SolutionEquation of the circle which touches the coordinate axes and passes through the point (2,1) is
View Solution(i) Find the equation of a circle which touches both the axes and whose centre is (2,2).
(ii) Find the equation of a circle, touching the lines x=0, y=0 and x=6.
View SolutionThe equation of circle which touches the line x+3y−2=0 and 3x+9y−2=0, also the point of contact an first line is(−1,1), is
View SolutionFind the equation of the circle which touches the lines x=0 ,y=0 and x=c
View Solution
