The equation of the locus of the middle point of a chord of the circle x2+y2=2(x+y) such that the pair of lines joining the origin to the point of intersection of the chord and the circle are equally inclined to the x-axis is x+y=2 (b) x−y=2 2x−y=1 (d) none of theseSolution in Tamil
x+y=2
x−y=2
2x−y=1
none of these
The correct Answer is:1
Solving y=mx and x2+y2−2x−2y=0, we get
x2+m2x2−2x−2mx=0
or x=0,2(1+m)1+m2
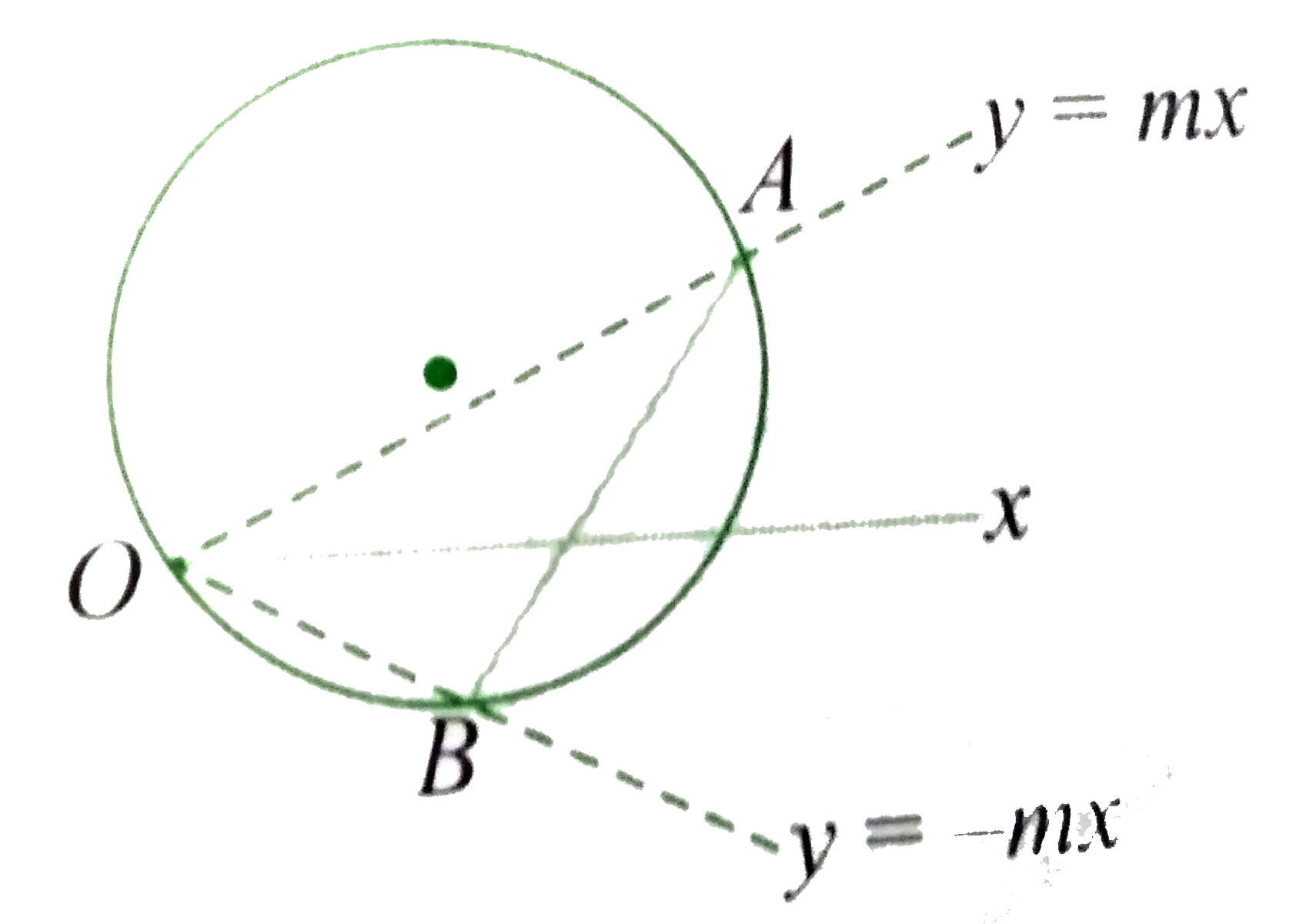
Similarly, solving y=−mx and the equation of the circle , we get
x=0,2(1−m)1+m2
:>A≡(2(1+m)1+m2,2m(1+m)1+m2)
and B≡(2(1−m)1+m2,−2m(1−m)1+m2)
Let the middle point of AB be (α,β). Then
α=12{2(1+m)1+m2,−2(1−m)1+m2}
and β=12{2m(1+m)1+m2,−2(1−m)1+m2}
∴α=21+m2,β=2m21+m2
Eliminating m from these, we get α+β=2.
Hence, the locus is x+y=2.
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Multiple)
25 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Comprehension)
46 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.6
5 Videos
- BINOMIAL THEOREMBOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
16 Videos
Similar Questions
about to only mathematics
View SolutionEach side of a square is of length 6 units and the centre of the square Is ( - 1, 2). One of its diagonals is parallel to x+y=0. Find the co-ordinates of the vertices of the square.
View SolutionIf the circle x2+y2+2x+3y+1=0 cuts x2+y2+4x+3y+2=0 at AandB , then find the equation of the circle on AB as diameter.
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
View SolutionThe equation of the locus of the middle point of a chord of the circle x2+y2=2(x+y) such that the pair of lines joining the origin to the point of intersection of the chord and the circle are equally inclined to the x-axis is x+y=2 (b) x−y=2 2x−y=1 (d) none of these
View SolutionFind the locus of the mid point of all chords of the circle x^(2)+y^(2)-2x-2y=0 such that the pairof.lines joining (0,0)& the point of intersection of the chords with the circles make equal an axis of x.
View SolutionIf the middle point of a chord of circle x^(2)+y^(2)+x-y-1=0be(1,1), then length of the chord is:
View SolutionThe locus of the middle point of the chord of the circle x^(2)+y^(2)=1 such that the segment of the chord on the parabola y=x^(2)-x subtends a right angle at the origin,is a circle whose centre and radius respectively are
View SolutionThe locus of points of intersection of the tangents to x2+y2=a2 at the extremeties of a chord of circle x2+y2=a2 which touches the circle x2+y2−2ax=0 is/are :
View SolutionFrom the origin chords are drawn to the circle x2+y2−2y=0. The locus of the middle points of these chords is
View SolutionThe locus of the middle points of the chords which are parallel to the chord joining (1,1) and (3,2) of the circle x2+y2−5x−y+4=0 is
View SolutionFrom the origin chords are drawn to the circle (x−1)2+y2=1. Show that the equation of the locus of the mid points of these chords is x2+y2=x=0
View SolutionThe locus of the centres of the circles passing thorugh the points of intersection of the circles x2+y2=1andx2+y2−2x+y=0 is
View SolutionThe equation of the locus of the middle point of a chord of the circle x2+y2=2(x+y) such that the pair of lines joining the origin to the point of intersection of the chord and the circle are equally inclined to the x-axis is x+y=2 (b) x−y=2 2x−y=1 (d) none of these
View SolutionFrom the origin, chords are drawn to the circle (x−1)2+y2=1. The equation of the locus of the mid-points of these chords
View Solution
