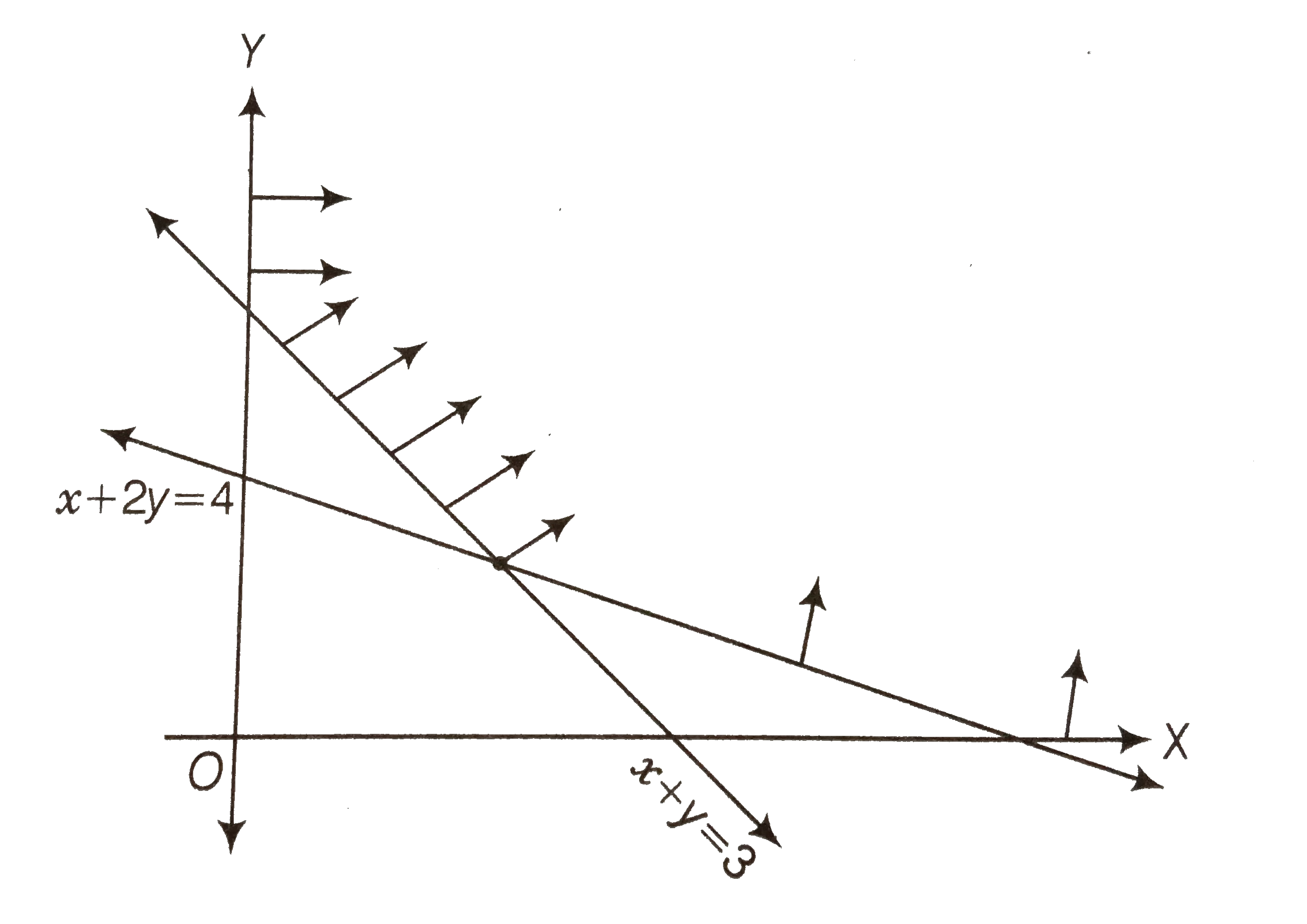
The feasible region for a LPP is shown in the following figure. Evaluate Z=4x+y at each of the corner points of the region. Find the minimum value of Z, if it exists
Video Solution
Text Solution
The correct Answer is:N/a
From the shaded region, it is clear that feasible region is unbouded with the corner points A (4,0), B(2,1), and C(0,3)
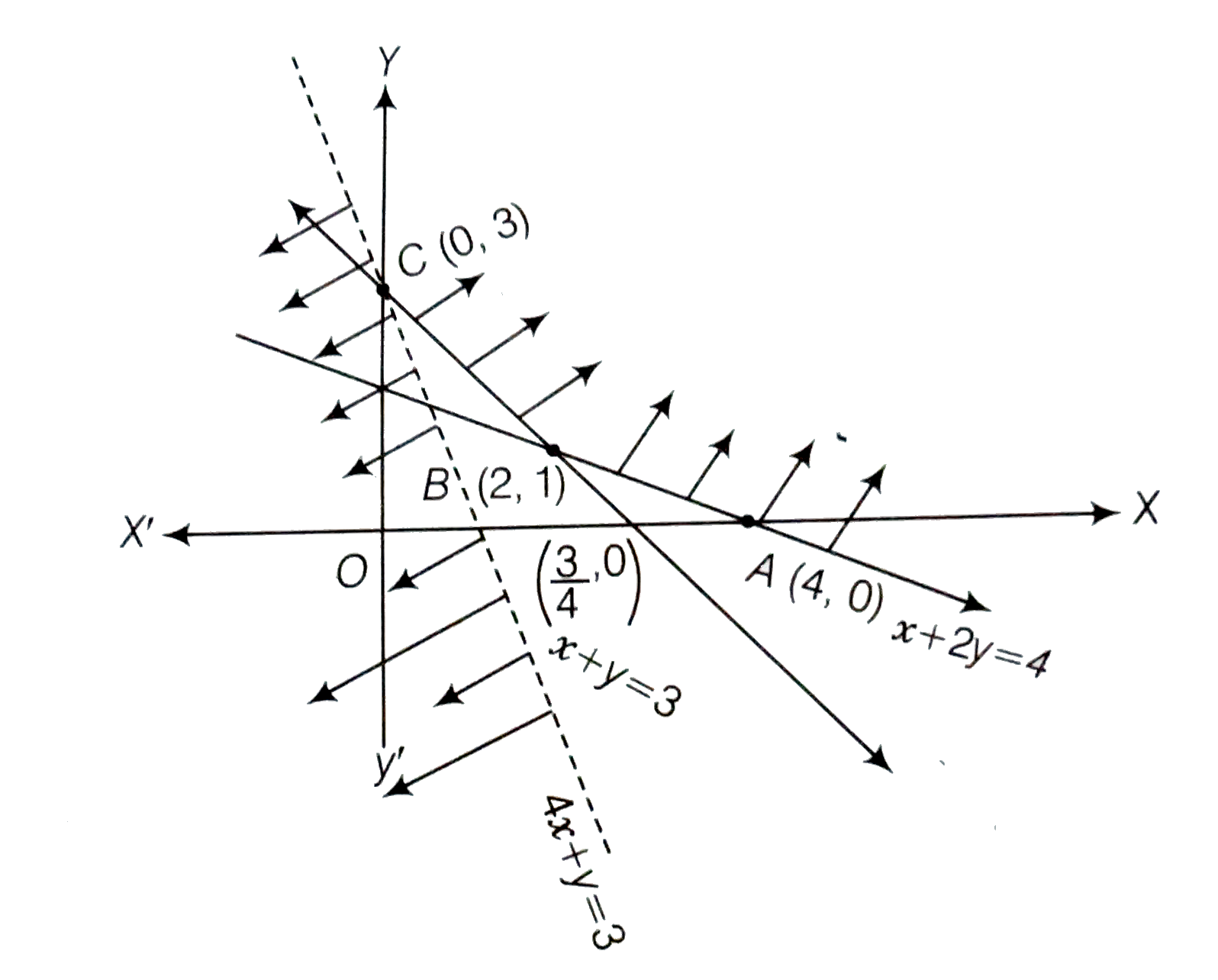
Now we see that 3 is the smallest value of Z at the corner point (0,3). Note that here we see that the region is unbounded, therefore 3 may or may not be the minimum value of Z. To decided this issue, we graph the inequality 4x+y≤3 and check whether ther resulting open half plan has not point in common with feasible reigon otherwise, Z has no minimum value. From the shown graph above,it is clear that there is no point in common with feasible region and hence Z has minimum value 3 at (0,3)
|
Topper's Solved these Questions
- LINEAR PROGRAMMINGBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - LINEAR PROGRAMMINGEXERCISE - OBJECTIVE
8 Videos
- LINEAR PROGRAMMINGBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - LINEAR PROGRAMMINGEXERCISE - FILLERS
7 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Fillers
18 Videos
- MATRICESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MATRICESEXERCISE - Solved example
101 Videos
Similar Questions
Feasible region (shaded) for a LPP is shown in following figure. Maximise Z=5x+7y.
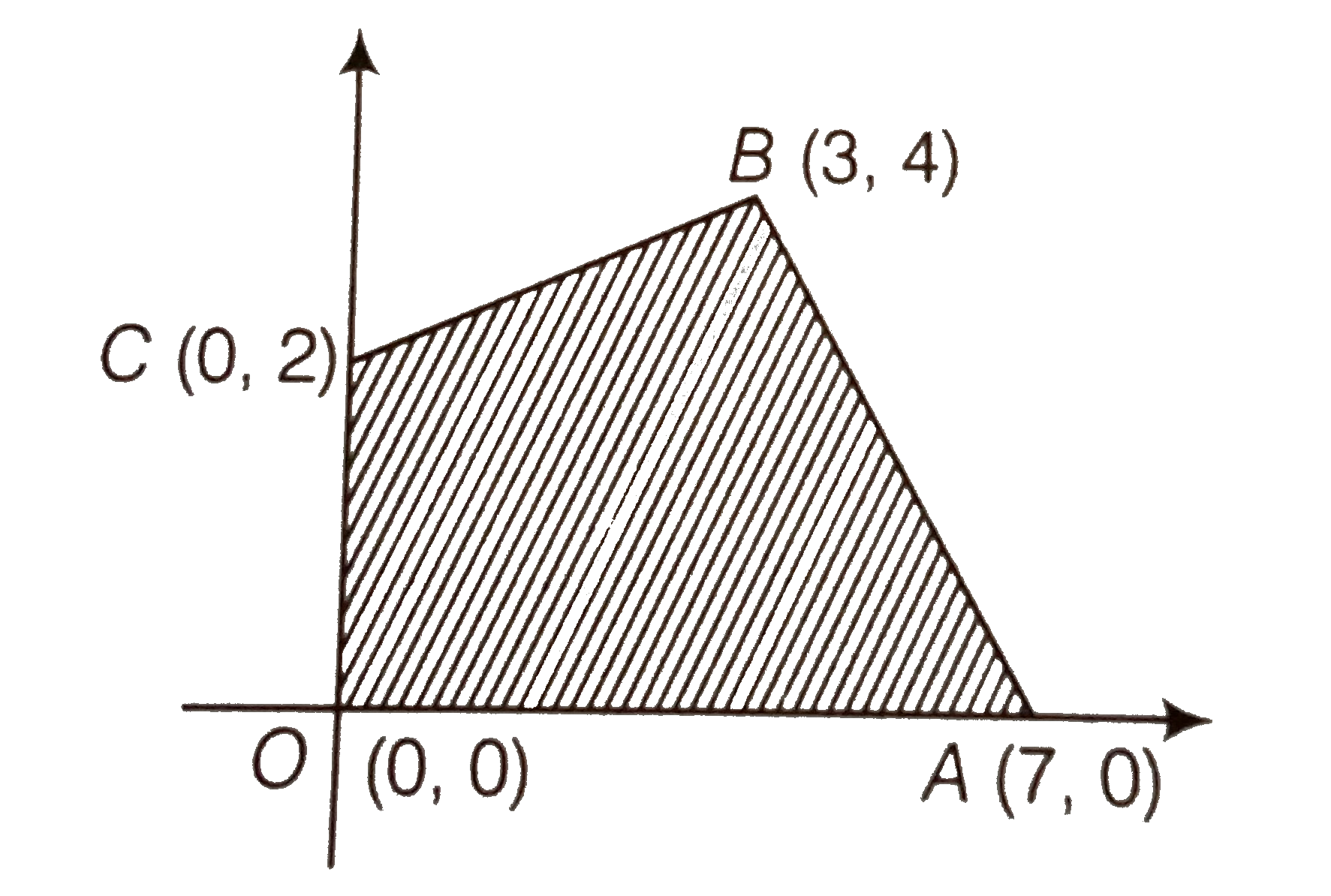
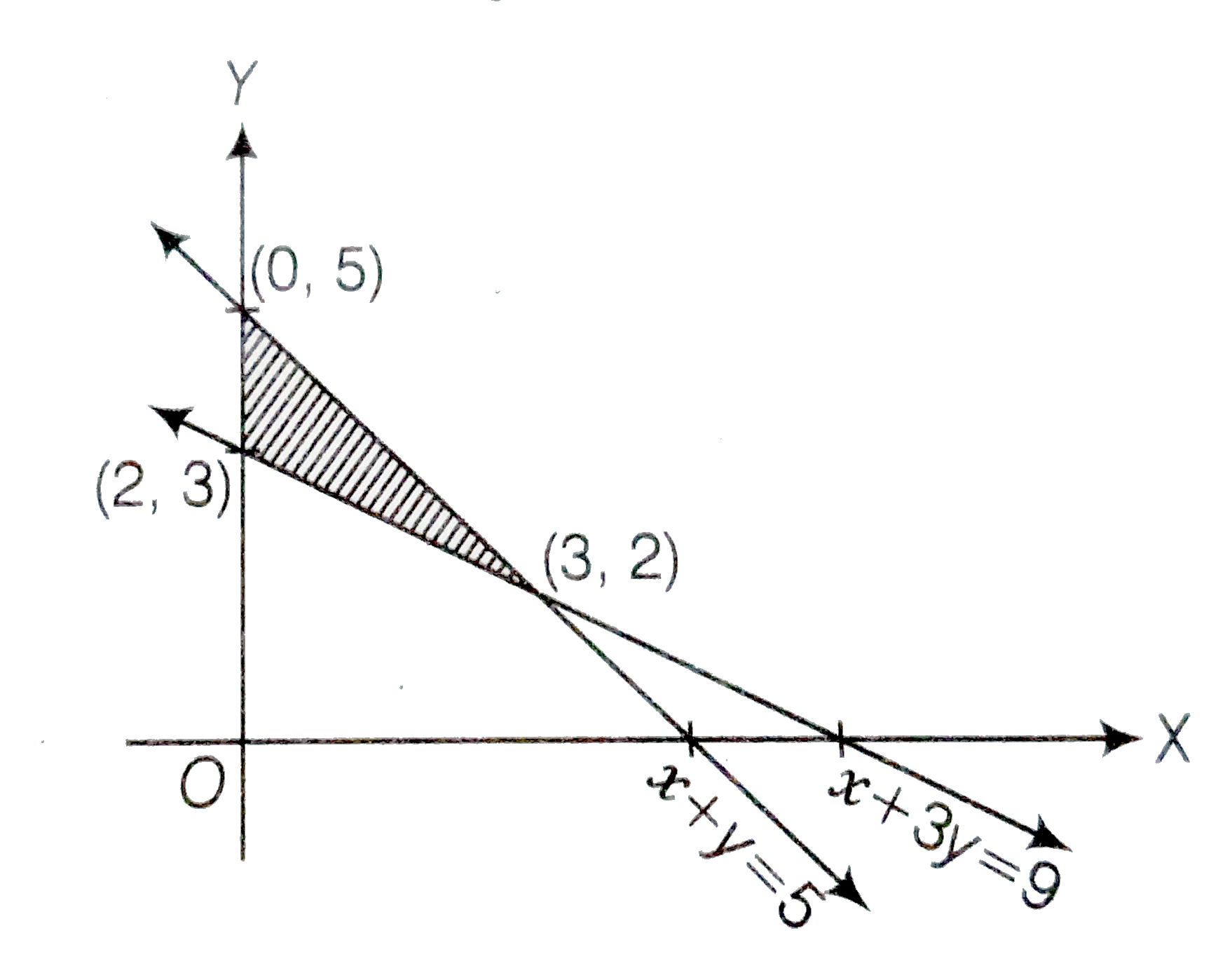
View SolutionThe feasible region for an LPP is shown in following figure. Find the minimum value of Z=11x+7y.
View SolutionThe feasible region for an LPP is shown in the following figure. Let F=3x−−4y be the objective function. Maximum value of F is
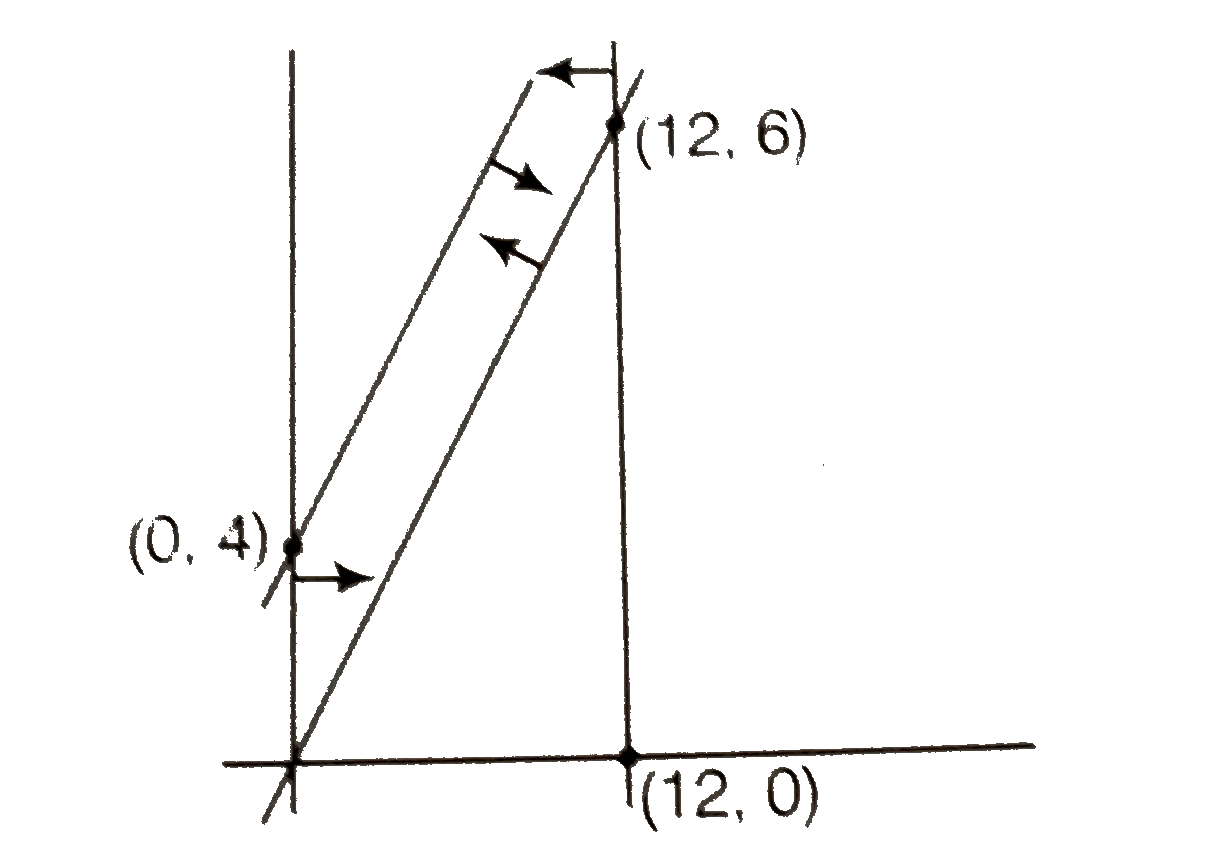
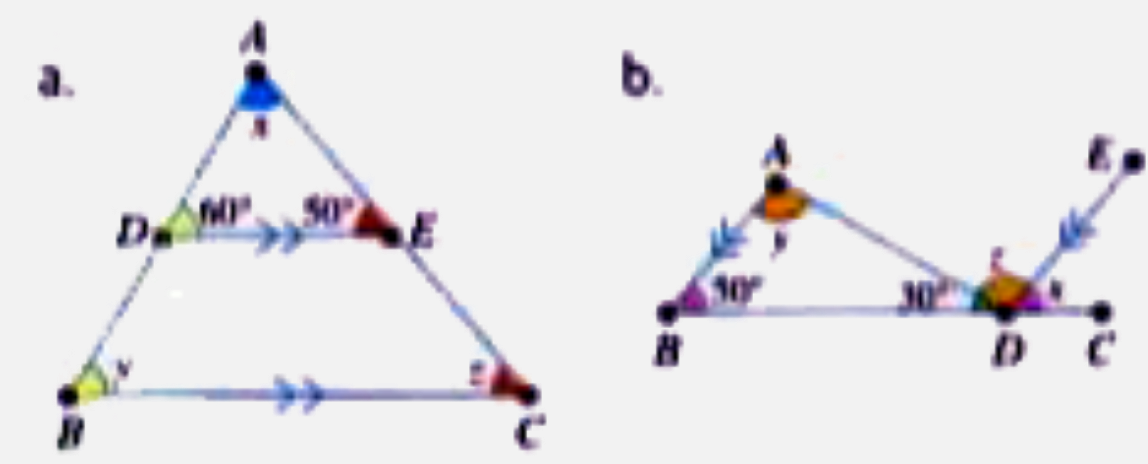
View SolutionFind x,y and z in each of the following figure:
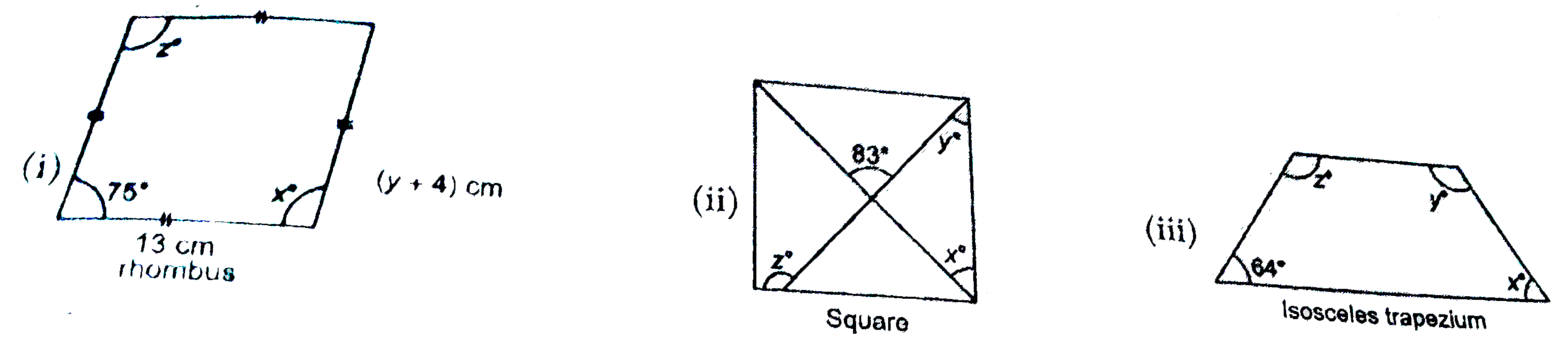
View SolutionFind the value of x,y and z.
View SolutionFind the value of x, y and z
View SolutionFind the value of x, y and z
View SolutionThe feasible solution for a LPP is shown in following figure. Let Z=3x-4y be the objective function, Minimum of Z occurs at
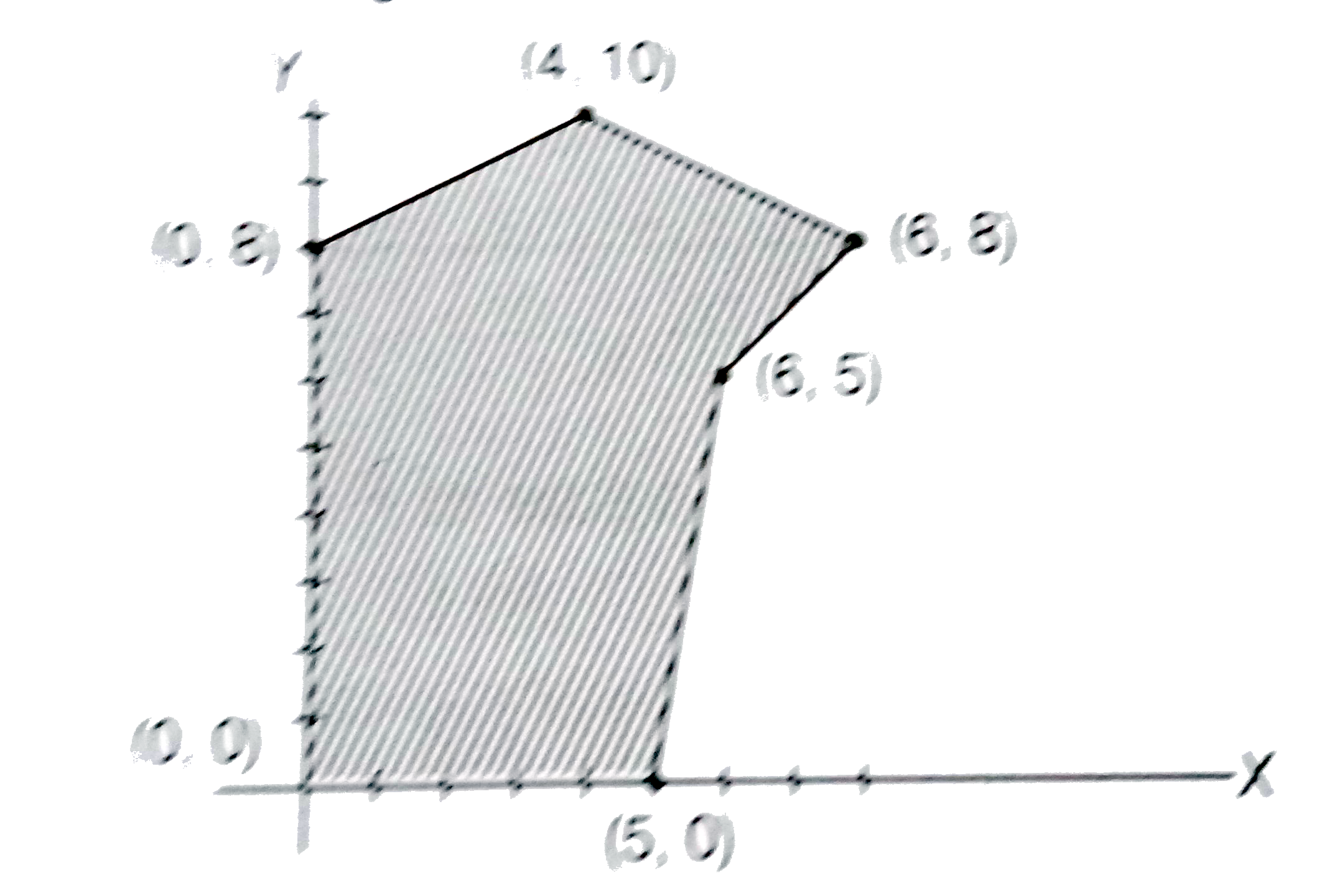
View SolutionFind the values of x,y, and z in the following figure.
View SolutionFind the minimum value of ∣z−1 if ||z−3|−|z+1∣∣=2.
View Solution
