The foci of the ellipse x225+y29=1 coincide with the hyperbola. It e = 2 for hyperbola, find the equation of the hyperbola. Solution in Bengali
The correct Answer is:3x2−y2=12
Step by step video, text & image solution for The foci of the ellipse (x^(2))/(25) + (y^(2))/(9) = 1 coincide with the hyperbola. It e = 2 for hyperbola, find the equation of the hyperbola. by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- HYPERBOLABOOK - CHHAYA PUBLICATIONCHAPTER - HYPERBOLAEXERCISE - SAMPLE QUESTIONS FOR COMPETITIVE EXAMS
12 Videos
- HYPERBOLABOOK - CHHAYA PUBLICATIONCHAPTER - HYPERBOLAEXERCISE - SHORT ANSWER TYPE QUESTIONS
37 Videos
- DIAGRAMMATIC REPRESENTATION OF DATABOOK - CHHAYA PUBLICATIONCHAPTER - DIAGRAMMATIC REPRESENTATION OF DATAEXERCISE - EXERCISE (Very short Answer Type Question)
7 Videos
- MATHEMATICAL INDUCTION BOOK - CHHAYA PUBLICATIONCHAPTER - MATHEMATICAL INDUCTION EXERCISE - Sample Questions for Competitive Exams
20 Videos
Similar Questions
The equation px2+2qxy+ry2+2sx+2ty+u=0 (where p≠q≠r and p,q,r,s,t and u are constants ) represents-
View SolutionFind (i) the length of axes (ii) length of latus rectum (iii) coordianates of vertices (iv) eccentricity (v) coordinates of foci and (vi) equations of the directries of each of the following hyperbolas:
(a) 4x2−9Y2=36 (b) 9y2−25x2=225
View SolutionThe following table gives the frequency distribution of heights in inches of a group of 100 peple:
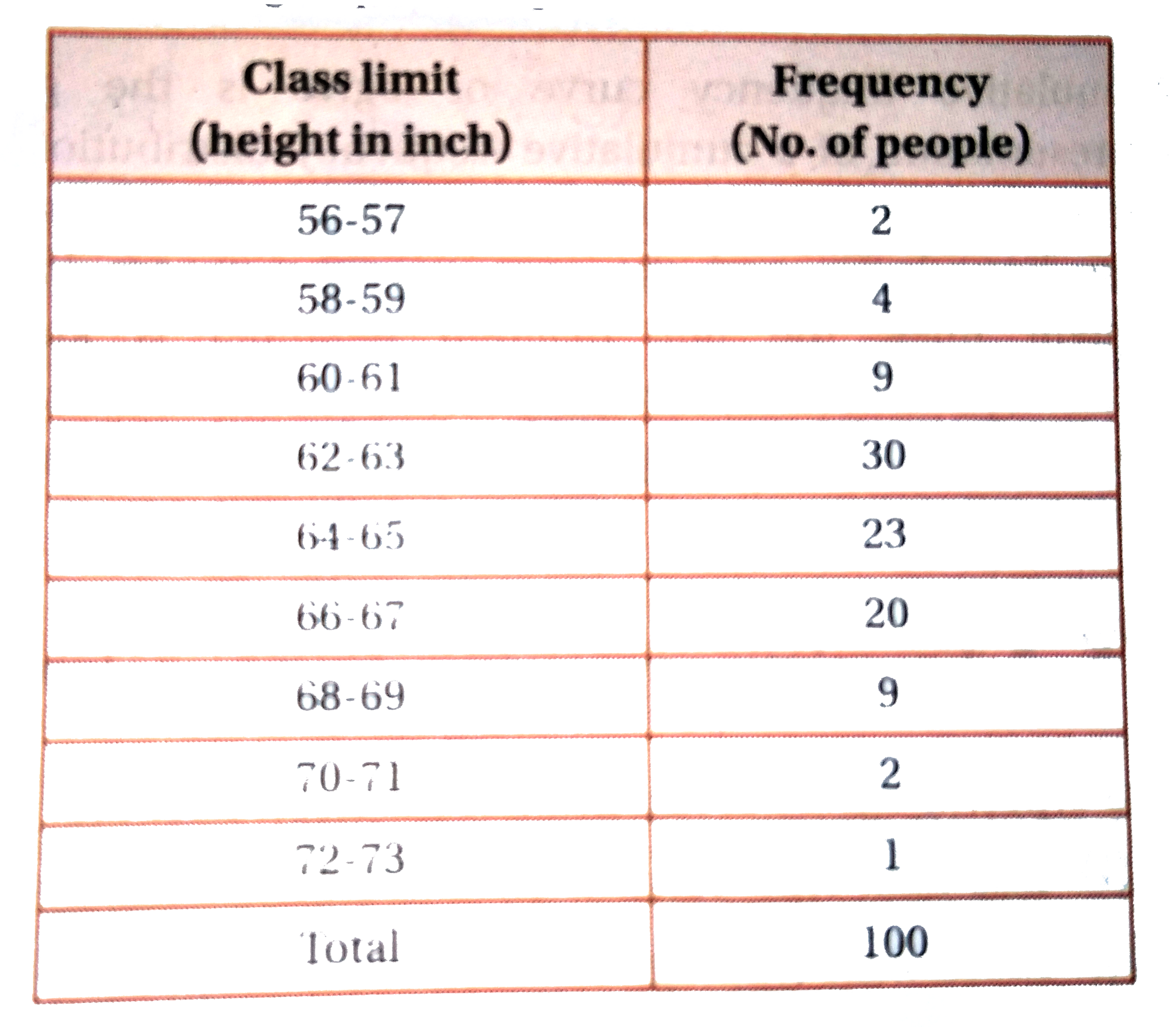 Draw a histogram for height distribution. Shade the portion of the histogram which will represent the total number of people whose heights lie between 60.5 and 66.5 inches.
Draw a histogram for height distribution. Shade the portion of the histogram which will represent the total number of people whose heights lie between 60.5 and 66.5 inches.
View SolutionIf for all n∈N and n≥1, then (32n−1) is always divisible by
View SolutionThe focii (x^(2))/(25)+(y^(2))/(9)=1 coincide with the hyperbola.It e=2 for hyperbola; find the equation of hyperbola.
View SolutionThe foci of a hyperbola coincide with the foci of the ellipse x225+y29=1. Find the equation of the hyperbola, if its eccentricity is 2.
View SolutionThe foci of a hyperbola coincide with the foci of the ellipse x225+y29=1. If the eccentricity of the hyperbola is 2, then the equation of the tangent of this hyperbola passing through the point (4,6) is
View Solutionएक अतिपरवलय की नाभियाँ दीर्घवृत x225+y216=1 की नाभियो से सम्पाती है । यदि अतिपरवलय की उत्केंद्रता ' sqrt (3) ' हो , तो उसका समीकरण ज्ञात कीजिए ।
View SolutionThe foci a hyperbola coincides with the foci of the ellispe x225+y29=1 . Find the equation of the hyperbola if its eccentricity is 2.
View SolutionIf a hyperbola passes through the foci of the ellipse x225+y216=1 . Its transverse and conjugate axes coincide respectively with the major and minor axes of the ellipse and if the product of eccentricities of hyperbola and ellipse is 1 then the equation of a. hyperbola is x29−y216=1 b. the equation of hyperbola is x29−y225=1 c. focus of hyperbola is (5, 0) d. focus of hyperbola is (5√3,0)
View SolutionIf a hyperbola passes through the foci of the ellipse x225+y216=1 . Its transverse and conjugate axes coincide respectively with the major and minor axes of the ellipse and if the product of eccentricities of hyperbola and ellipse is 1 then the equation of a. hyperbola is x29−y216=1 b. the equation of hyperbola is x29−y225=1 c. focus of hyperbola is (5, 0) d. focus of hyperbola is (5√3,0)
View SolutionThe foci of a hyperbola coincide with the foci of the ellipse x225+y29=1. Find the equation of the hyperbola, if its eccentricity is 2.
View SolutionIf the equation of ellipse x225+y216=1 then find the equation of hyperbola such that product of eccentircities of both curves equal to 1 and the axes of ellipse are coinciding with hyperbola and also hyperbola passes through the focii of ellipse.
View SolutionThe foci of a hyperola coincide with the foci of the ellipse x225+y29=1. Find the equation of the hyperola, if its eccentricity is 2.
View Solutionएक अतिपरवलय की नाभियां दीर्घवृत्त x225+y29=1 की नाभियों के संपाती हो तो अतिपरवलय का समीकरण जिसकी उत्केन्द्रता 2 है, होगा
View Solution
