The function $f (x) = {(9 - x^{2})}^{2}$ increases in

A

$(- 3, 0) \cup (3, \infty)$

B

$(- \infty, - 3) \cup (3, \infty)$

C

$(- \infty, - 3) \cup (0, 3)$

D

$(- 3, 3)$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Given $f (x) = {(9 - x^{2})}^{2}$
$\Rightarrow f' (x) = 2 (9 - x^{2}) (- 2 x)$
Now put $f' (x) = 0$
$\Rightarrow 2 (9 - x^{2}) (- 2 x) = 0$
$\Rightarrow x = 0, \pm 3$

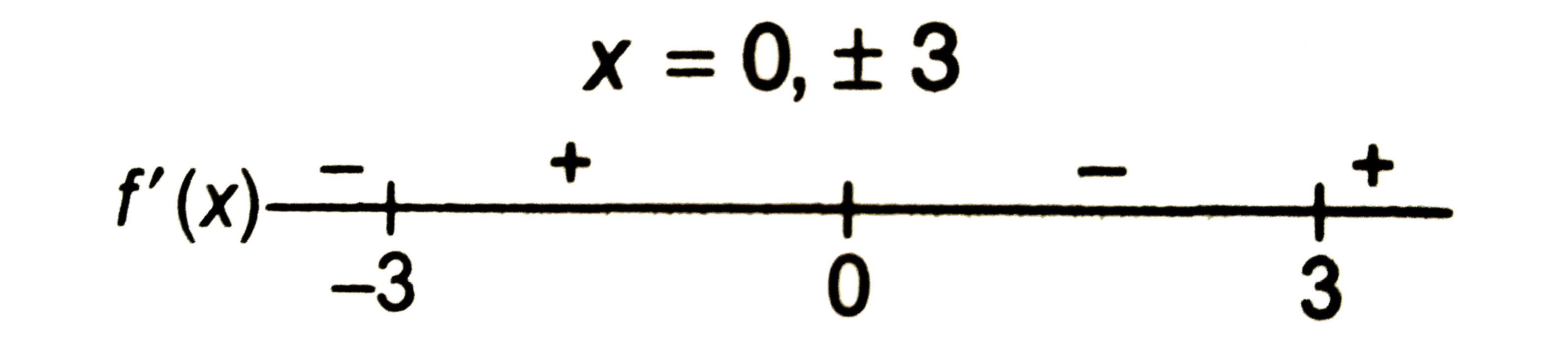
$∵ f' (x)$ is increasing in $(- 3, 0) \cup (3, \infty)$

|

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF DERIVATIVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The interval in which the function $f (x) = x^{e^{2 - x}}$ increases is
A $(- \infty, 0)$
B $(2, \infty)$
C $(0, 2)$
Dnone of these
Question 2 - Select One
What is the interval in which the function f(x) = $\sqrt{9 - x^{2}}$ is increasing ? $(f (x) > 0)$
A $0 < x < 3$
B $- 3 < x < 0$
C $0 < x < 9$
D $- 3 < x < 3$
Question 3 - Select One
The function f(x) = $x^{2} - 2 x$ increase forall
A $x > - 1$ only
B $x < = 1$ only
C $x > 1$ only
D $x < 1$ only

MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS-APPLICATIONS OF DERIVATIVES-MHT CET CORNER

Home
Ask Doubt
Profile