The line 6x+8y=48 intersects the coordinates axes at A and B, respecively. A line L bisects the area and the perimeter of triangle OAB, where O is the origin.
The number of such lines possible is
a. 1
b. 2
c. 3
d. 4Solution in Bengali
does not intersect AB
does not intersect OB
does not intersect OA
can intersect all the sides
The correct Answer is:C
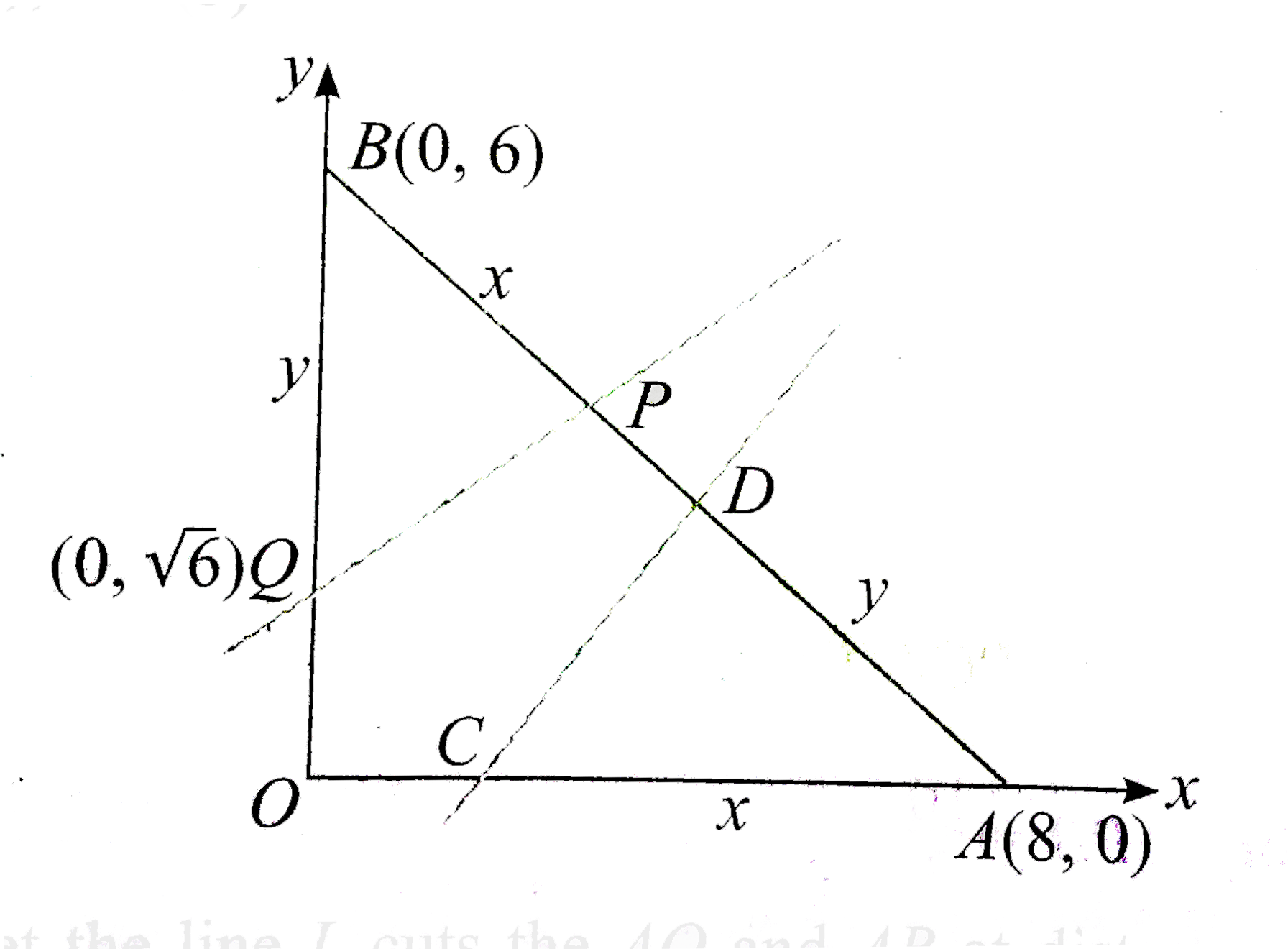
Case I : Let the line L cuts the AO and AB at distances x and y, respectively, from A. Then, the area of the triangle with sides x and y is
or xy=40
Also, x+y=12 (from perimeter bisection). Then x and y are the roots of which has imaginary roots.
Case II: if the line L cuts OB and BA at distances y and x, respectively, from B, then we have xy=30 and x+y=12.
Solving, we get
Case III: If the line L cuts the sides OA and OB at distances x and y, respectively, from O, then
x+y=12 and xy=24
(not possible)
So there is a unique line possible. Let point P be .
Using the parametric equation of AB, we have
Hence, the slope of PQ is
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MATRIX MATCH TYPE)8videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (NUMERICAL VALUE TYPE)13videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MULTIPLE CORRECT ANSWERS TYPE)30videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )1videos
THEORY OF EQUATIONS
Similar Questions
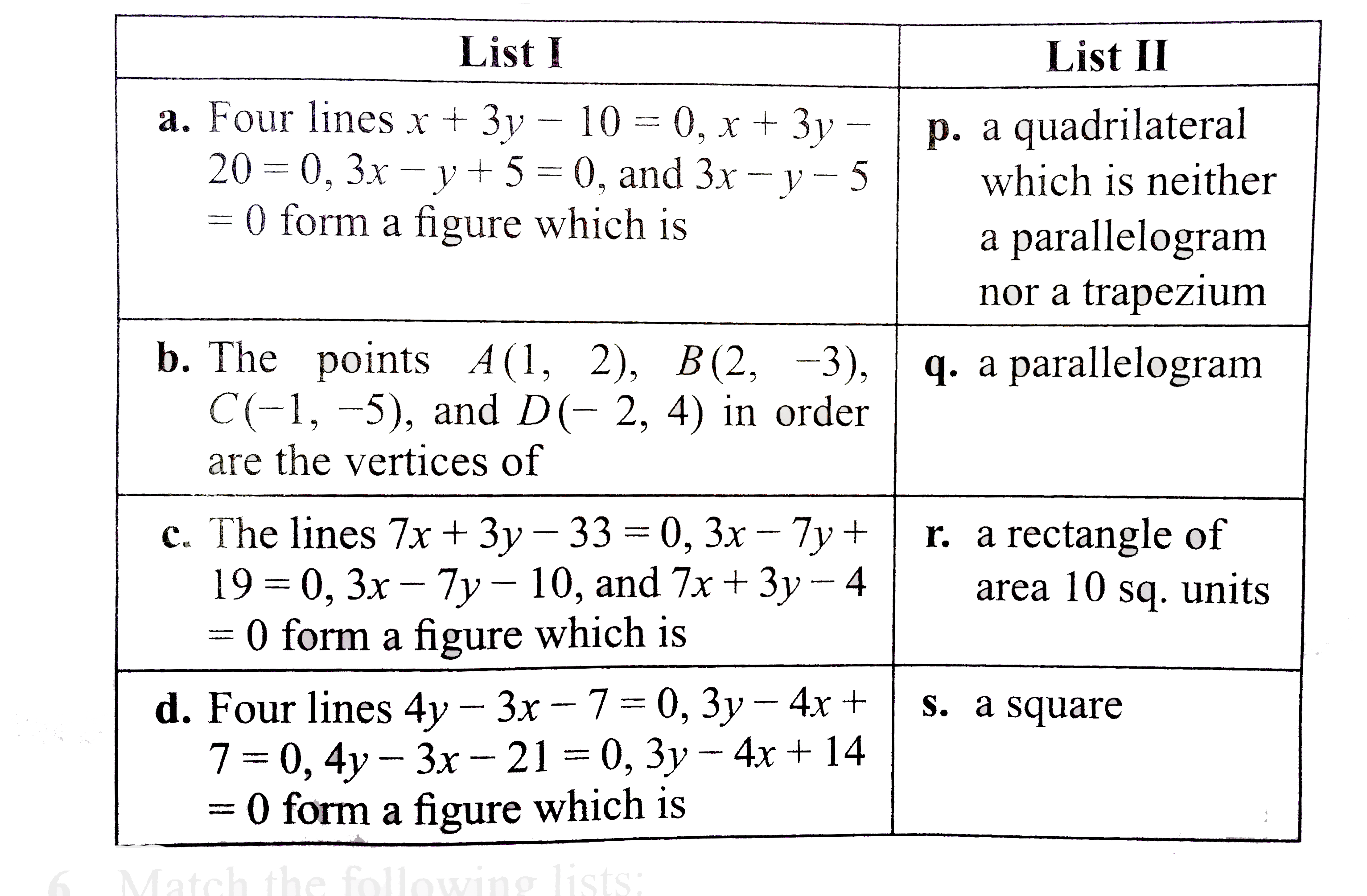
Match the following lists:
 View Solution
View SolutionFind the number of permutations of the set {1,2,3,4} in which no two adjacent positions are filled by consecutive integers ( increasing orders).
View SolutionIf P is a point (x,y) on the line y=−3x such that P and the point (3, 4) are on the opposite sides of the line 3x−4y=8, then x>815 (b) x>85 y<−85 (d) y<−815
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
08:36View SolutionThe line 6x+8y=48 intersects the coordinates axes at A and B, respecively. A line L bisects the area and the perimeter of triangle OAB, where O is the origin.
The number of such lines possible isView SolutionThe line 6x+8y=48 intersects the coordinates axes at A and B, respecively. A line L bisects the area and the perimeter of triangle OAB, where O is the origin.
The slope of line L can be03:57View SolutionThe line 6x+8y=48 intersects the coordinates axes at A and B, respecively. A line L bisects the area and the perimeter of triangle OAB, where O is the origin.
Line LView SolutionTangents at P to rectangular hyperbola xy=2 meets coordinate axes at A and B, then area of triangle OAB (where O is origin) is :
03:14View SolutionThe line 6x + 8y - 48 intersects the co - ordinate axis at A and B respectively. A line L bisects the area and the perimeter of the traingle OAB, where O is the origin , the number of such lines possible is
03:15View SolutionThe line 6x + 8y - 48 intersects the co - ordinate axis at A and B respectively. A line L bisects the area and the perimeter of the traingle OAB, where O is the origin .
The slope of the line L can be02:59View SolutionA straight line meets the coordinates axes at A and B, so that the centroid of the triangle OAB is (1, 2). Then the equation of the line AB is
02:40View SolutionTangents at P to rectangular hyperbola xy=2 meets coordinate axes at A and B, then area of triangle OAB (where O is origin) is :
02:40View SolutionThe line 6x+8y=48 intersects the coordinates axes at A and B, respecively. A line L bisects the area and the perimeter of triangle OAB, where O is the origin.
The number of such lines possible is a. 1 b. 2 c. 3 d. 402:40View SolutionThe line 6x+8y=48 intersects the coordinates axes at A and B, respecively. A line L bisects the area and the perimeter of triangle OAB, where O is the origin.
Line L05:29View SolutionIf the lines joining the origin to the points of intersection of a line L and x2+y2=4 are coordinate axes then equation of L is
05:56View Solution
