"The maximum or the minimum of the objective function occurs only at the corner points of the feasible region". This theorem is know as fundamental theorem of
Agebra
Arithmetic
Calculus
Extreme points
The correct Answer is:D
Topper's Solved these Questions
Similar Questions
Knowledge Check
The maximum or minimum of the objective funtion occurs only at the corner points of the feasible region. This theorem is known as fundamental theorem of
AAlgebraBArithmeticCCalculusDExtreme pointOne of the corner points of the feasible region of inequalities gives
AOptimal solution of LPPBObjective functionCConstraintsDLinear assumptionsWhich of the following statements is false?
AThe feasible region is always a concave regionBThe maximum (or minimum) solution of the objective function occurs at the vertex of the feasible regionCIf two corner points produce the same maximum (or minimum) value of the objective function, then every point on the line segment joining these points will also give the same maximum (or minimum) two. valuesDAll of the above
Maximum value of the objective function Z = ax +by in a LPP always occurs at only one corner point of the feasible region.
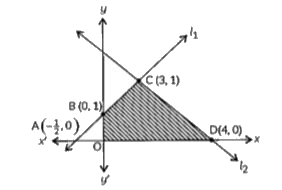
View SolutionThe corner points of the feasible region, shown as shaded in the graph below, are :
View SolutionIn a LPP, the maximum value of the objective function Z = ax +by is always 0, if origin is one of the corner point of the feasible region.
View SolutionIn a LPP, the maximum value of the objective function Z = ax +by is always 0, if origin is one of the corner point of the feasible region.
View SolutionConsider the following statements
I. If the feasible region of an LPP is undbounded then maximum or minimum value of the obJective function Z = ax + by may or may not exist .
II. Maximum value of the objective function Z = ax + by in an LPP always occurs at only one corner point of the feasible region.
Ill. In an LPP, the minimum value of the objective function Z = ax + by is always 0, if origin is one of the corner point of the feasible region.
IV. In an LPP, the maximum value of the objective function Z = ax + by is always finite.
Which of the following statements are true?
View Solution
