Maximum value of the objective function Z = ax +by in a LPP always occurs at only one corner point of the feasible region.
Video Solution
Text Solution
N/a
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
Consider the following statements
I. If the feasible region of an LPP is undbounded then maximum or minimum value of the obJective function Z = ax + by may or may not exist .
II. Maximum value of the objective function Z = ax + by in an LPP always occurs at only one corner point of the feasible region.
Ill. In an LPP, the minimum value of the objective function Z = ax + by is always 0, if origin is one of the corner point of the feasible region.
IV. In an LPP, the maximum value of the objective function Z = ax + by is always finite.
Which of the following statements are true?AI and IVBII and IIICI and IIIDII and IVThe maximum or minimum of the objective funtion occurs only at the corner points of the feasible region. This theorem is known as fundamental theorem of
AAlgebraBArithmeticCCalculusDExtreme pointThe optimal value of the objective function is attained at the point
Agiven by intersectionn of inequations with axis onlyBgiven by intersection of inequations with X-axis onlyCgiven by corner points of the feasible regionDnone of the above
In a LPP, the maximum value of the objective function Z = ax +by is always finite.
View SolutionIn a LPP, the objective function is always.
View SolutionIn a LPP, if the objective function Z=ax+by has the same maximum value of two corner points of the feasible region, then every point of the line segment joining these two points give the same ..value.
View SolutionThe maximum value of objective function Z=3x+y under given feasible region is
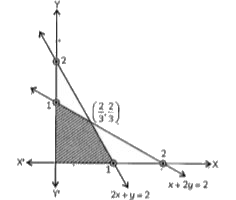
View SolutionThe, objective function is maximum or minimum at a point, which lies on the boundary of the feasible region.
View Solution
