The mean and standard deviation of six observations are 8 and 4, respectively. If each observation is multiplied by 3, find the new mean and new standard deviation of the resulting observations.
as we know that, ¯x=∑xin=8
if 3 is multiplied b each observation, then
¯x'=∑(3xi)n=3∑xin
=3⋅8=24
now, Variance is
σ2=∑(xi−¯x)2n
new variance will be
σ2'=∑(3xi−3¯x)2n
=9∑(xi−¯x)2n
=9⋅16=144
SD'=√σ2'=√144=12
answer
Topper's Solved these Questions
- STATISTICSBOOK - NCERT ENGLISHCHAPTER - STATISTICSEXERCISE - SOLVED EXAMPLES
18 Videos
- STATISTICSBOOK - NCERT ENGLISHCHAPTER - STATISTICSEXERCISE - EXERCISE 15.3
5 Videos
- SETSBOOK - NCERT ENGLISHCHAPTER - SETSEXERCISE - EXERCISE 1.5
7 Videos
- STRAIGHT LINESBOOK - NCERT ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE 10.4
4 Videos
Similar Questions
Find the mean and the standard deviation from the following:
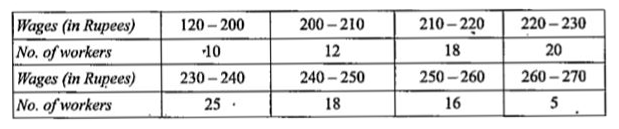
View SolutionThe mean of 15 observations is 32. Find the resulting mean, if each observation is multiplied by 2
View SolutionFind the mean and standard deviation from the following data :

View SolutionThe standard deviation of the observations 22, 26, 28, 20, 24, 30 is
View SolutionConsider the following statements :
(i) Mean of 100 observations is 50 and standard deviation is 10. If 5 is added to each observation the new mean and standard deviation are 55, 10.
(ii) Mean of 100 observations is 50 and standard deviation is 10. If each observation is multiplied by 3 then the new mean and standard deviation are 50, 10/3.
The true statements are :
View SolutionFind the mean and standard deviation of the following frequency distribution :
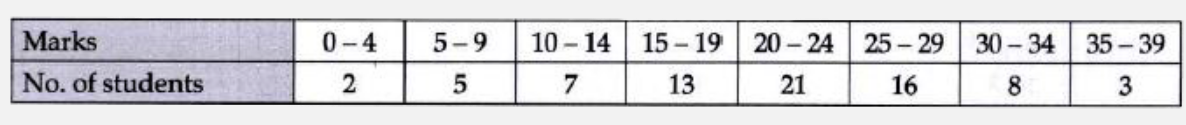
View SolutionMean and standard deviations of 10 observations are 20 and 2 respectively. If p (p≠0) is multiplied to each observation and then q (q≠0) is subtracted then new mean and standard deviation becomes half of original value . Then find q
View SolutionIf the men and standards deviation of 100 observations are 50 and 4 respectively. Find the sum of all the observations and the sum of their squares.
View SolutionLet s be the standard deviation of n observations. Each of the n observations is multiplied by a constant c. Then the standard deviation of the resulting number is
View SolutionThe mean of 15 observations is 32. Find the resulting mean, if each observation is increased by 3
View Solution
