The mean of a distribution is 23, the median is 24 and the mode is 25.5. It is most likely that this distribution is : Solution in Tamil
Positively skewed
Symmetrical
Asymptotic
Negatively skewed
Step by step video & image solution for The mean of a distribution is 23, the median is 24 and the mode is 25.5. It is most likely that this distribution is : by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Topper's Solved these Questions
- BOOK - SURA PUBLICATIONCHAPTER - STATISTICSEXERCISE - Additional Questions and Answers (Exercise 8.61videos
STATISTICS
- BOOK - SURA PUBLICATIONCHAPTER - STATISTICSEXERCISE - Additional Questions and Answers (Exercise 8.71videos
STATISTICS
- BOOK - SURA PUBLICATIONCHAPTER - STATISTICSEXERCISE - Additional Questions and Answers (Exercise 8.41videos
STATISTICS
- BOOK - SURA PUBLICATIONCHAPTER - SET LANGUAGEEXERCISE - UNIT TEST20videos
SET LANGUAGE
- BOOK - SURA PUBLICATIONCHAPTER - TRIGONOMETRYEXERCISE - UNIT TEST16videos
TRIGONOMETRY
Similar Questions
The middle value of an ordered array of numbers is
01:38View SolutionThe weights of students in a school is a :
View SolutionThe mean of first 10 natural numbers.
02:08View SolutionThe set P={x∣x∈Z,−1<x<1} is a
02:37View SolutionFrom the given figure, find all the trigonometric ratios of angle B.
 02:11View Solution
02:11View SolutionThe median of a distribution is 60 and mean deviation about median is 24, then the coefficient of mean deviation of the distribution is-
05:21View SolutionThe mean and the median of a distribution are 45.9 and 46 respectively. The mode will be
01:38View SolutionThe relation between mean, median, and mode for a frequency distribution is
01:56View SolutionIn a frequancy distribution,if the mean = 35 & median = 33, then find the mode of the frequency distribution.
01:16View SolutionIf mode of a distribution is 8 and its mean is 8 then median is .......
01:50View SolutionCalculate the mean, the median and the mode of the following distribution:
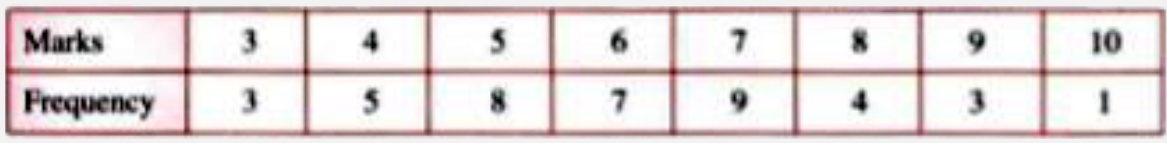 01:14View Solution
01:14View SolutionFor a frequency distribution, mean, median and mode are connected by the relation . . . . .
02:07View SolutionFor a frequency distribution, Mode-Median = . . . . . × (Median-Mean)
02:07View SolutionFor a given frequency distribution, median = 13.2 and mean = 15.3. Then by the relation between mean, median and mode, mode = . . . . . .
View SolutionThe median and mean of a distribution of 29 and 32 respectively then mode
01:33View Solution

